Continuity ⁠

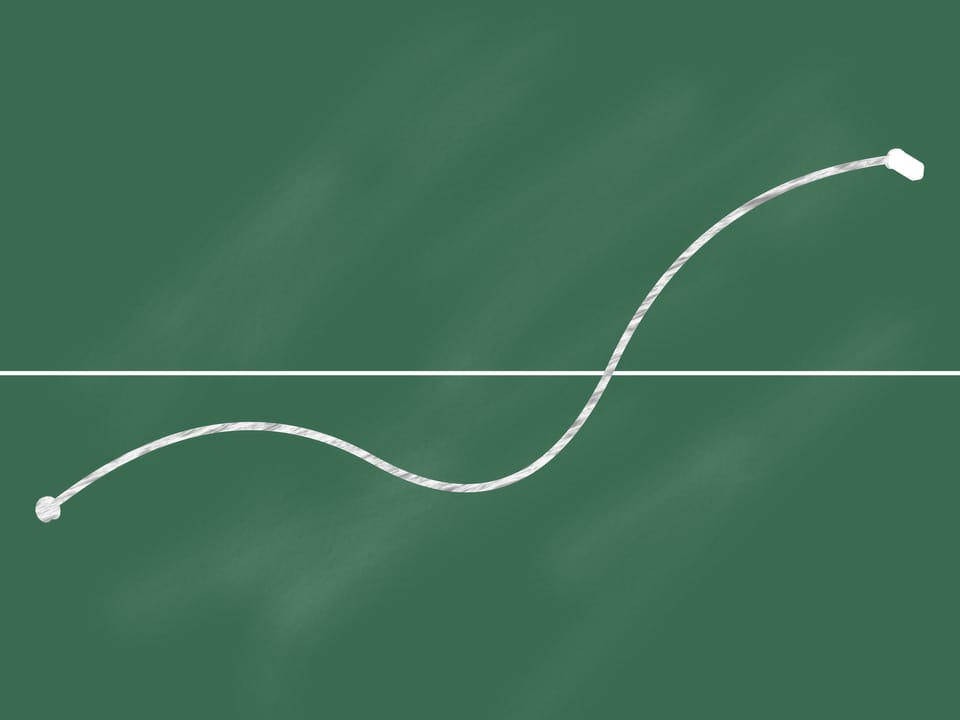
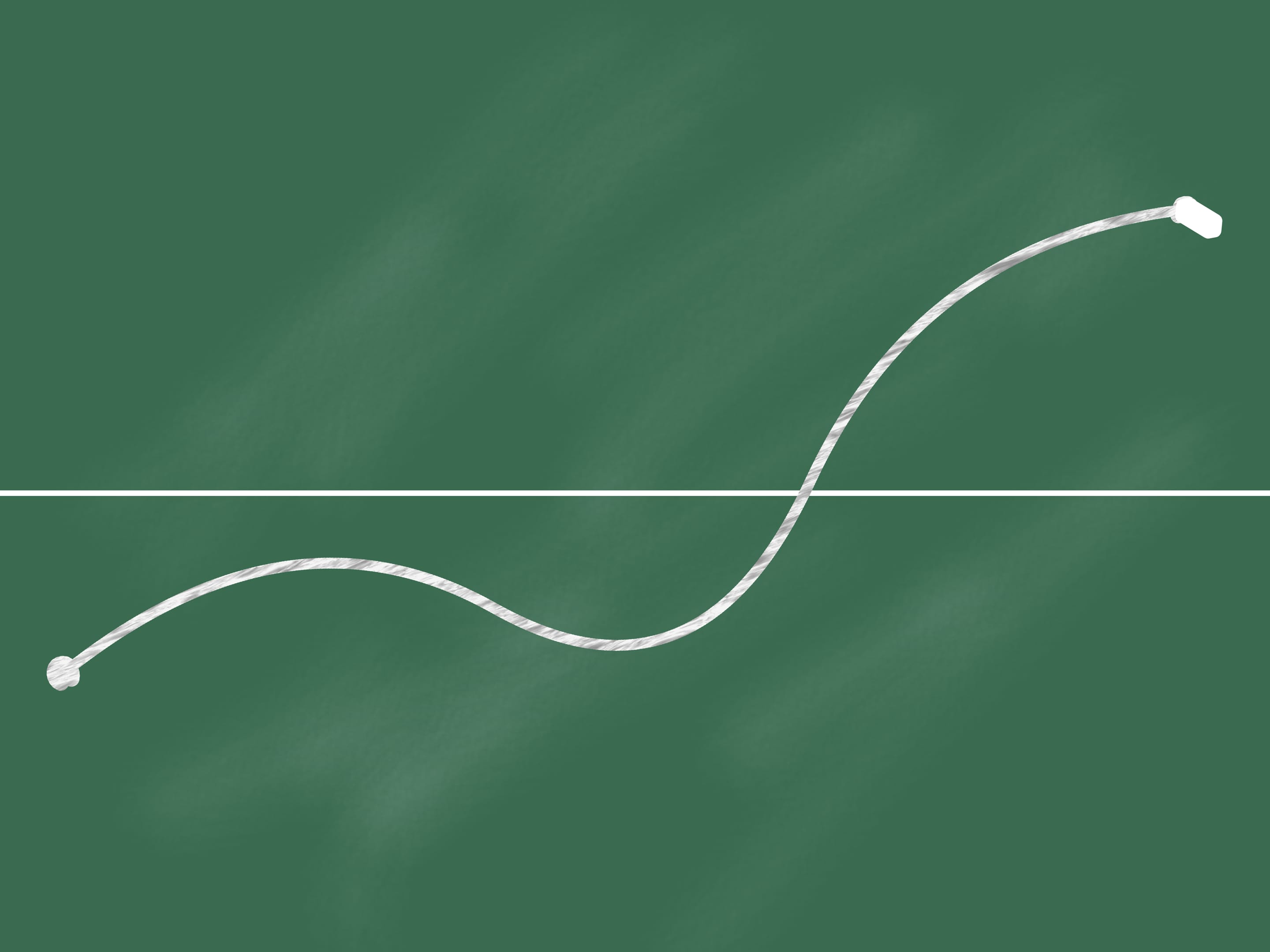
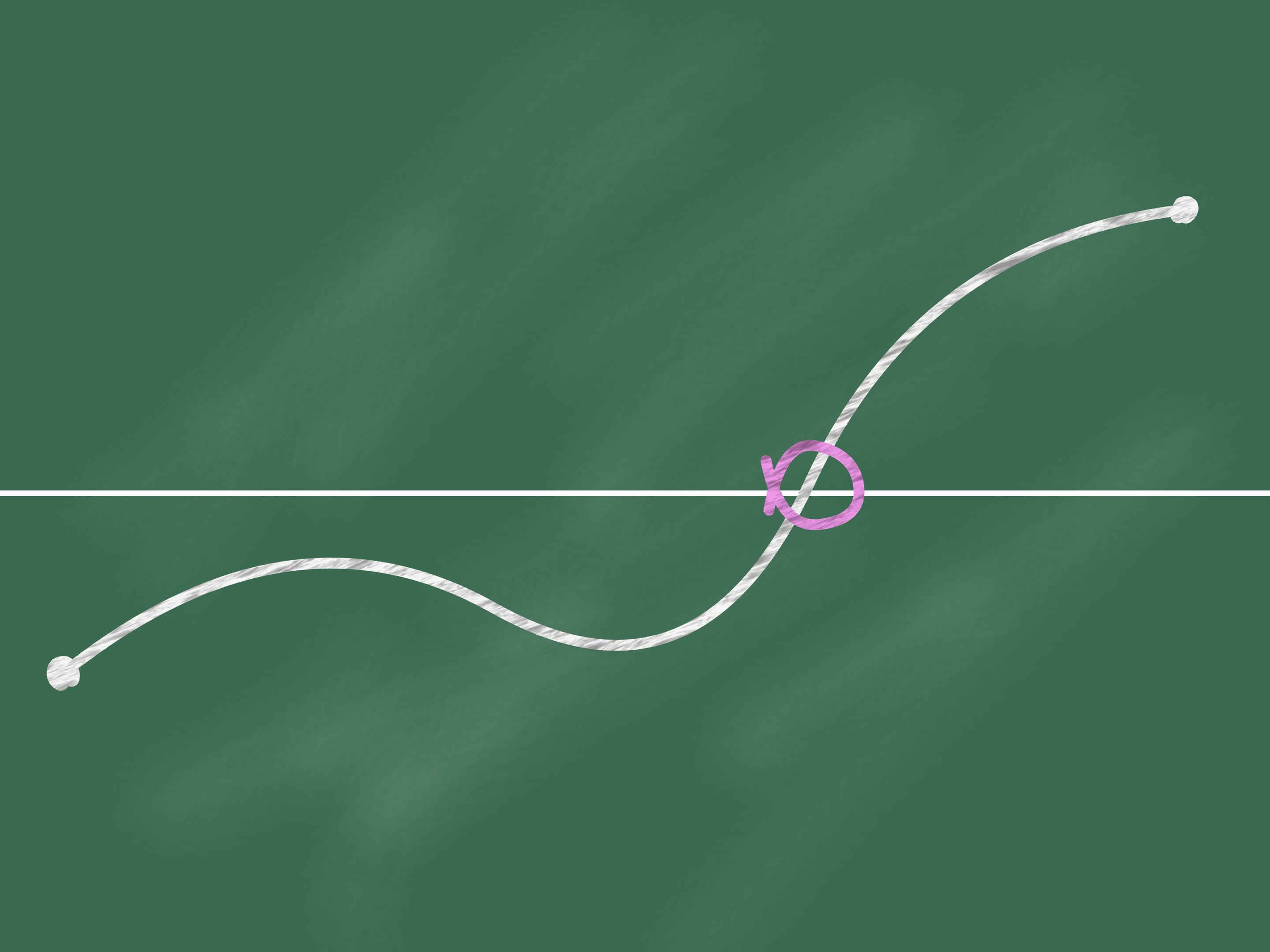
On the black­board draw a hori­zontal line that divides it into two parts. Let us mark two points, one located in the lower part and one at the upper part.

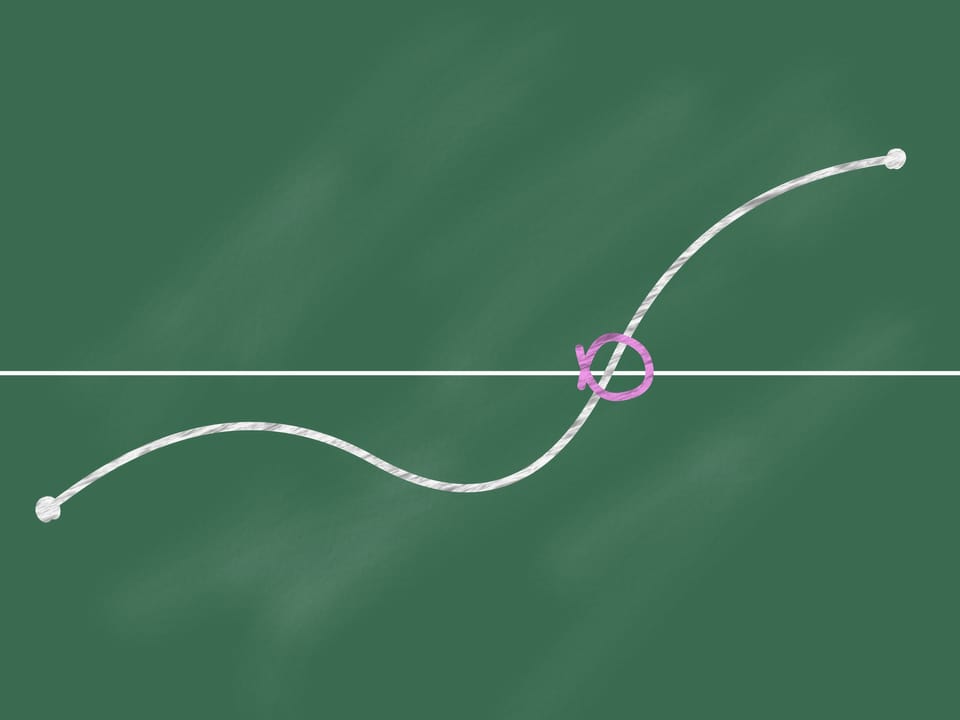
We join these points by a contin­uous line (i.e. not moving away the chalk from the black­board). Then at some point (which can be not unique) our line inter­sects the hori­zontal line.

Should you think that this fact even obvious for chil­dren can be useful in math­e­matics? Despite the apparent obvi­ous­ness, this propo­si­tion is a theorem, namely the Bolzano-Cauchy theorem, and requires a demon­stra­tion.

We will not give here the proof of this theorem, but we will observe only that all its assump­tions are impor­tant, i.e. neces­sary. If the line is not contin­uous (i.e., if it were allowed to remove the chalk from the board) it is obvious that we could jump from the bottom to the top of the black­board, without crossing the hori­zontal line. If we did not consider the inter­sec­tion with the hori­zontal line (which repre­sents the set of all real numbers), but, for example, the inter­sec­tion with the set of rational numbers only, then again the inter­sec­tion could be avoided.

The most surprising thing is that this seem­ingly childish obser­va­tion is a very powerful tool used in the proof of some math­e­mat­ical propo­si­tions. The draw­back is that the proof is not construc­tive: the line some­where cross the hori­zontal line, but at what point exactly, given a precise contin­uous line, it is impos­sible to say using this theorem.

Some exam­ples of the Bolzano-Cauchy theorem will be shown in the films of Section “Conti­nuity”.