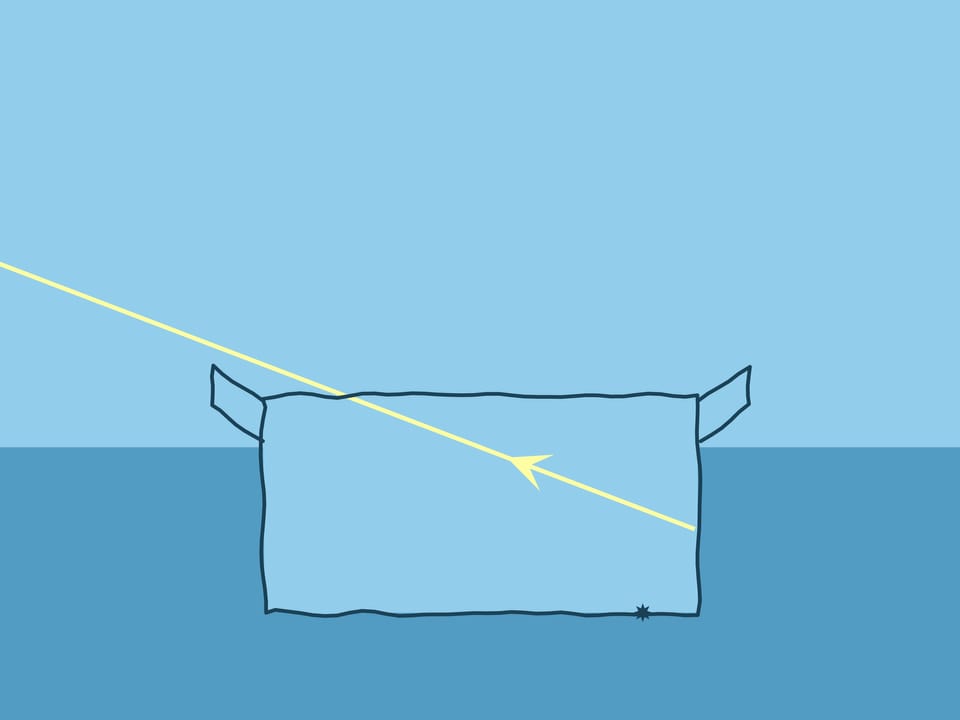
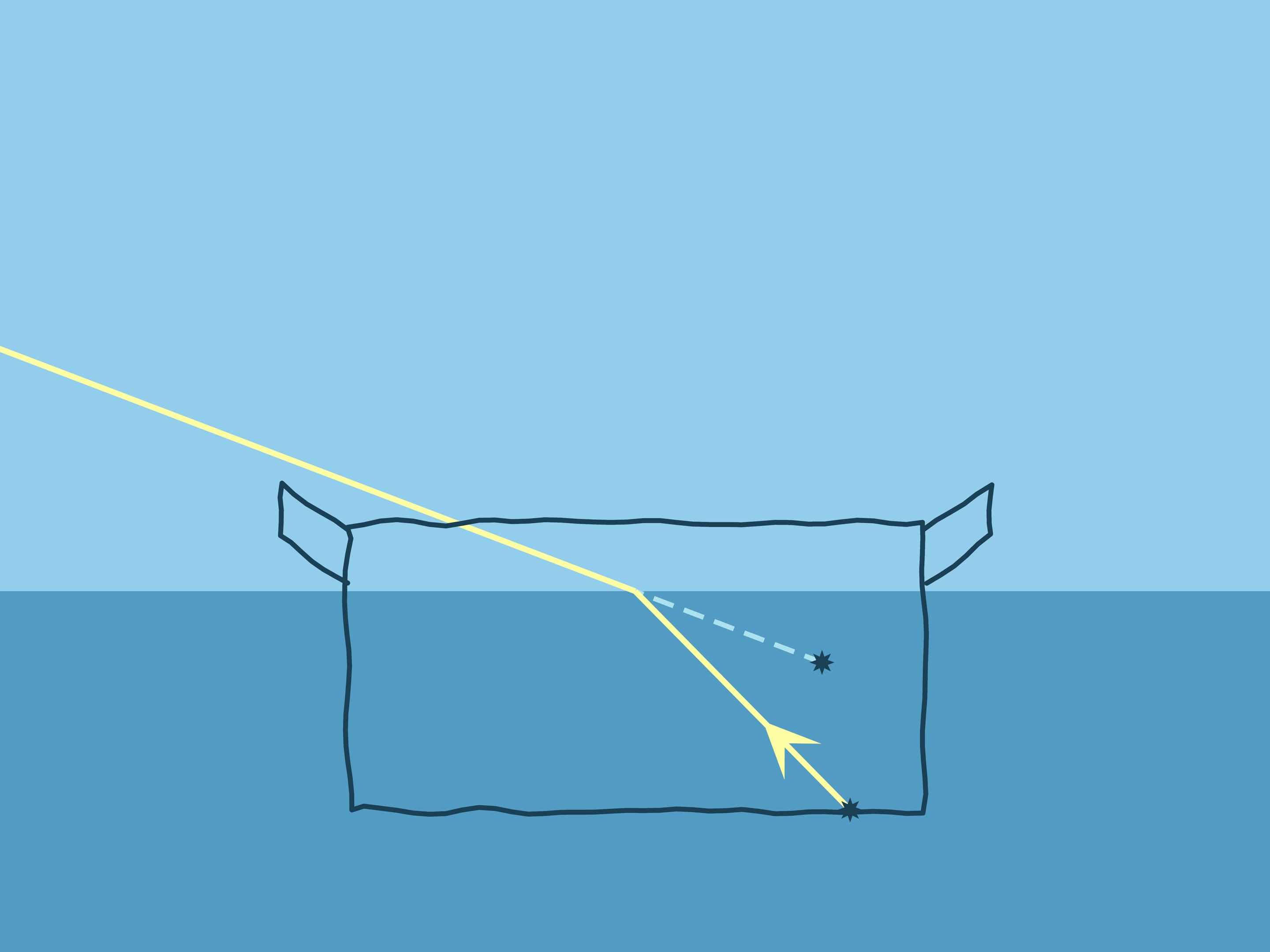
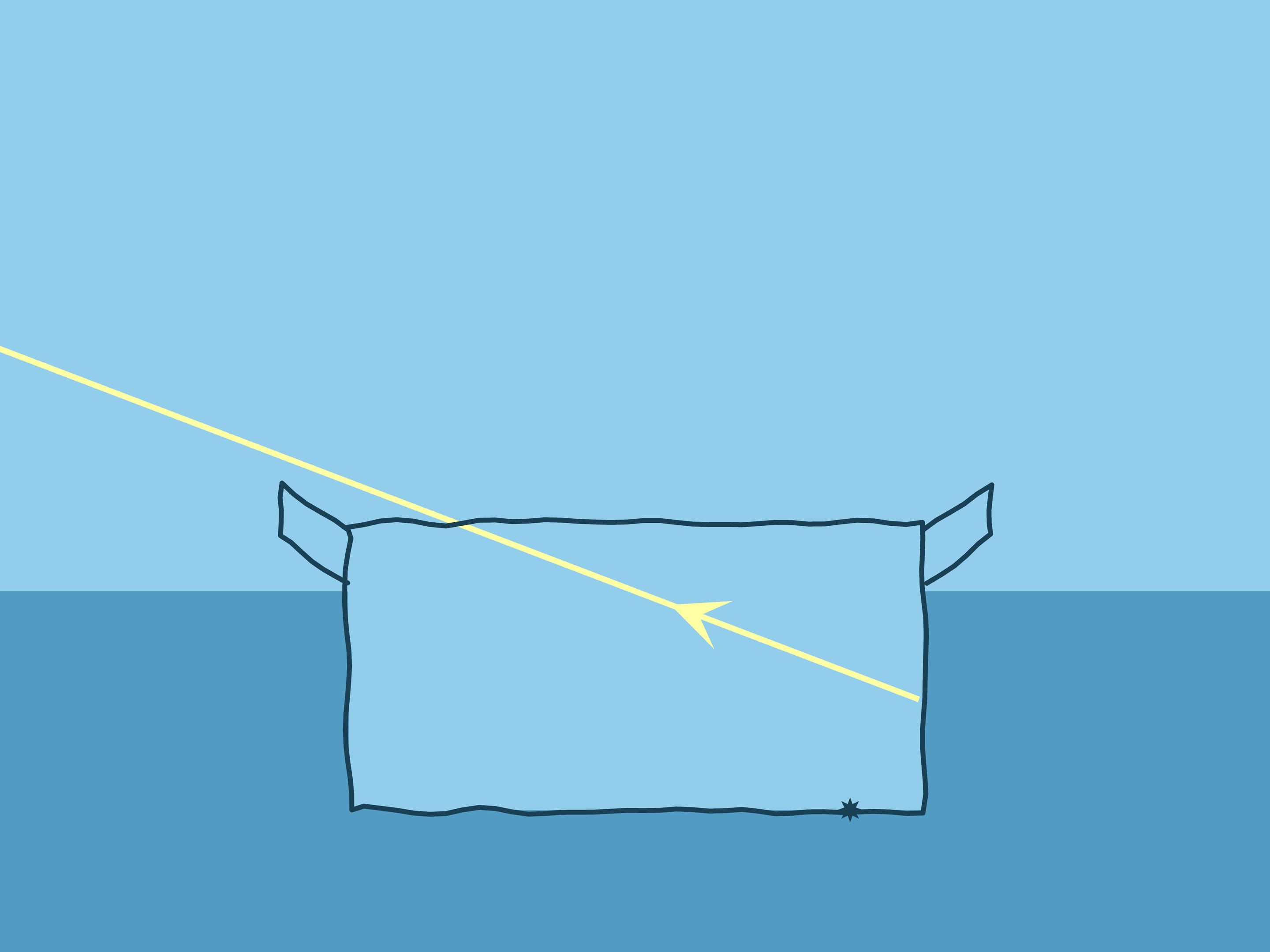
Which pot seems to be deeper, the empty one or filled with water? Do an experiment — take a pot, put a coin on the bottom to make it clearer, position the pot so that the coin is out of sight, and keeping the pot still, start pouring water…
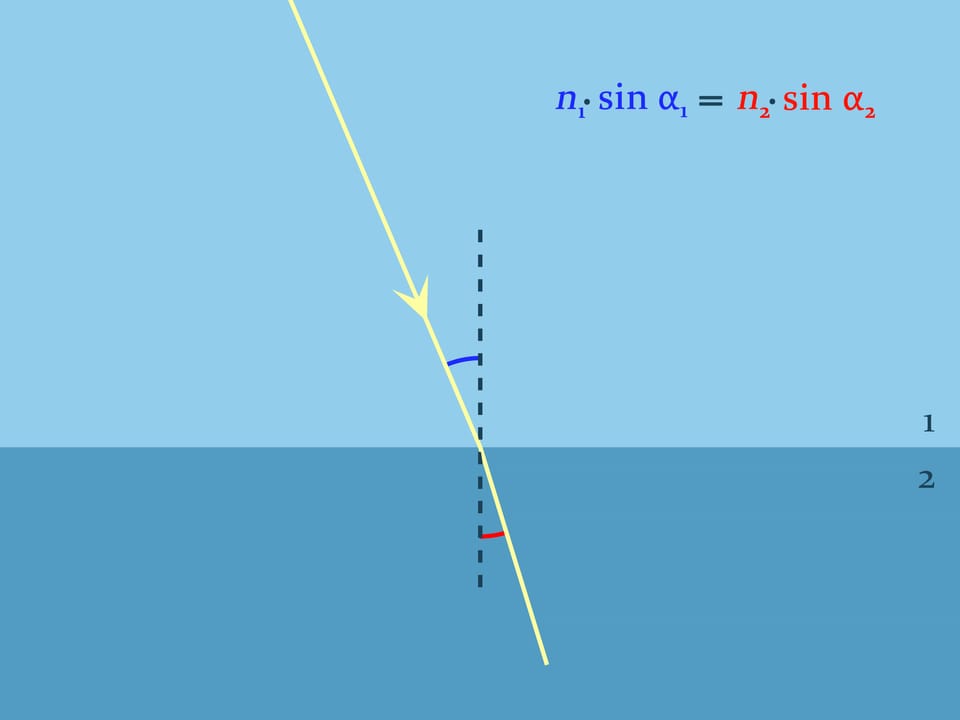
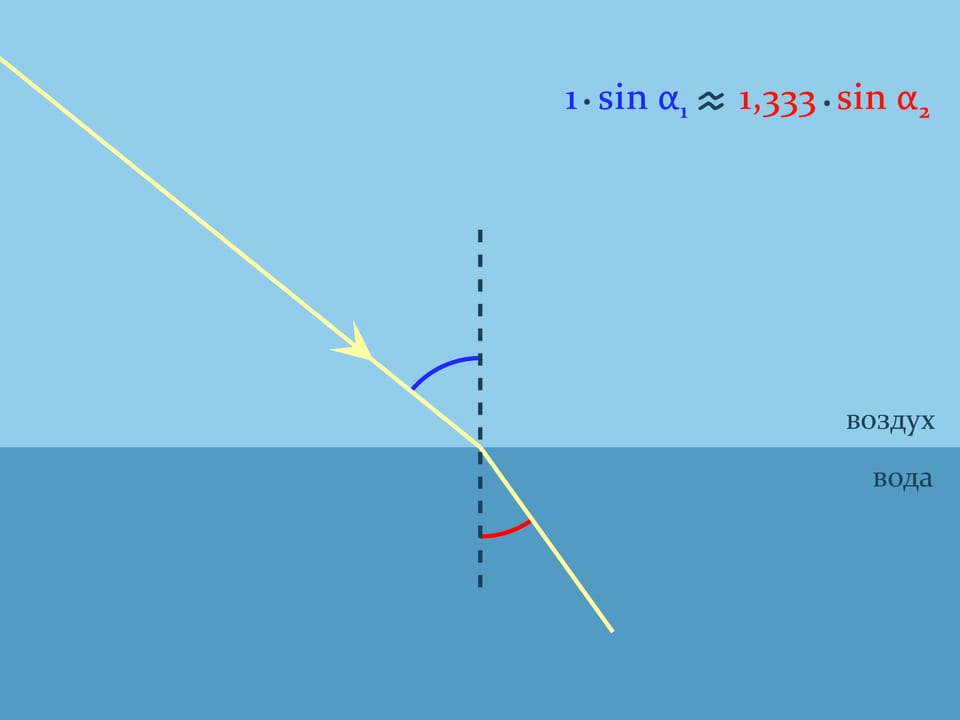
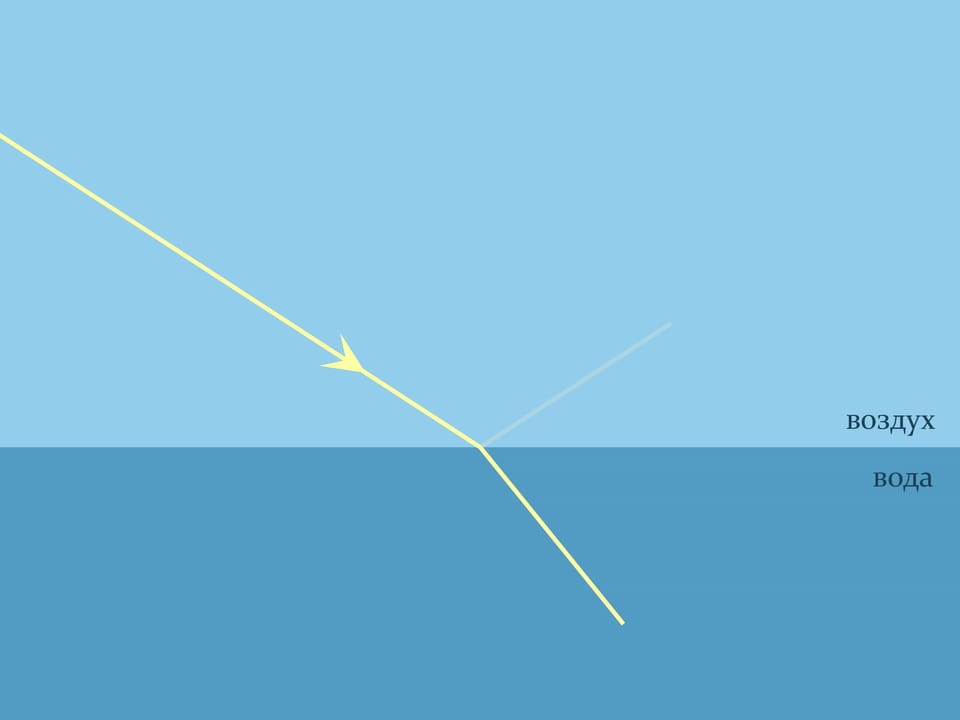
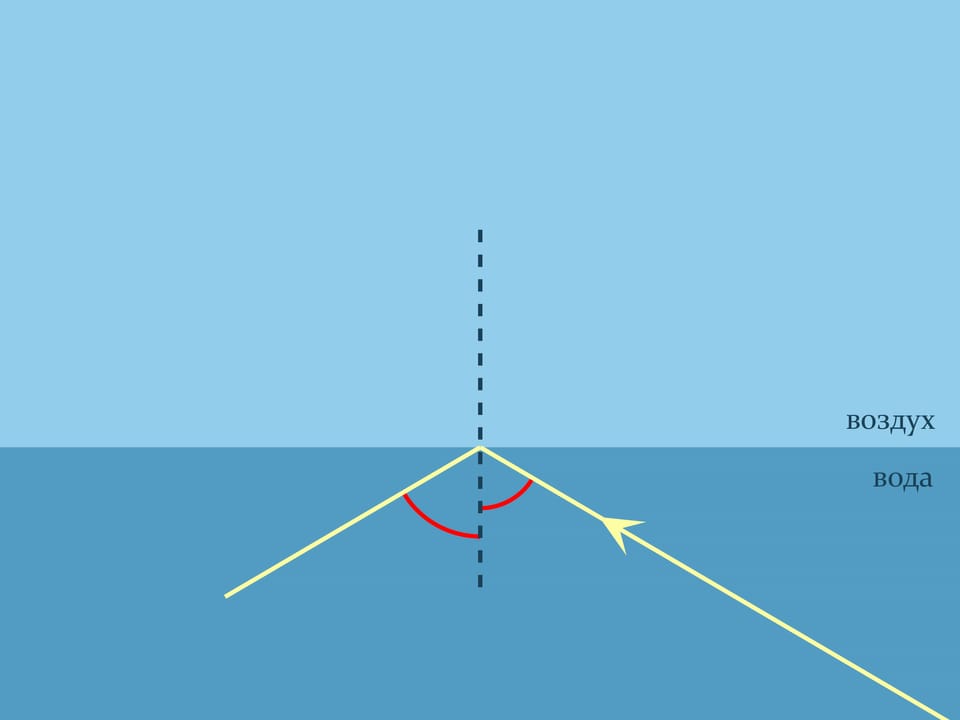
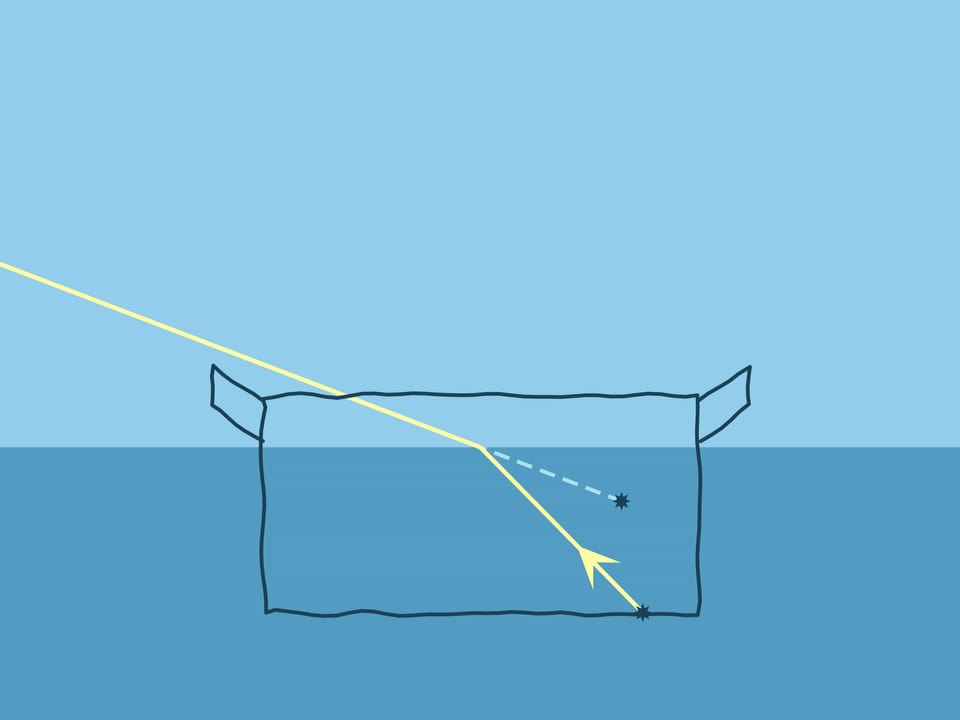
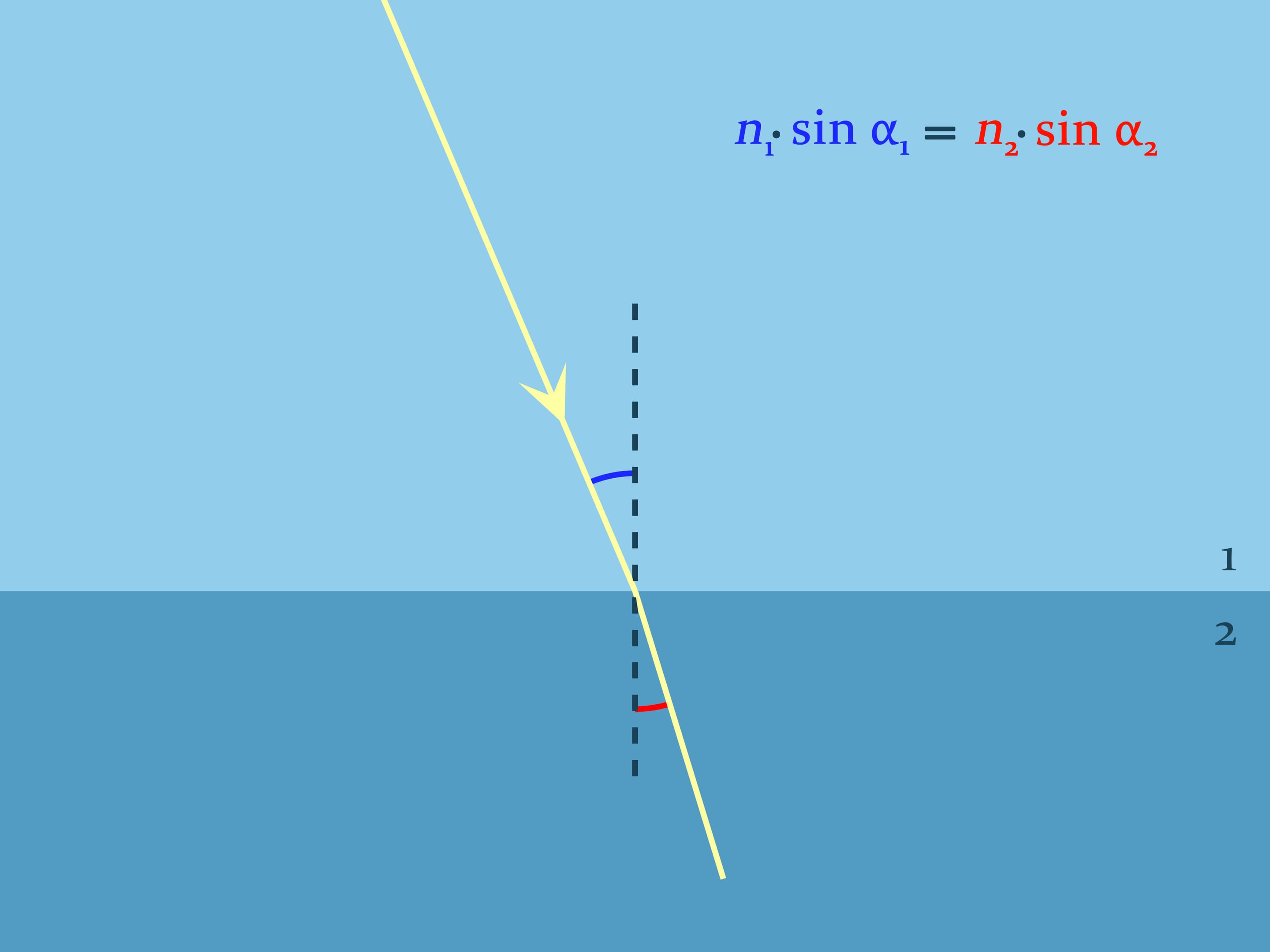
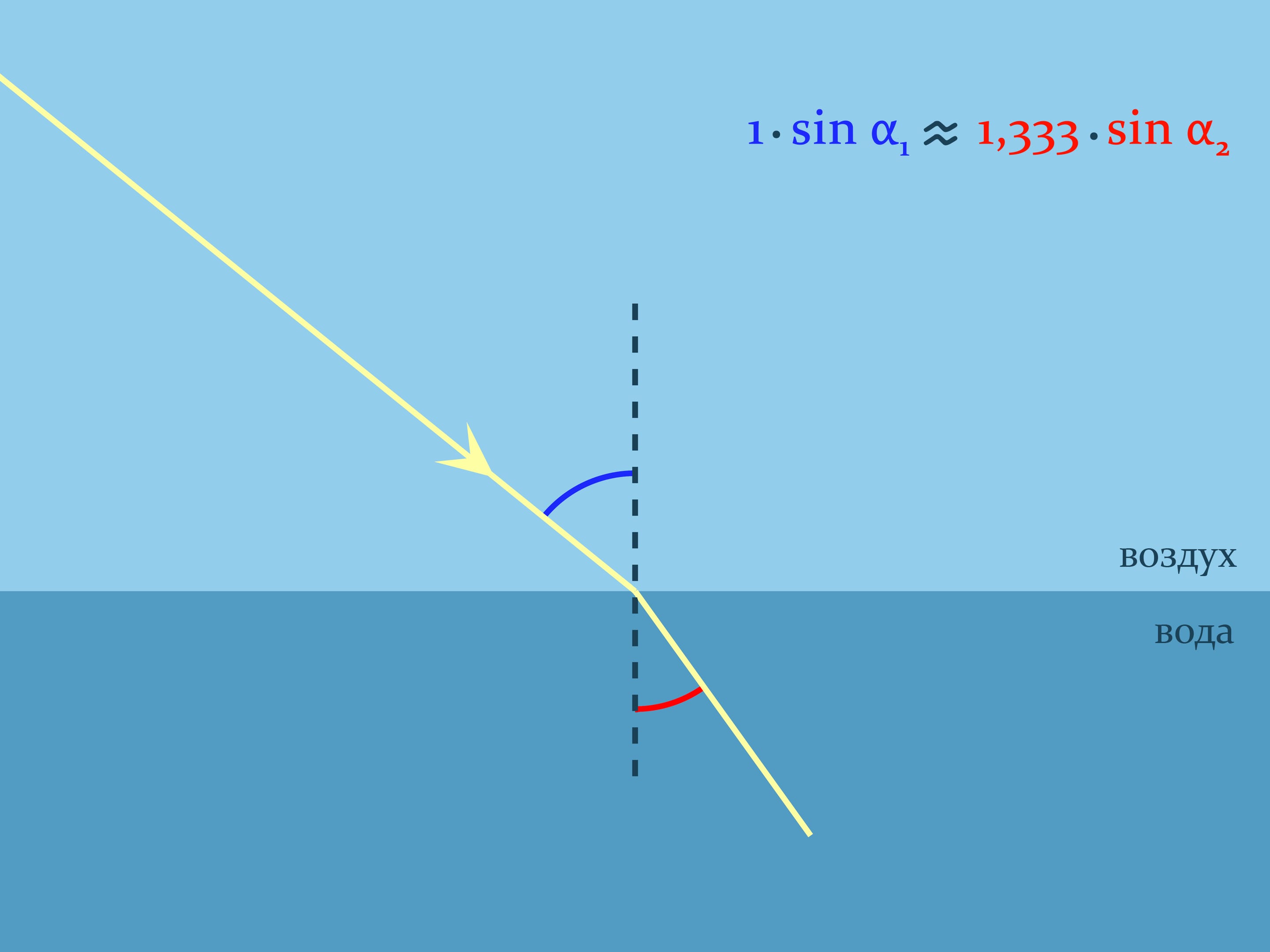
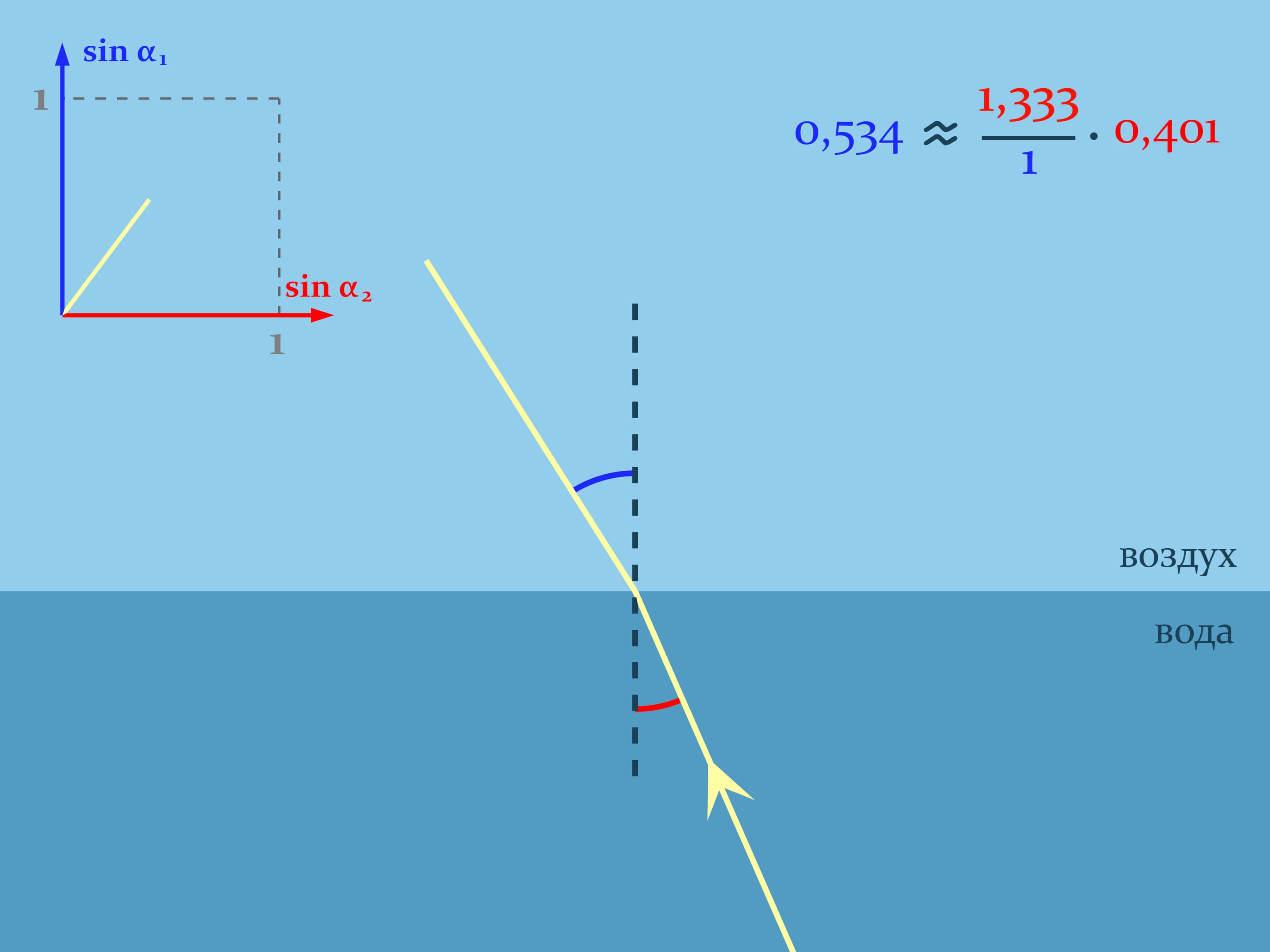
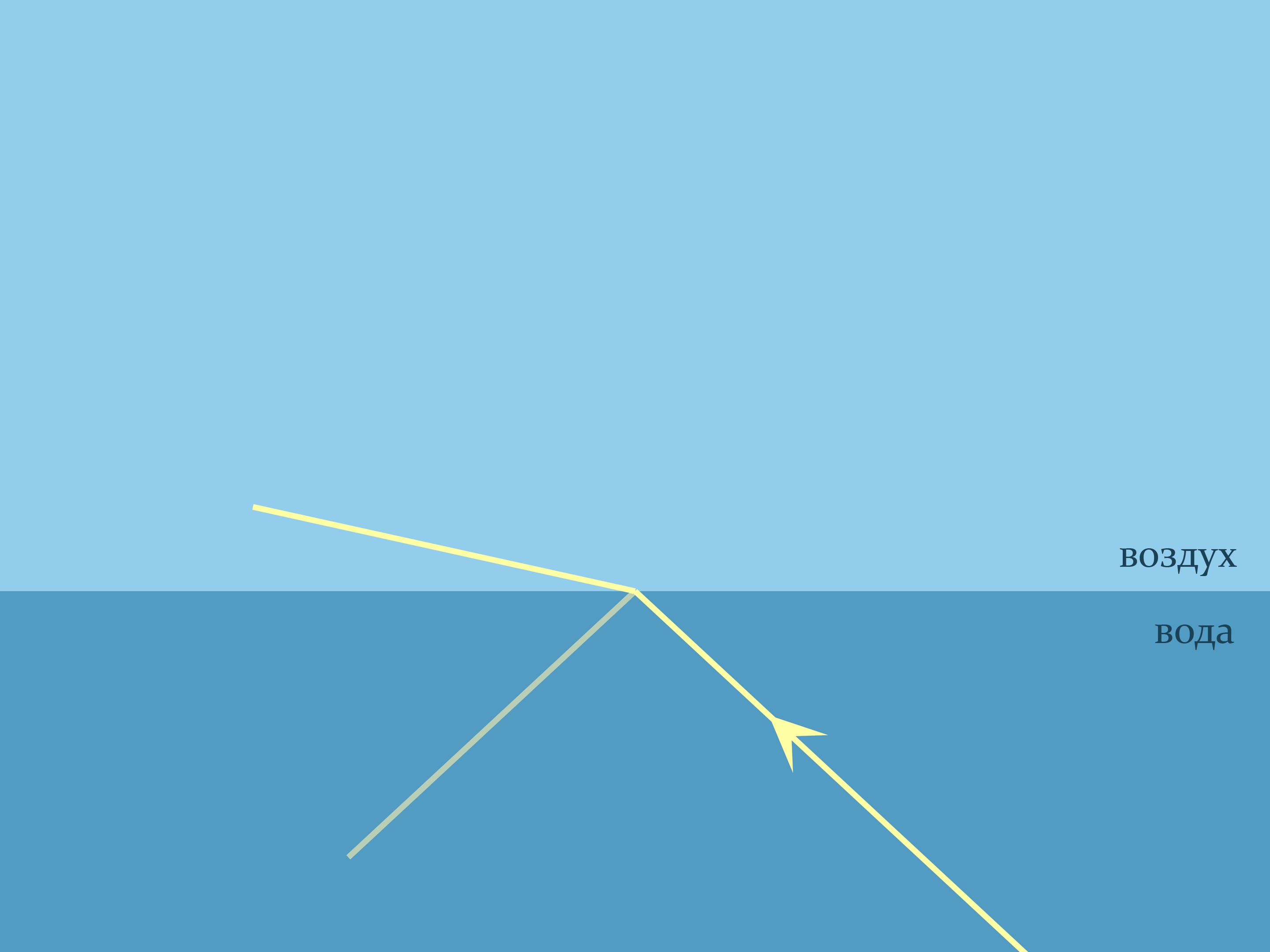
The refraction law of light of geometric optics, named after the Dutch mathematician, physicist and astronomer W. Snell, helps to substantiate what you see. It explains how light is refracted when it passes between two optically divergent media. Snell’s law states how refraction will occur: $n_1\sin\alpha_1= n_2\sin\alpha_2$, where $n_1$ and $n_2$ are the refractive indices of the media, describing how many times the speed of light in the medium is lower than the speed of light in a vacuum, and the angles are counted from the normal to the boundary. For the air the refractive index is nearly equal to $1$, and, for example, for the water at room temperature $n\approx1{,}333$.
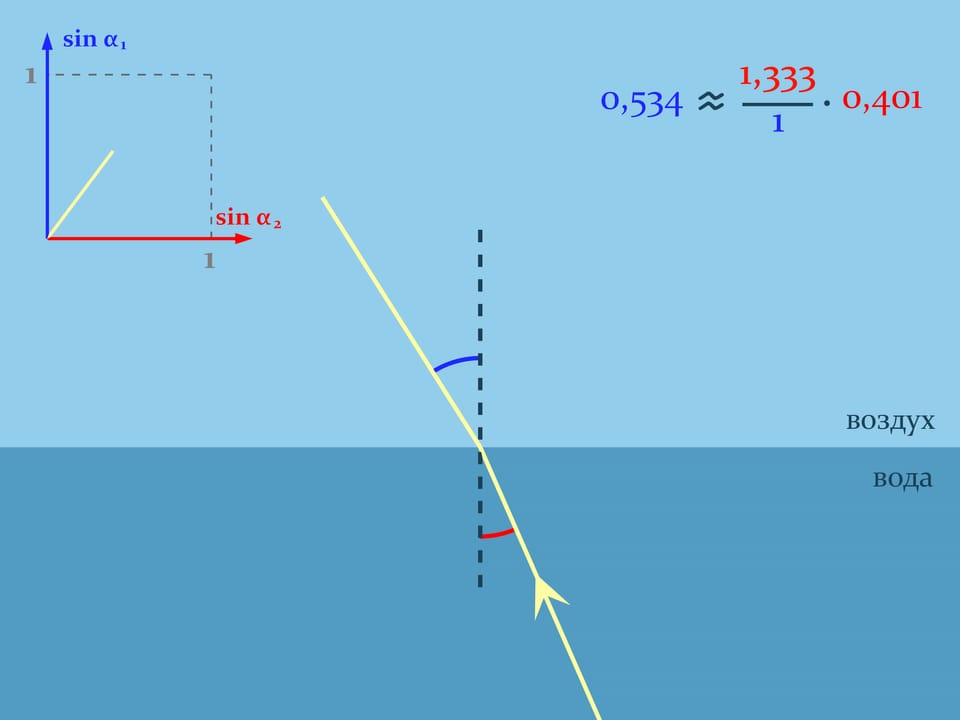
It seems that both the picture and the formula are symmetric — if the ray in water is emitted, it should travel along the same path as the ray coming from the air. But that is not always true.
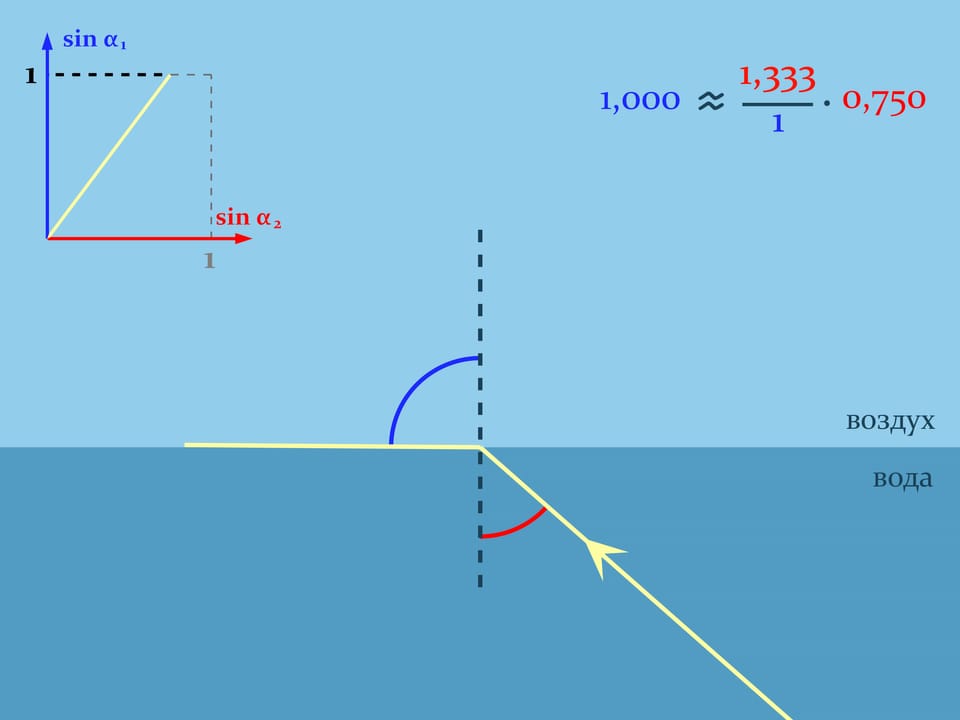
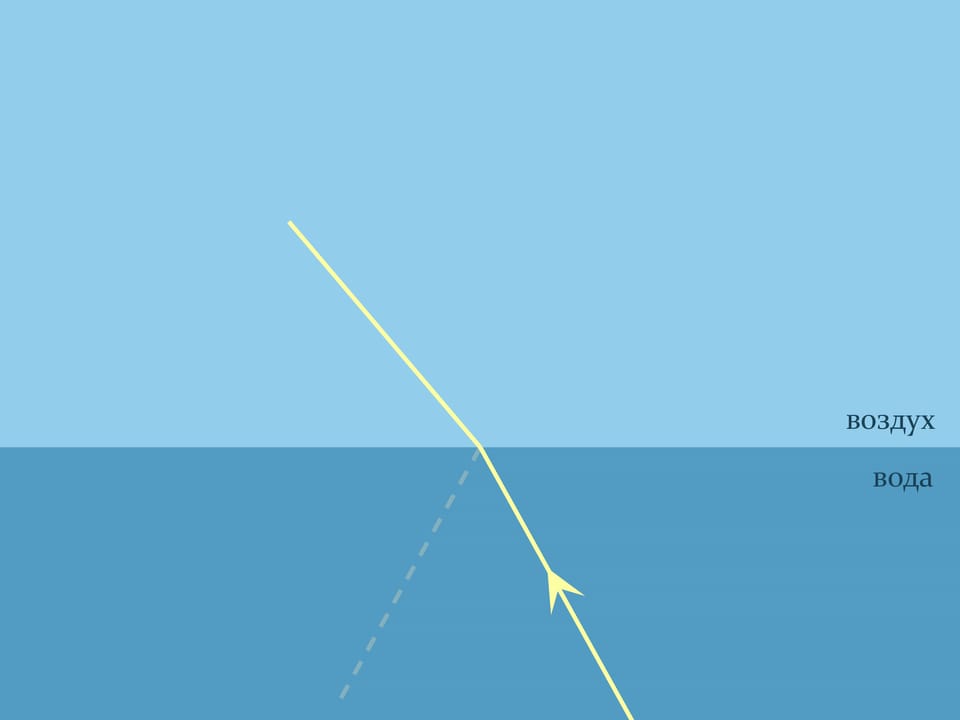
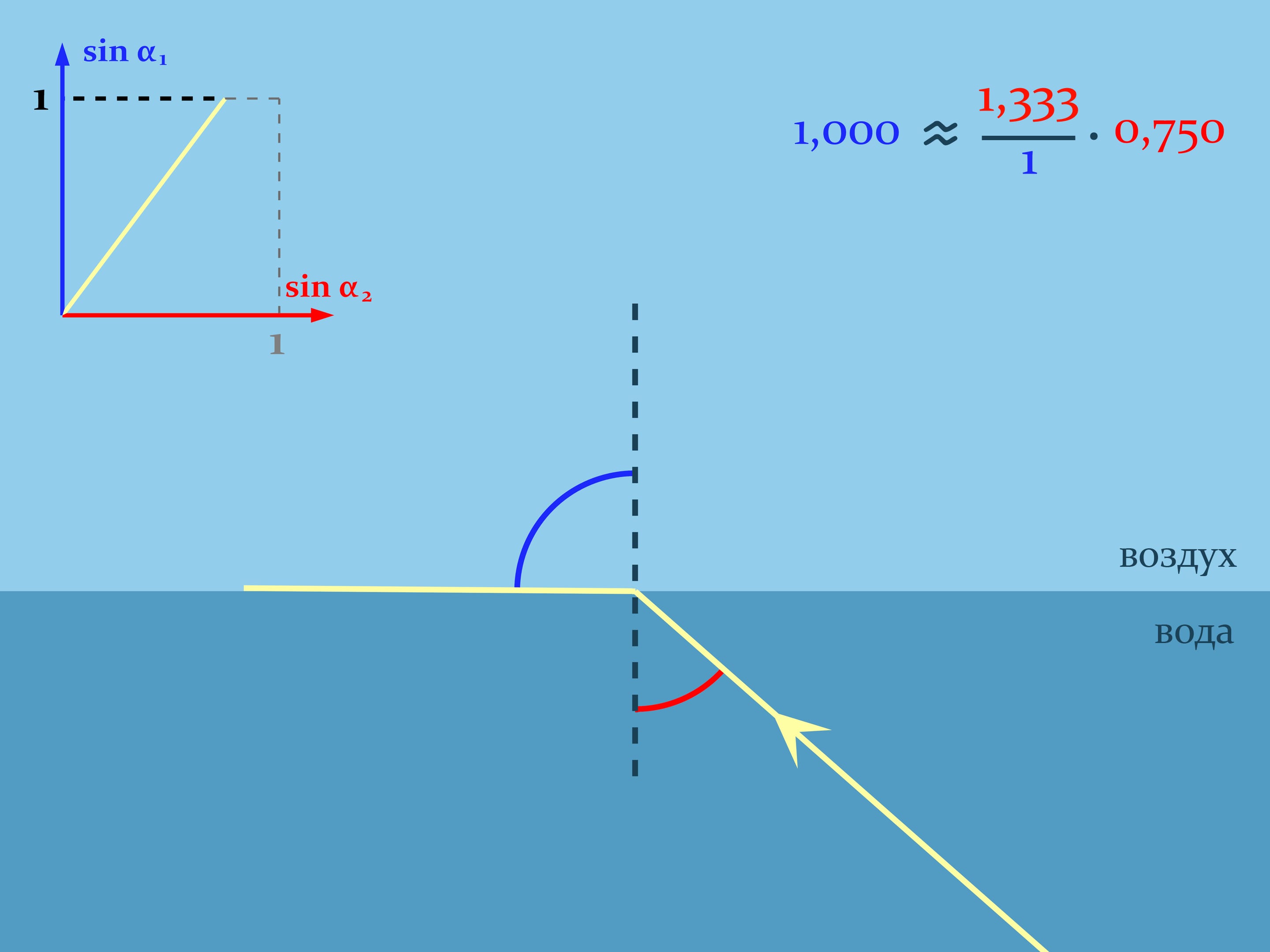
Let the ray out of water and watch the graph of the function that links the sines of the angles: $\sin\alpha_2=\frac{n_1}{n_2}\sin\alpha_1$. When the sine of the angle in water will be $\approx 3/4$, an exit angle of the ray in the air will be $90^\circ$, and consequently, its sine will be equal to one. And the sine of the angle in water will be $0{,}75$ and continue to increase. What would happen if we continue to increase the angle between the normal and the ray in water? A more comprehensive analysis of the ray passage through the media boundary will help to explain it.
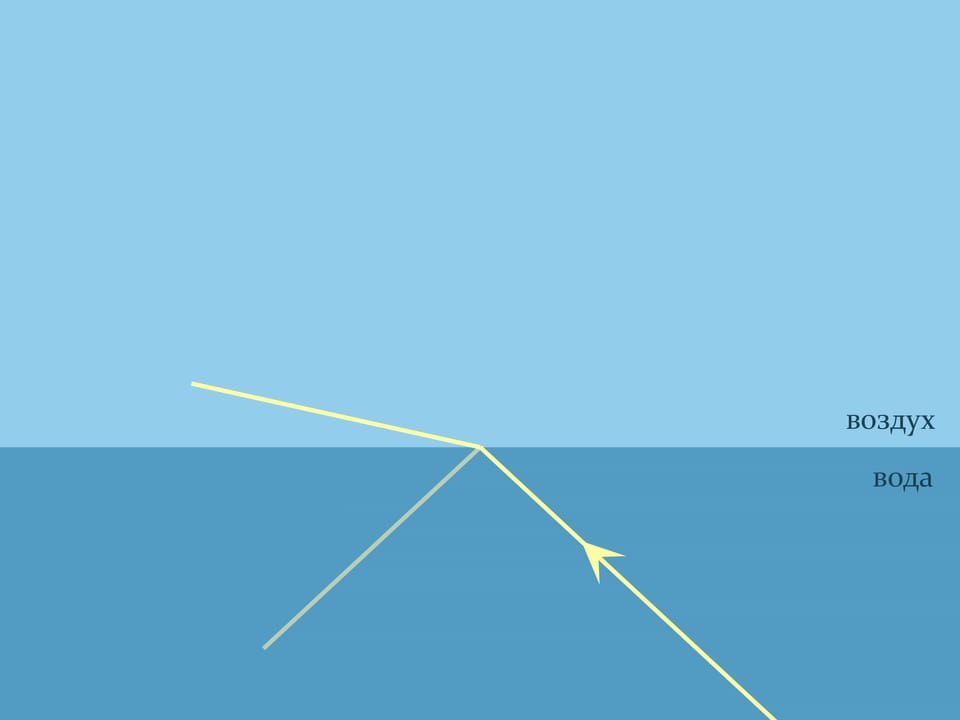
When a beam hits the border of two media, both refraction (according to Snell’s law) and reflection (according to the law the angle of incidence equals the angle of reflection) always occur. The direction where most of the beam goes depends on the angle. And when the ray approaches almost parallel to the boundary from the optically more dense side, a total internal reflection takes place — the whole ray is reflected from the less dense medium like from a mirror.
Having considered Snell’s law at all possible angles of the beam and the normal, let us return to the experiment with the pot. If water is poured, the coin “rises”: if there were no water, the observer would see the wall of the pot at this angle.
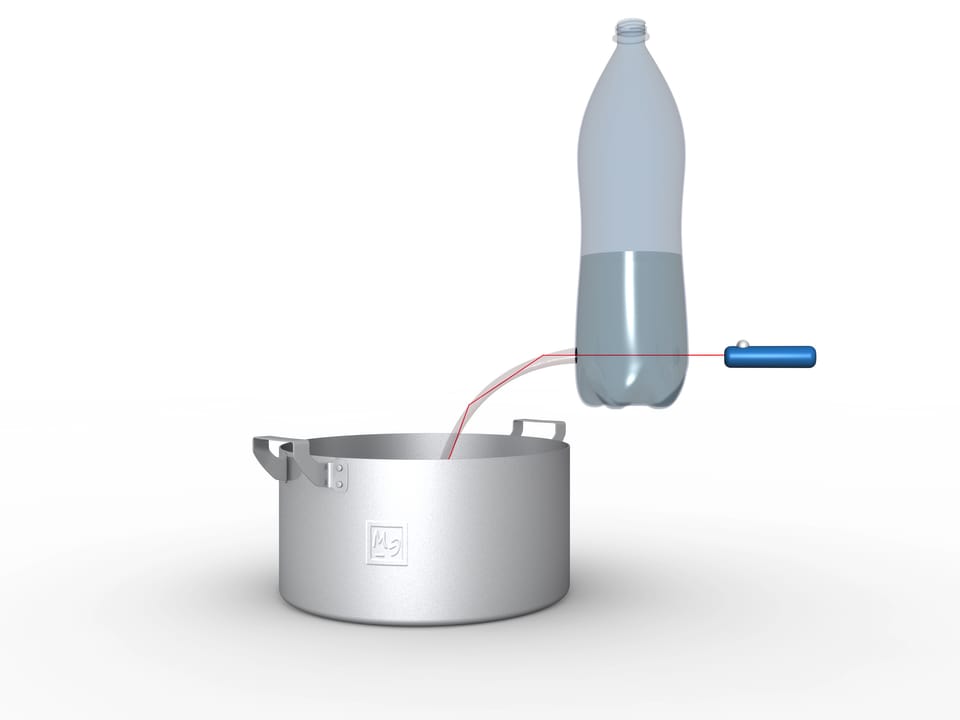
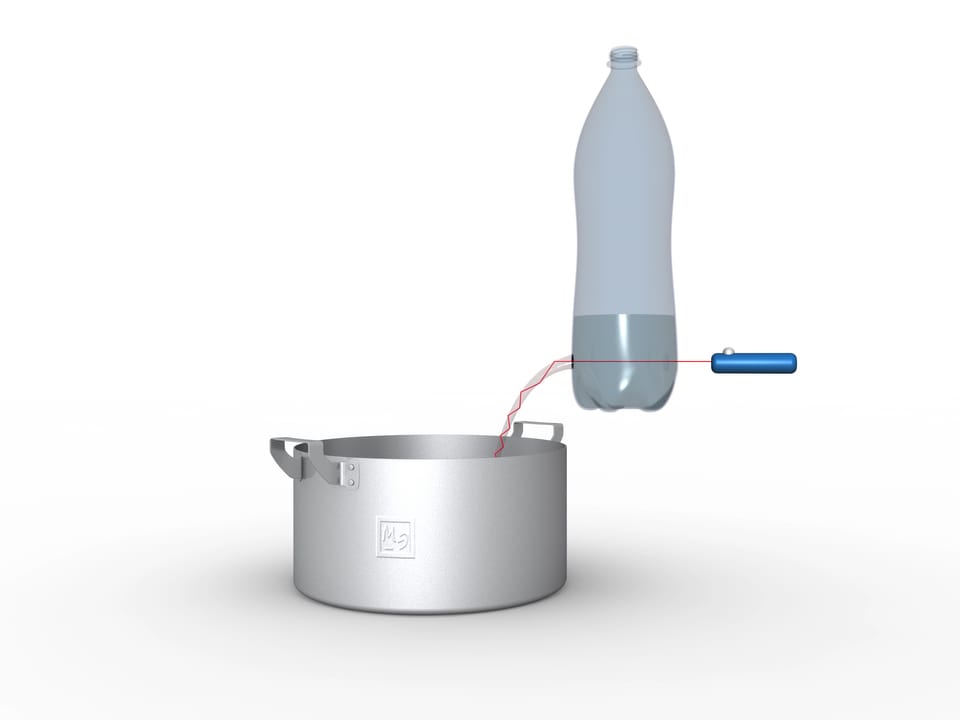
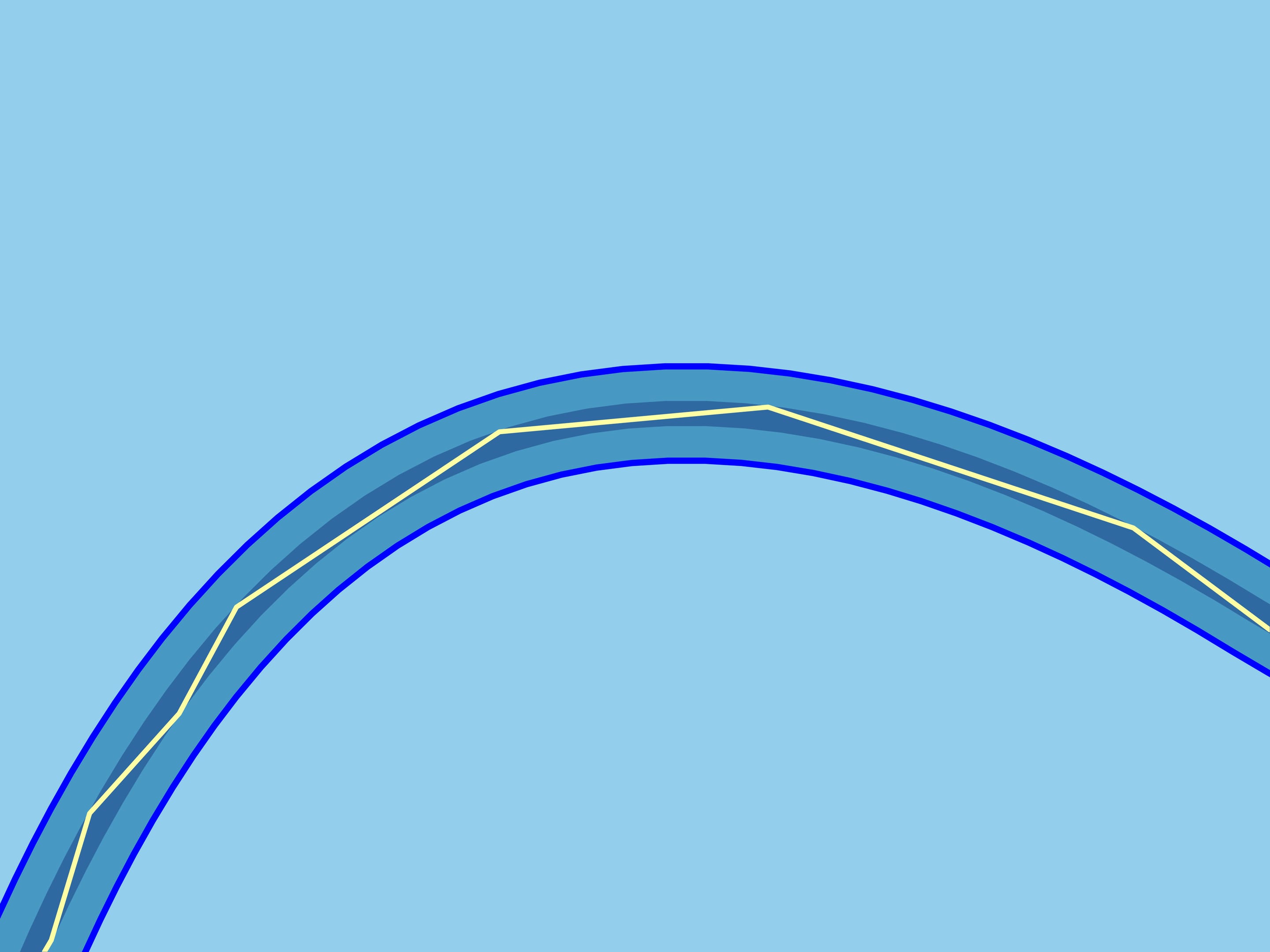
The total internal reflection principle is at the heart of optical fibre, which is used for transmitting signals. The optically denser core is surrounded by a less dense shell. A ray emitted into the core at a small angle to its axis is completely reflected from the shell and cannot leave the core: it goes where the optical fibre directs it.
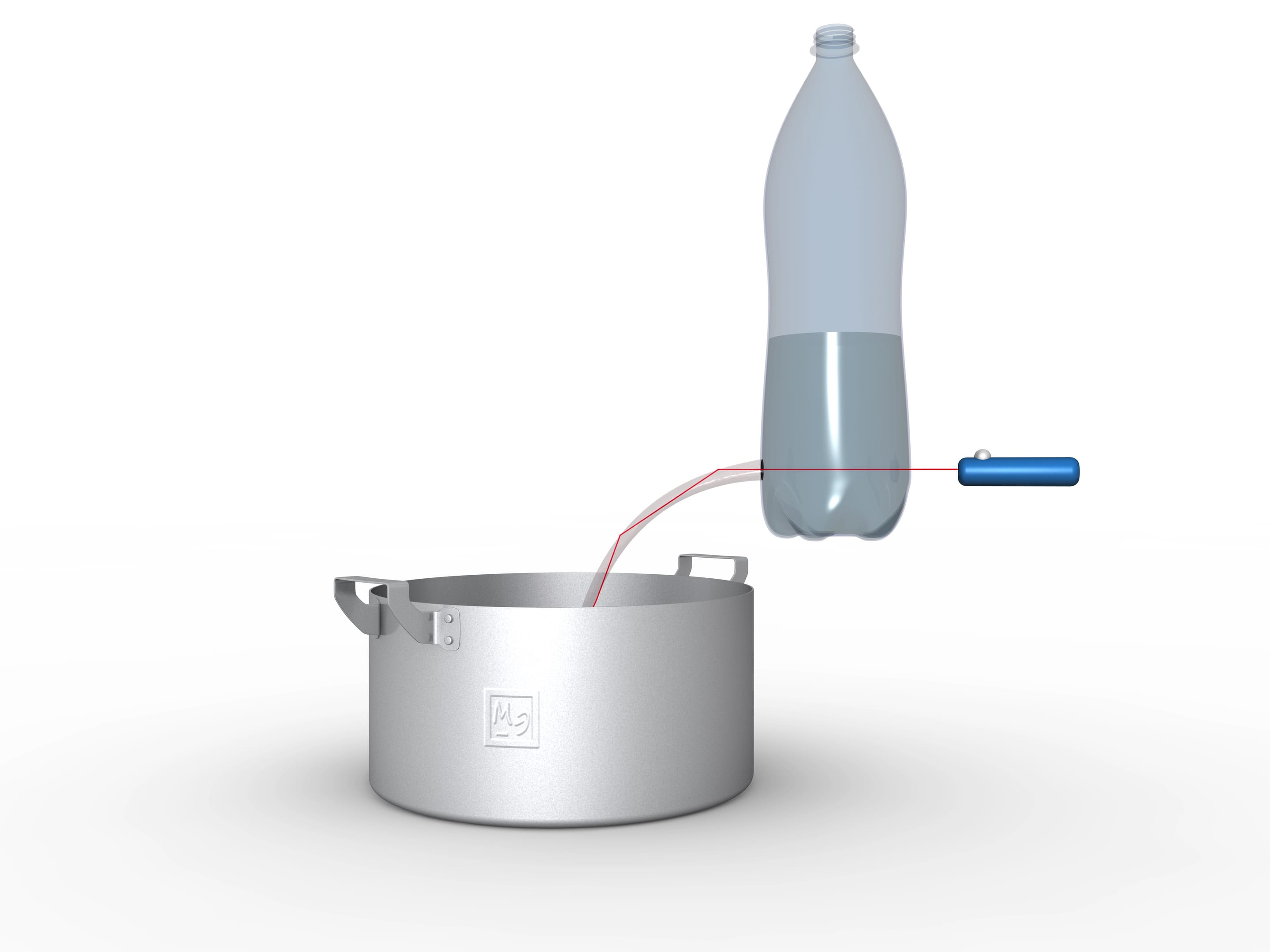
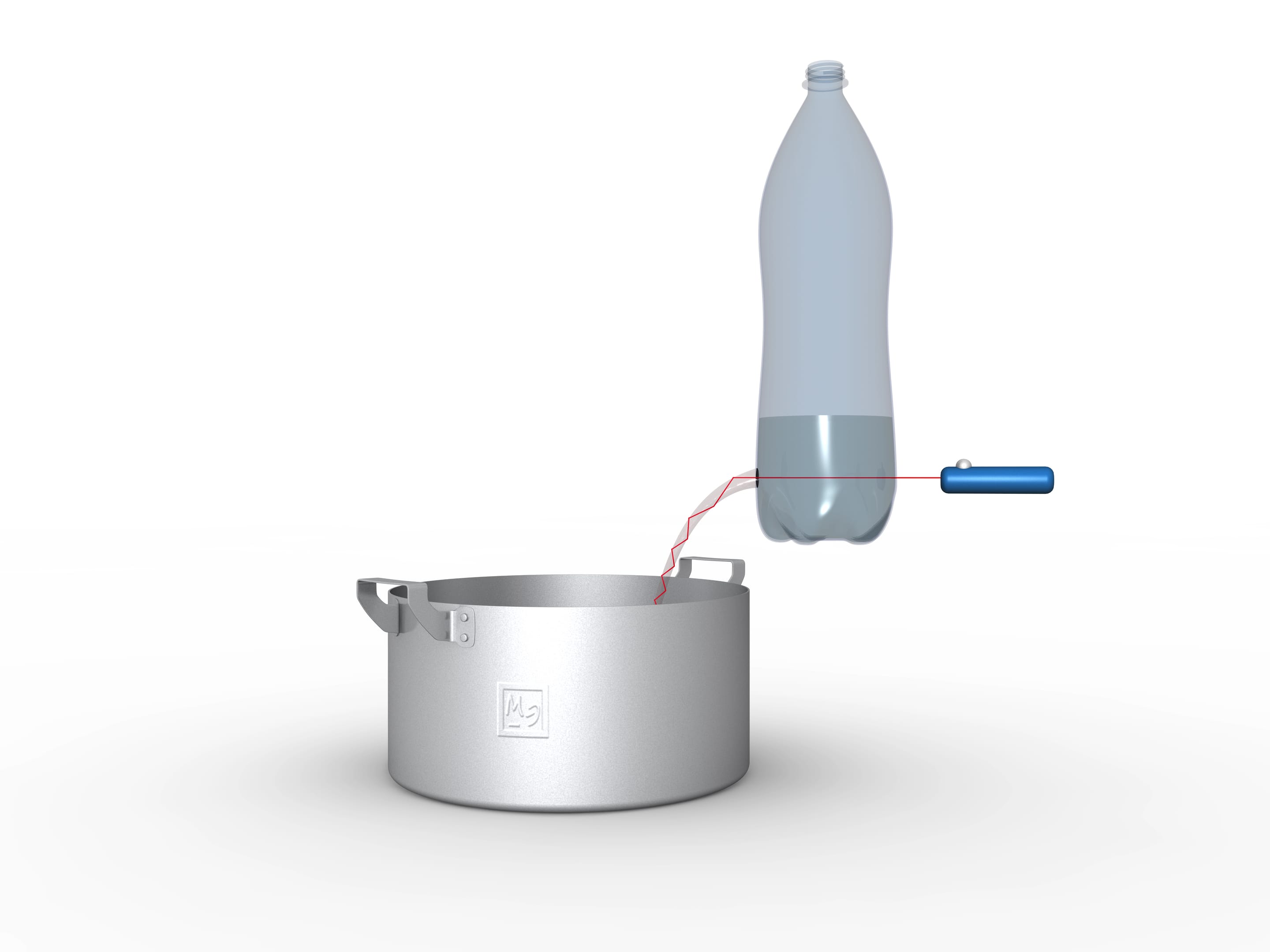
It is not difficult to make a working light tube at home. Make a round hole in the bottom of a clear plastic bottle, pour water in, and direct the laser pointer ray into the flowing stream. You’ll see the glowing polyline follows the changing shape of the stream. If you add a little color to the water, not only will the stream glow, but the water inside the pot or sink will glow as well.