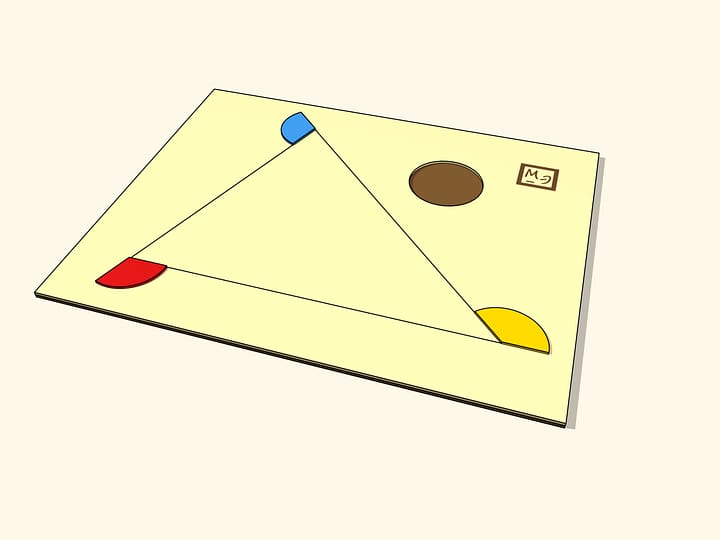
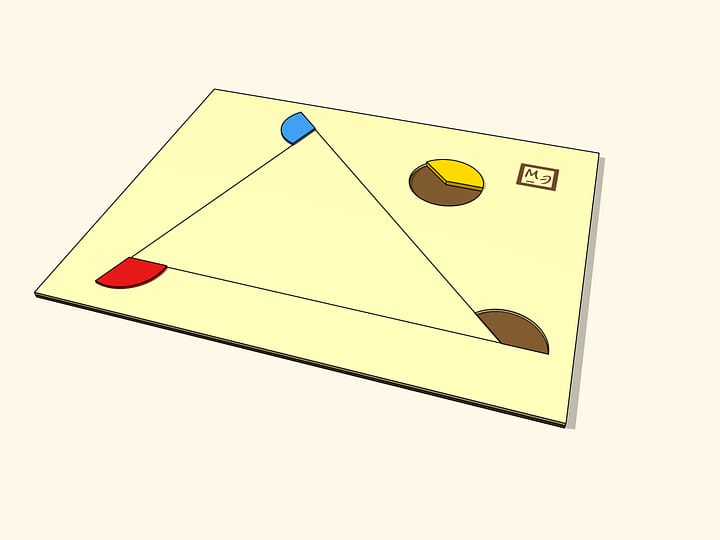
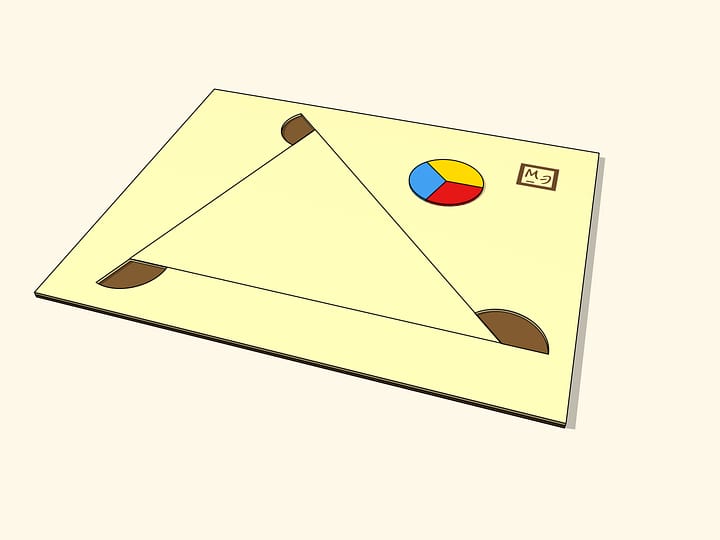
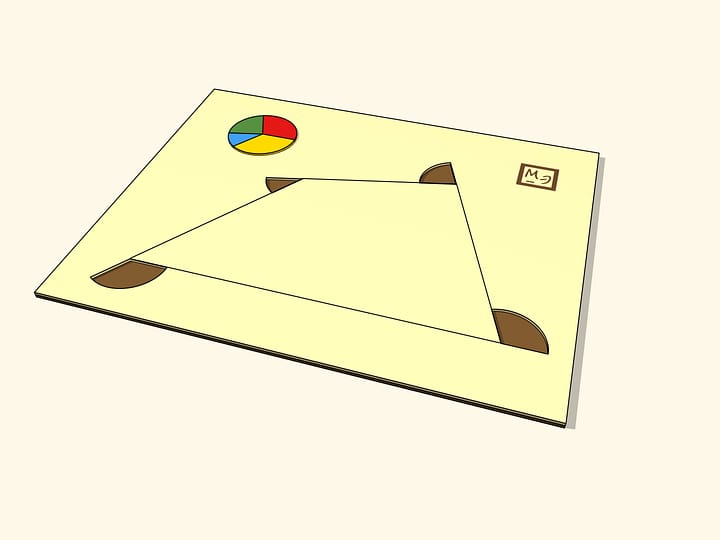
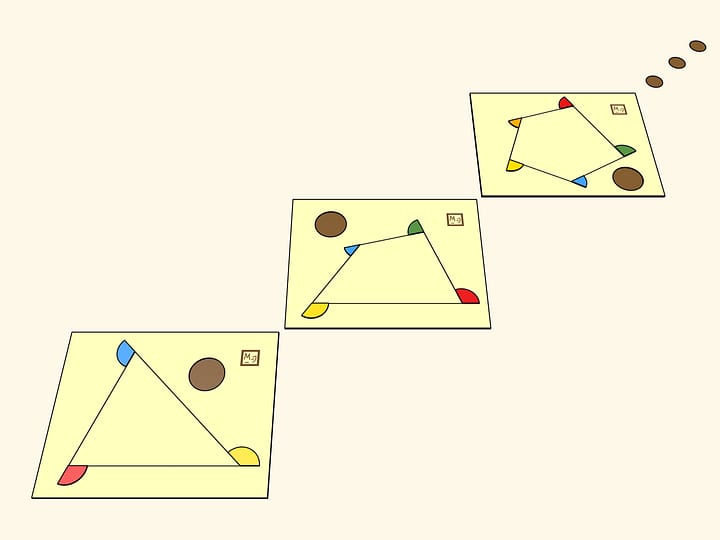
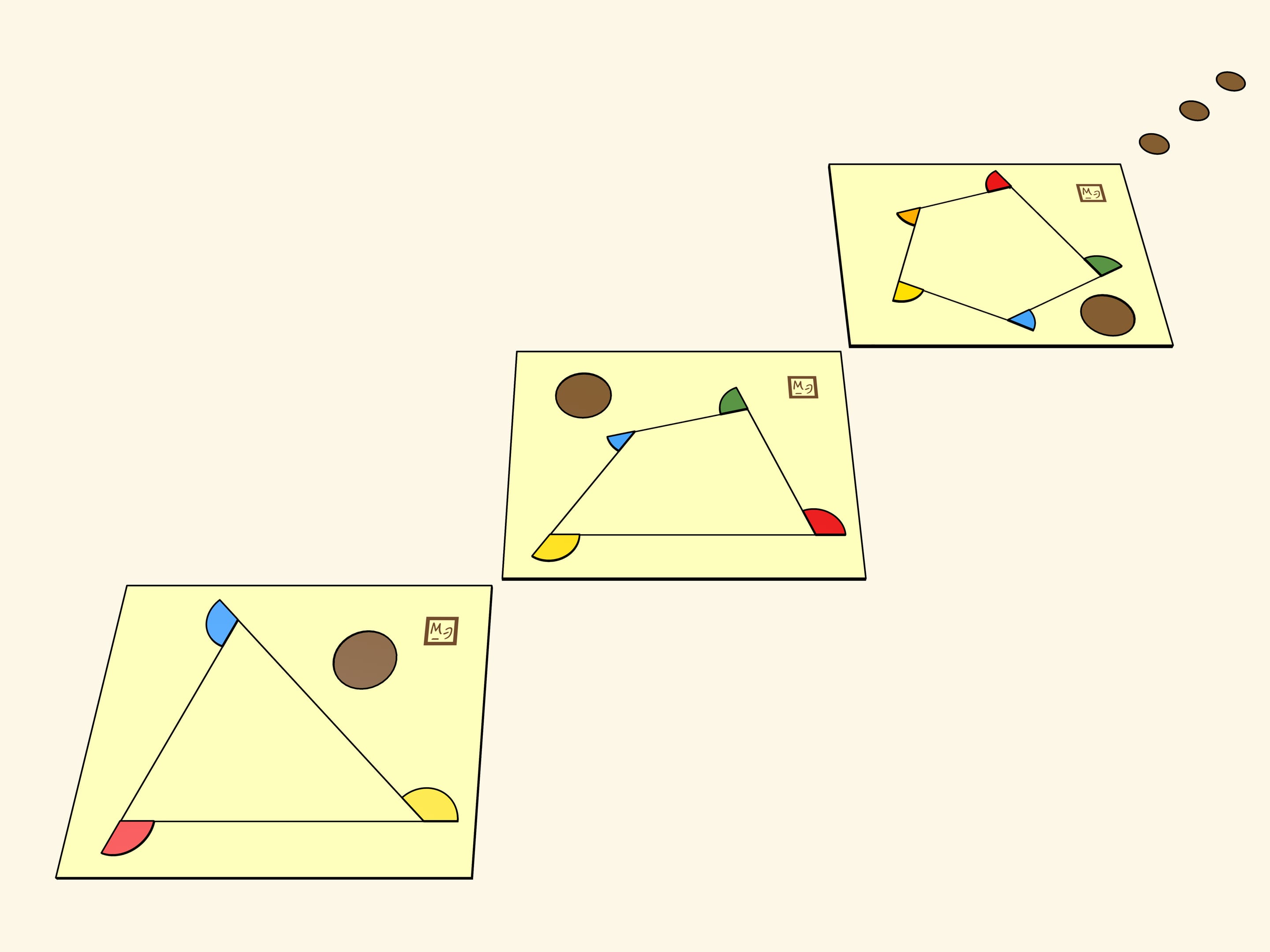
A model demonstrating the theorem on the sum of exterior angles of a convex polygon can be made of two sheets of cardboard or plywood.
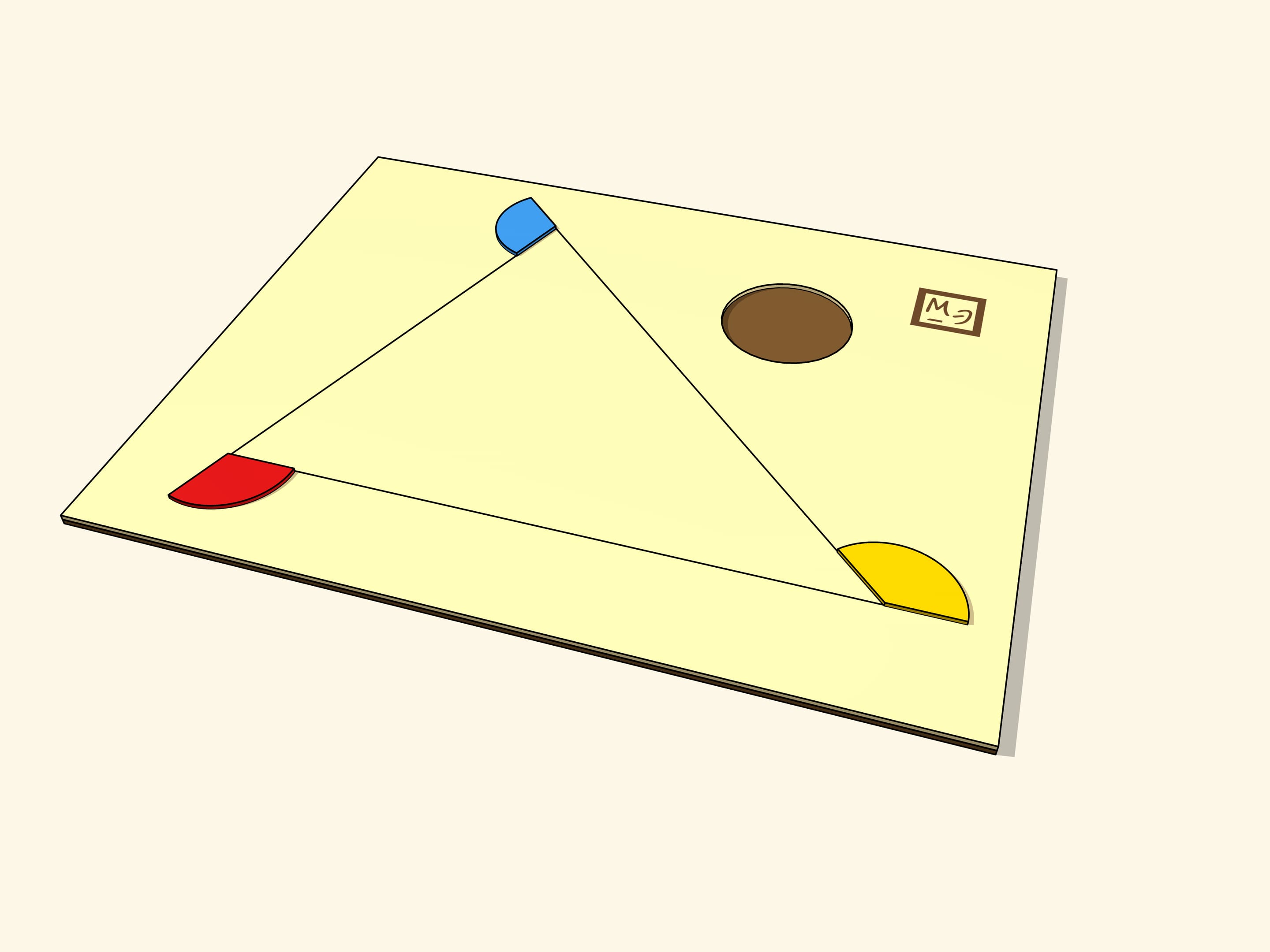
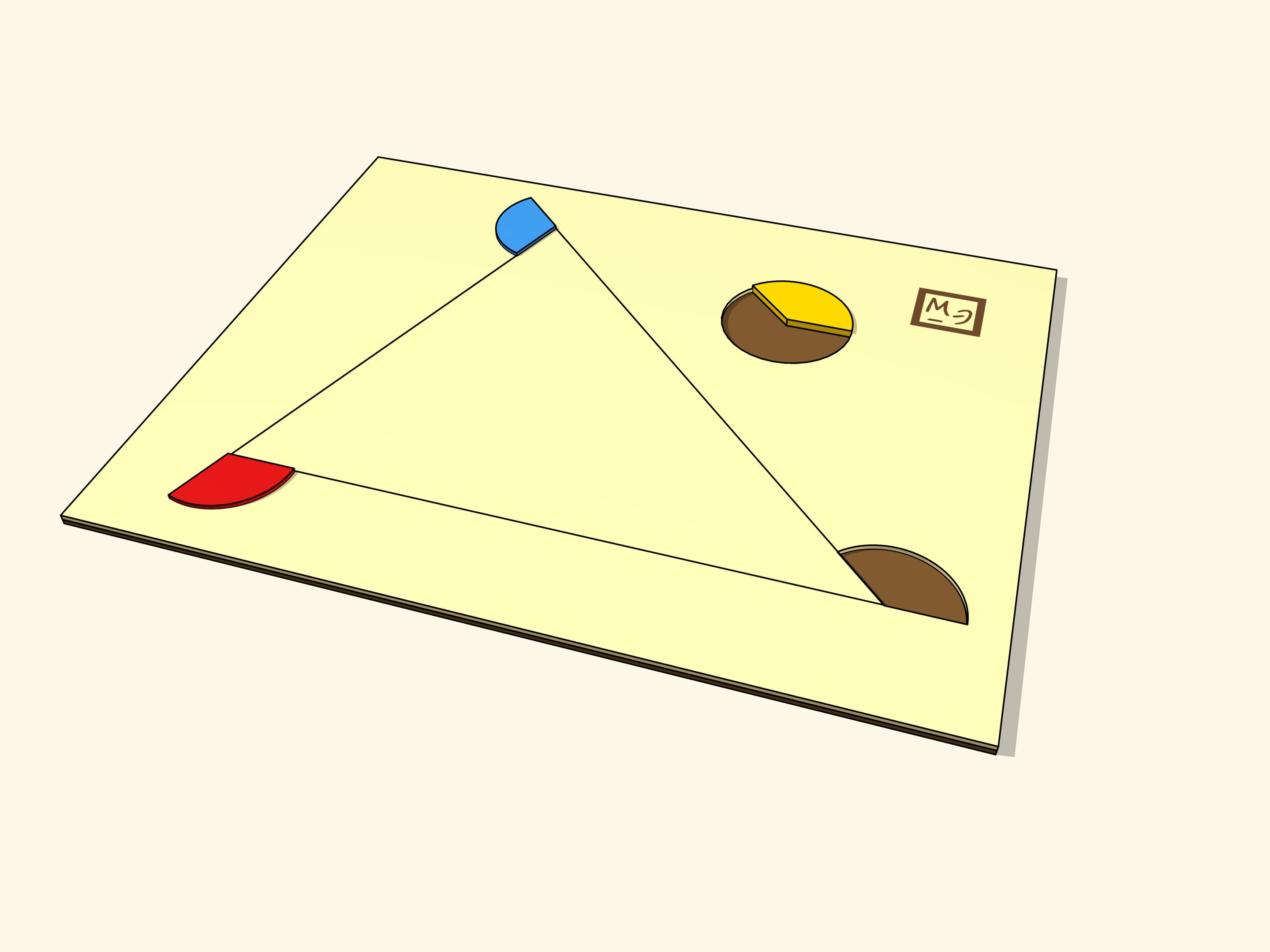
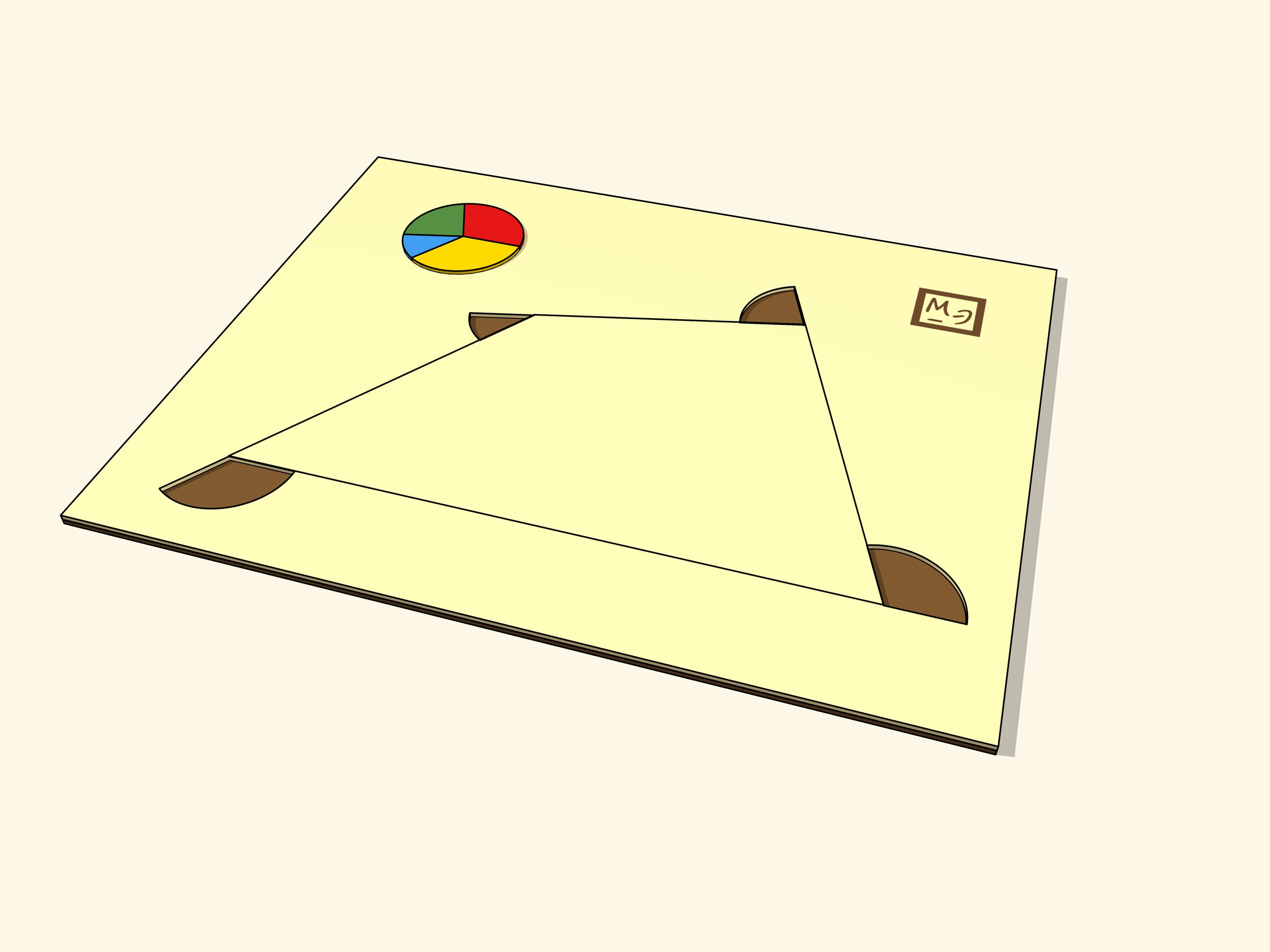
The bottom layer is a rectangular base. A convex polygon is drawn on the top layer with equal radius sectors cut out at its exterior angles. A circle of radius as that of the sectors is also carved out of the top layer. One can put all the sectors together to make sure they fill the circle. Interestingly, it even isn't necessary to rotate the sectors when moving them to the circle — translating is enough.
It is better to make the sector pieces twice as thick as the top layer so that taking them out was more convenient.