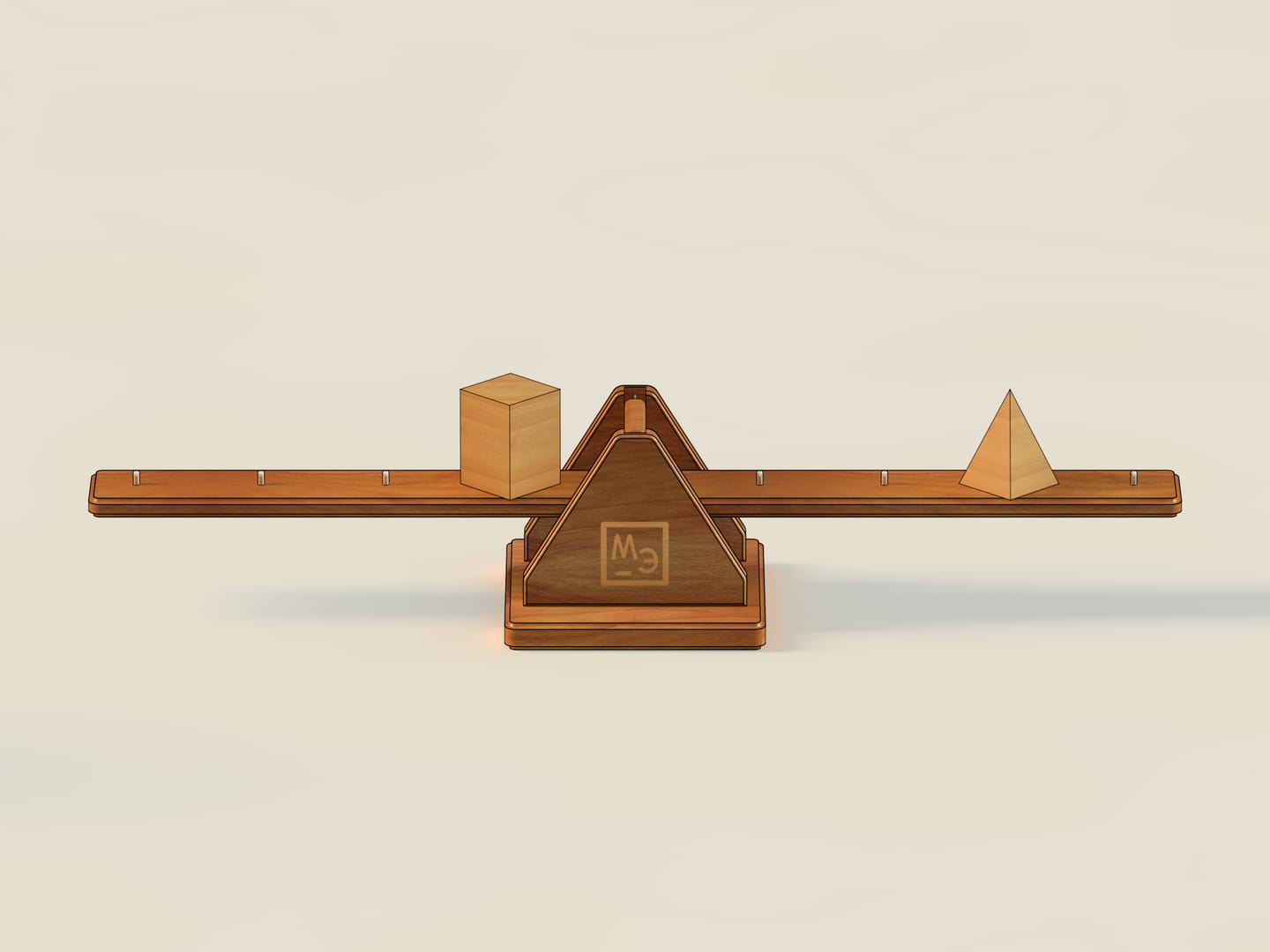
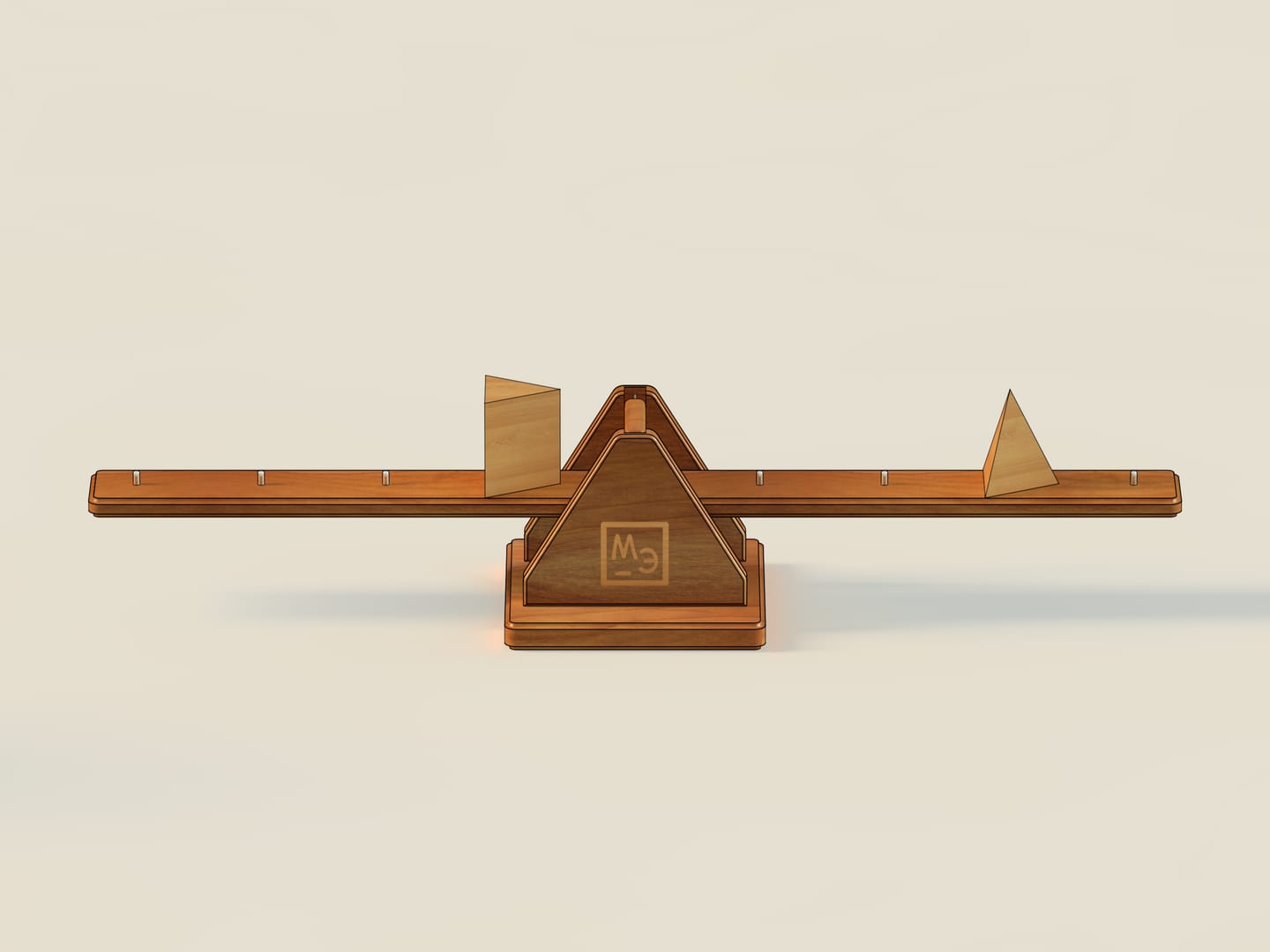
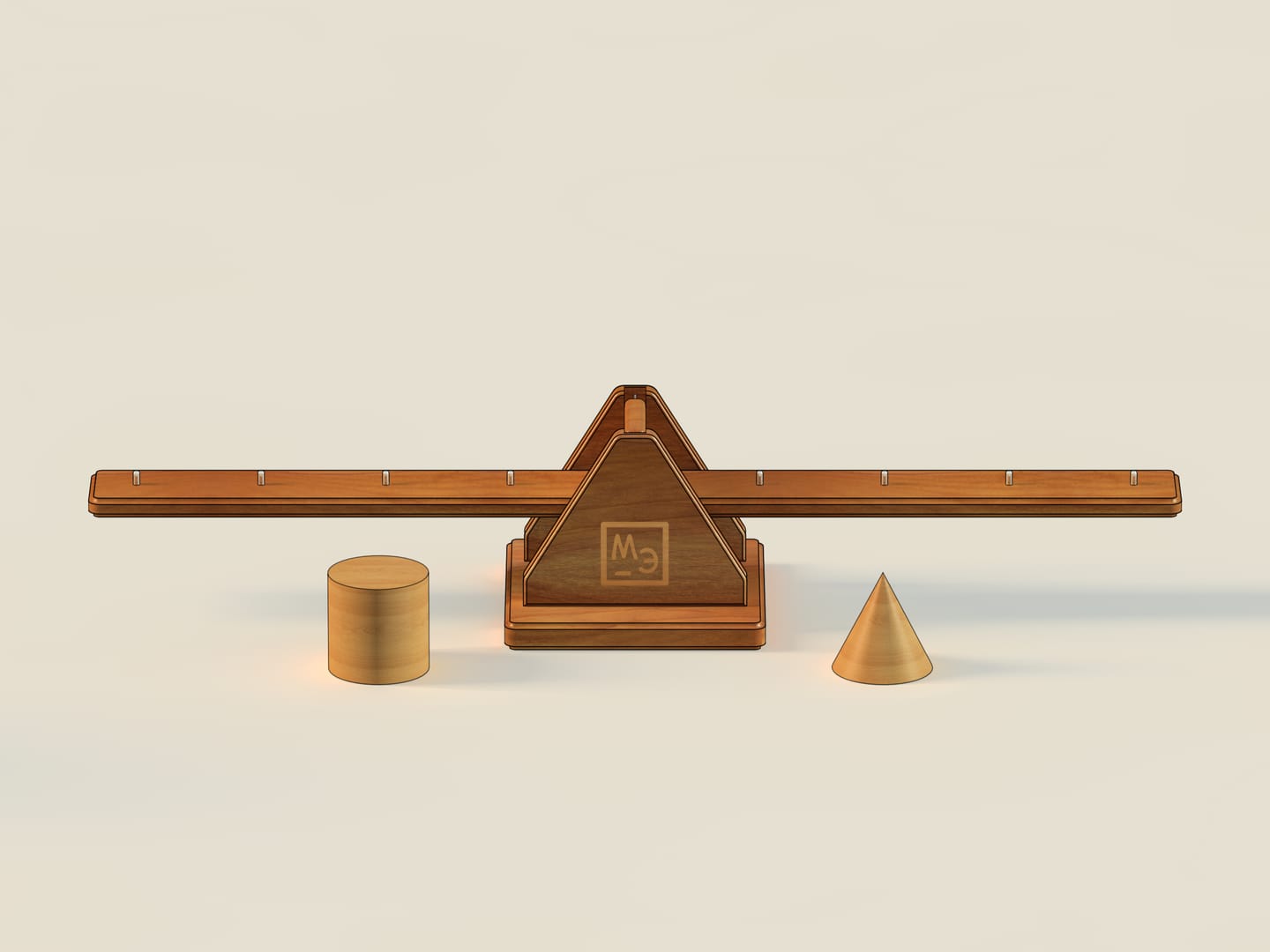
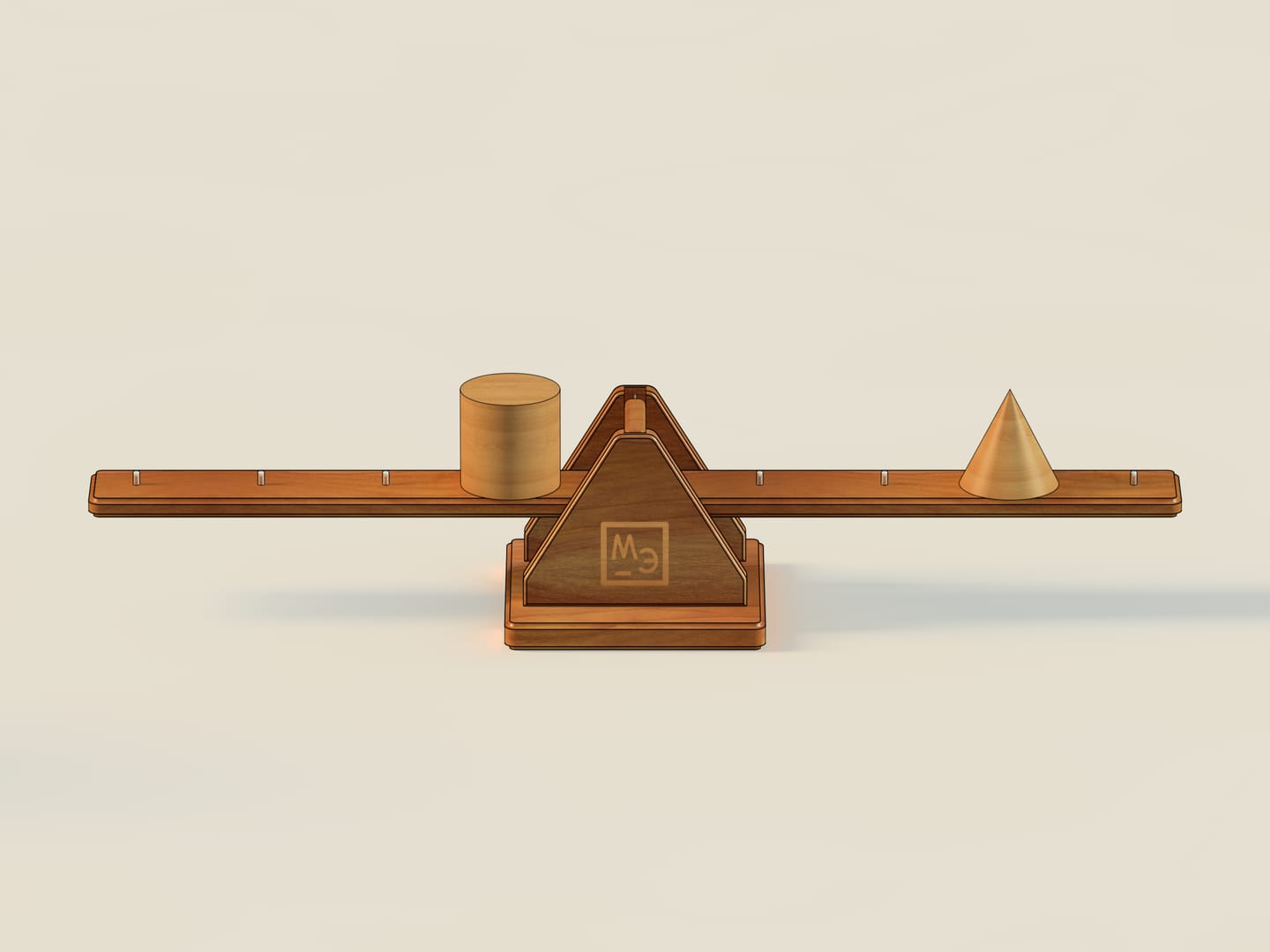
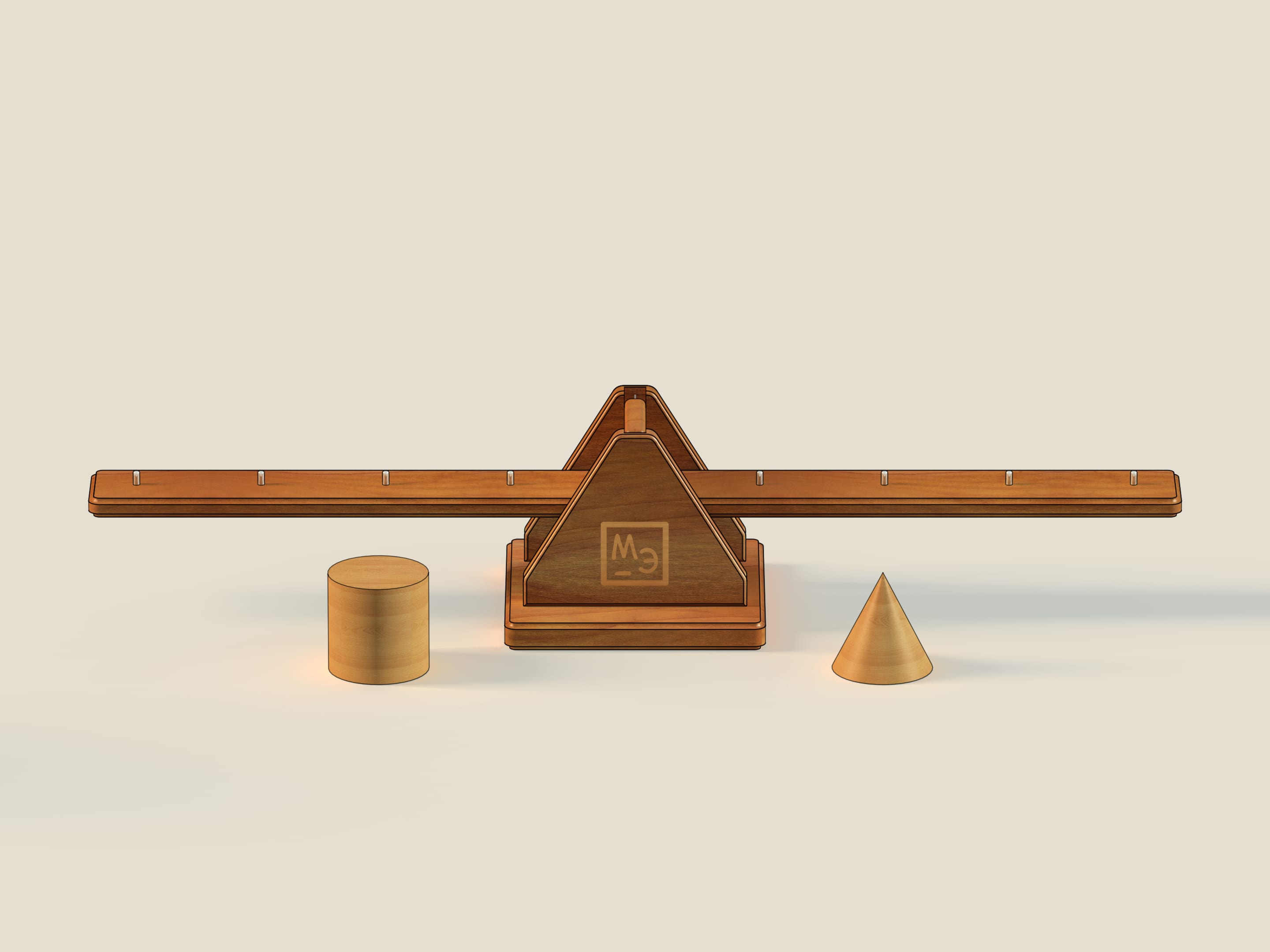
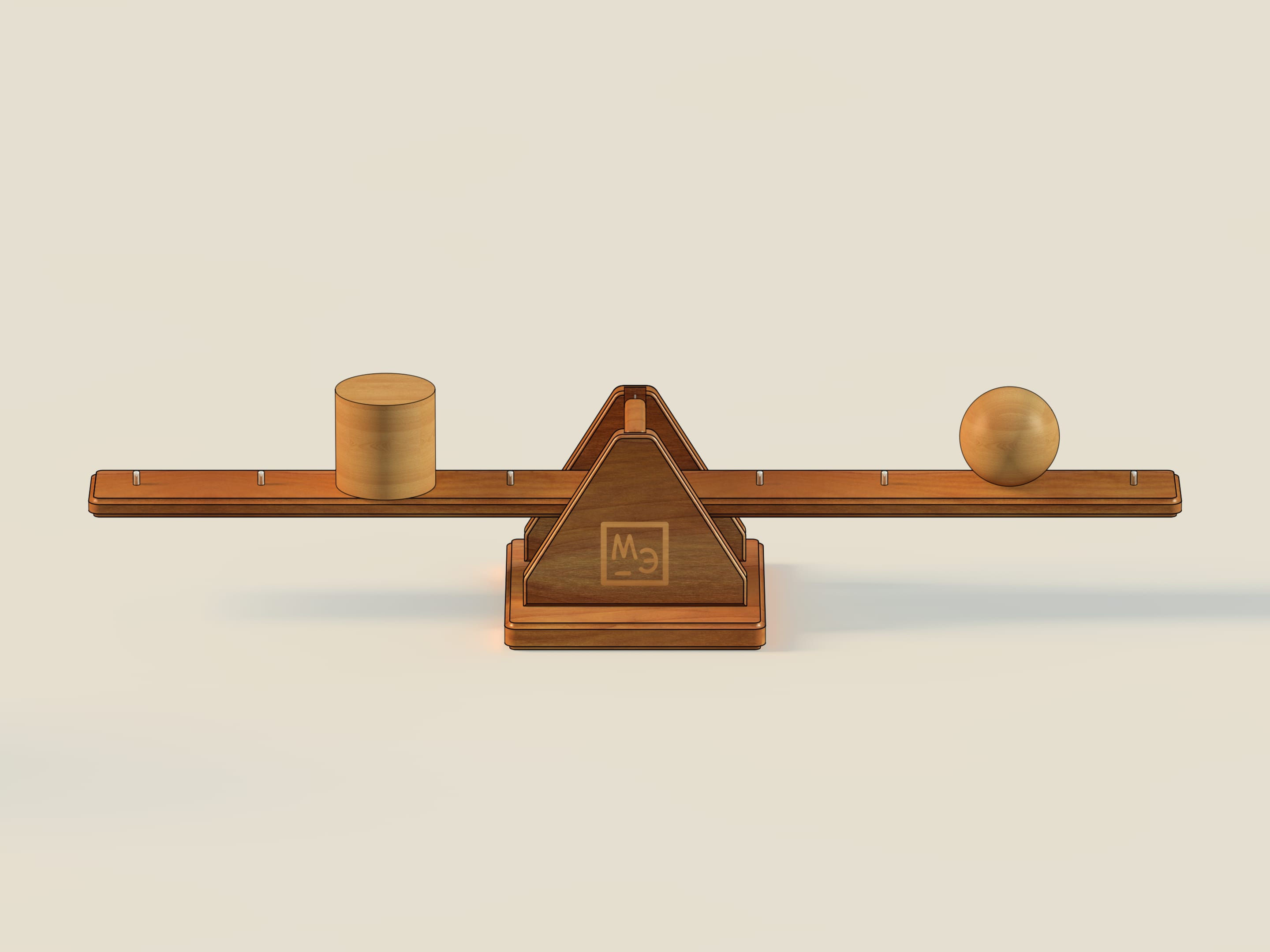
Lever scales enable you to calculate the ratio between the volumes of a pyramid and a prism, a cone and a cylinder, a sphere and a cylinder.
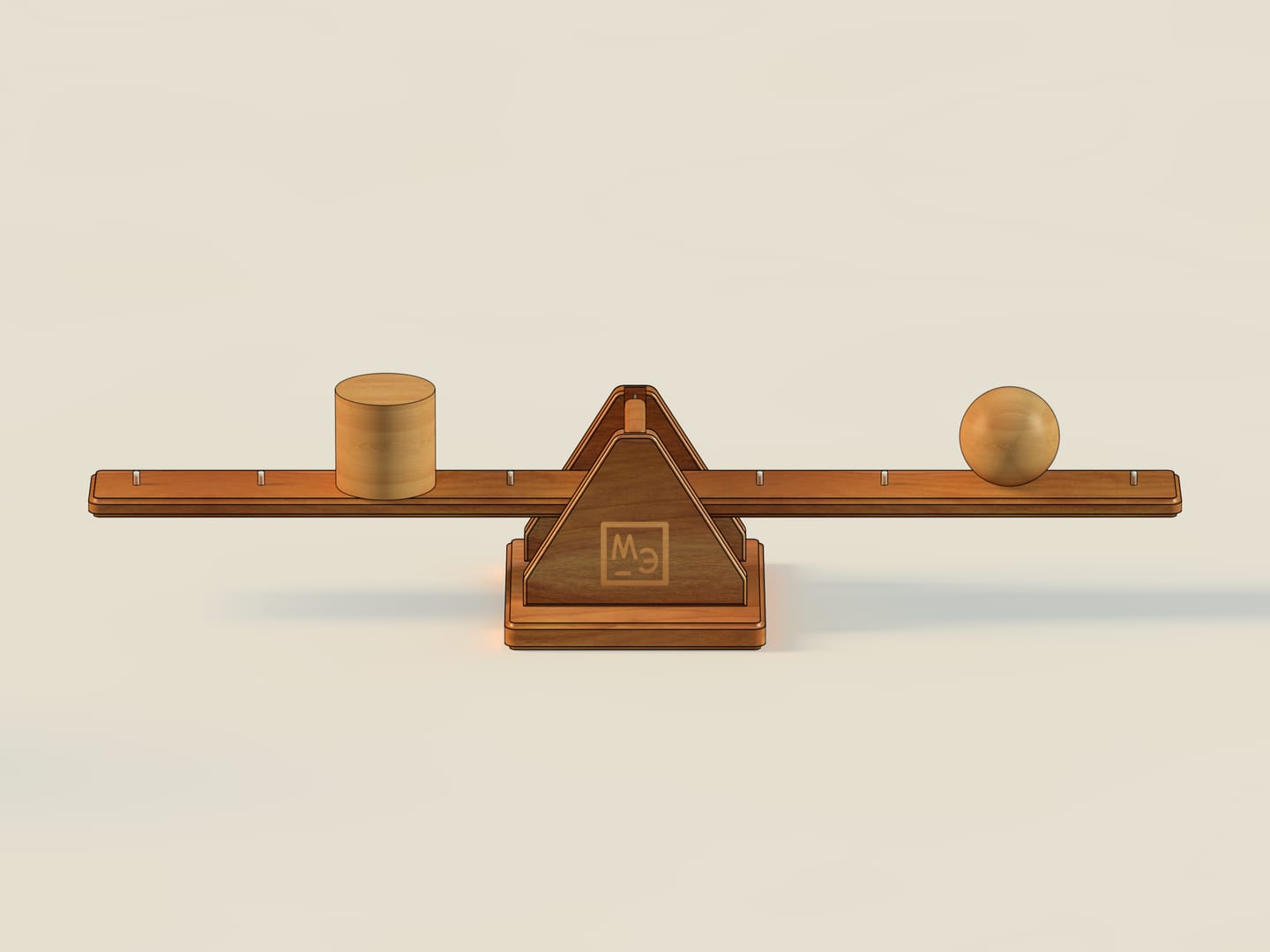
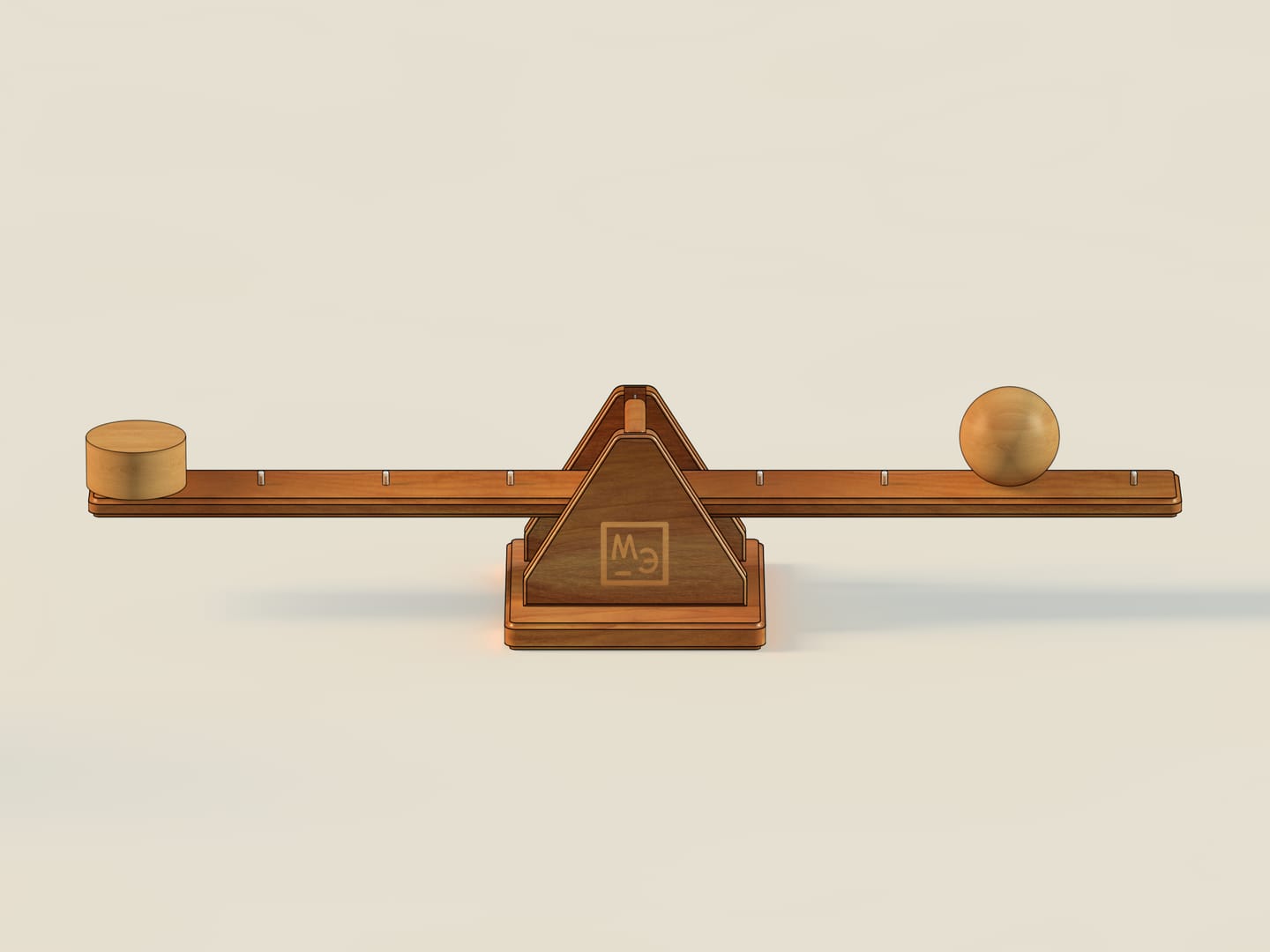
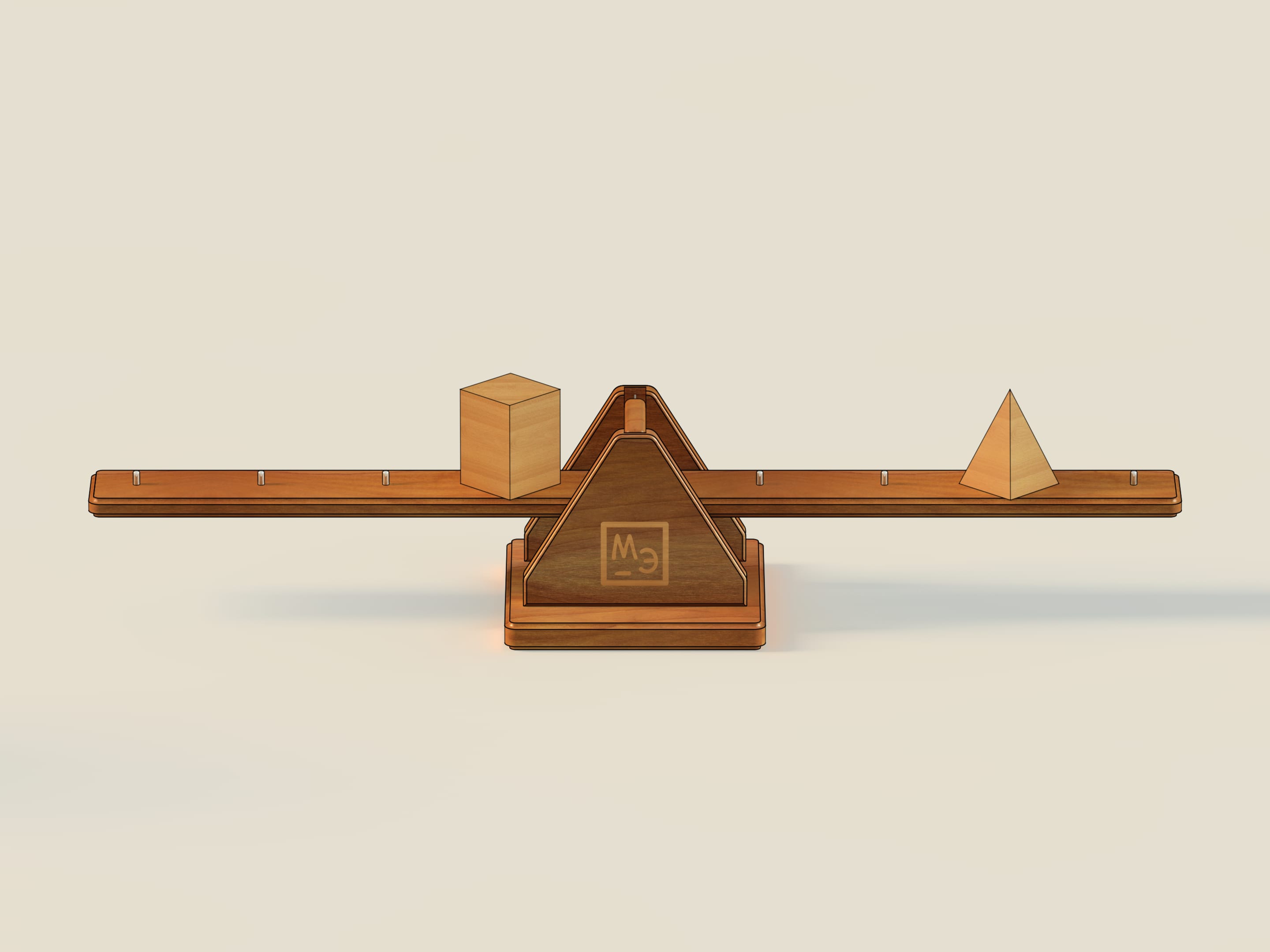
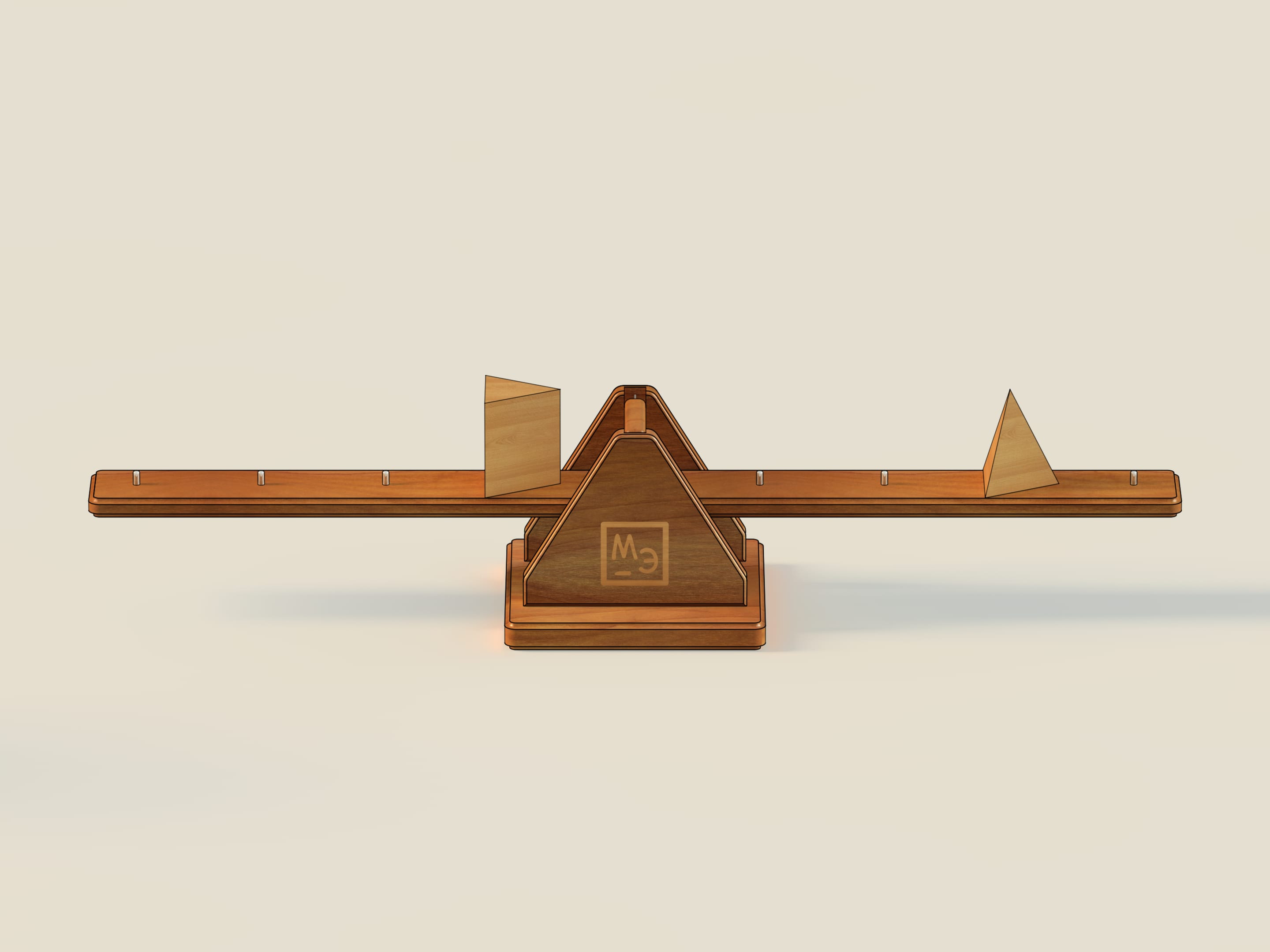
The key principle of seesaw scale is intuitively obvious: to balance a heavy body one needs to put the light body farther from the fulcrum. Quantitatively, this principle is expressed in the fact that in the equilibrium position the relationship between the masses (therefore, volumes, since the objects’ densities are the same) is equal to inverse relationship of the distances from the fulcrum to the bodies being weighed.
In the case given, the pyramid should be placed three times as far as the prism. The volume of the pyramid is one third of the volume of the prism with the equal base and height.
A cylinder and a cone with equal bases and equal heights can be represented as prism and pyramid limits respectively. So the volume of a cone is equal to one third of the volume of a cylinder with the same base and height.
A ball and a cylinder. The radius of the base of the cylinder is equal to the radius of the sphere and the height of the cylinder is equal to the diameter of the sphere. With these dimensions, a sphere can be inscribed into the cylinder.
How do the volumes of a cylinder and a ball relate: how must the bodies be placed on a lever scale to bring them to equilibrium?
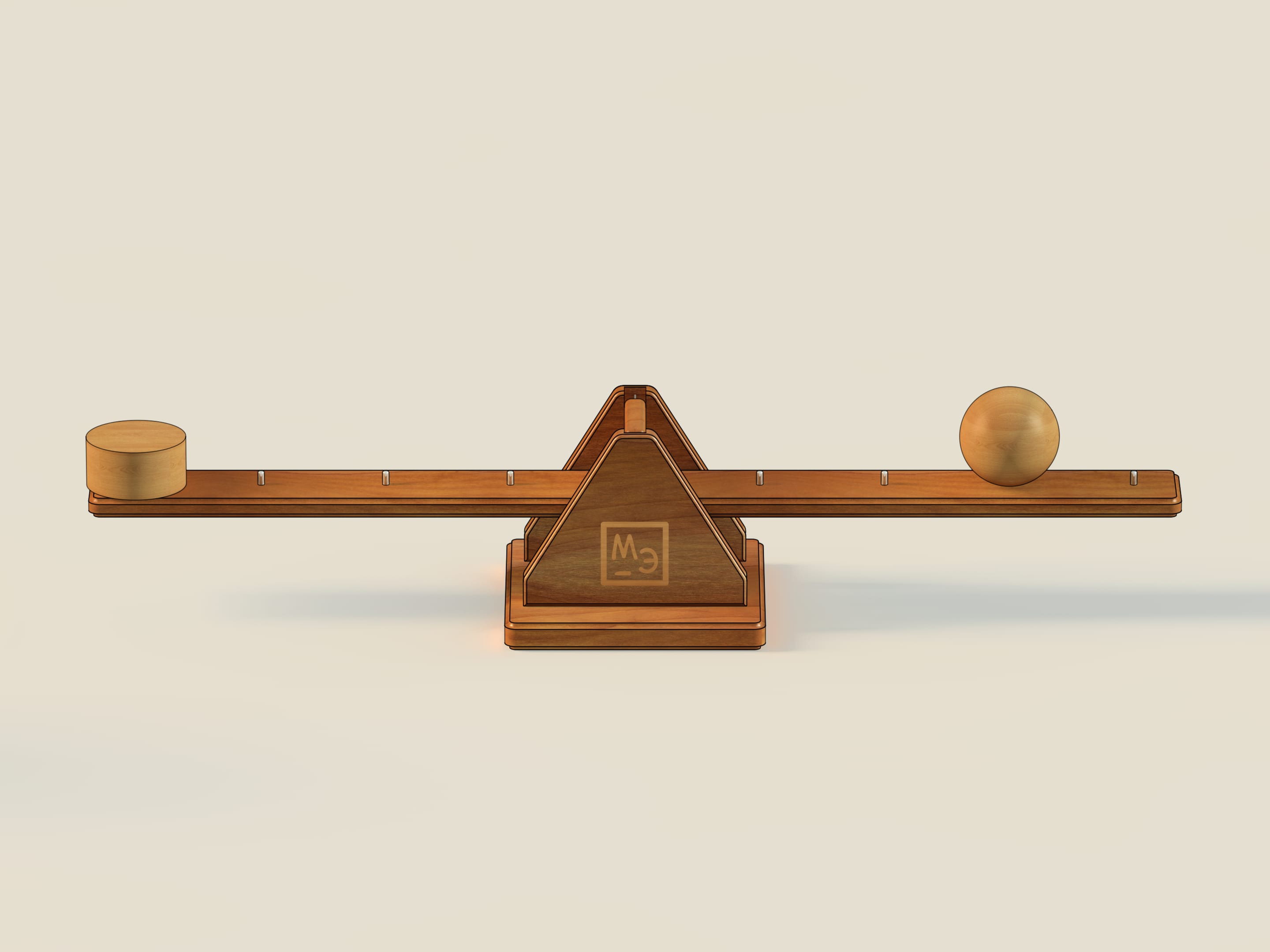
It can be verified that relationship of scale arms (arm is the distance from the fulcrum to the point where the weighted object is placed) in equilibrium will be $2:3$. Thus, the ball volume is equal to two thirds of the cylinder volume.
It is believed that out of his discoveries, Archimedes most appreciated the finding of the relationship between the volumes of the ball and the cylinder, thereby determining the ball volume.
The formula for a ball volume can be derived from this relationship.
Let's use a formula for a cylinder volume — product of the cylinder base area by its height. The base area is $\pi \cdot R^2$, cylinder height is $2 \cdot R$, where $R$ is the ball radius. Thus, the cylinder volume is $(\pi \cdot R^2) \cdot (2 \cdot R) = 2 \cdot \pi \cdot R^2$.
Multiplying by the coefficient of $2/3$, we get the formula for the ball volume — $4/3 \cdot \pi \cdot R^3$.
It is worth considering the possibility of dividing the cylinder into two parts equal in height. If we balance the ball with the cylinder having the height equal to the ball radius, the scale’s arms relation will be exactly $4:3$
When I was questor in Sicily I managed to track down his <Archimedes> grave. The Syracusians knew nothing about it, and indeed denied that any such thing existed. But there it was, completely surrounded and hidden by bushes of brambles and thorns.
I remembered having heard of some simple lines of verse which had been inscribed on his tomb, referring to a sphere and cylinder modelled in stone on top of the grave.
And so I took a good look round all the numerous tombs that stand beside the Agrigentine Gate. Finally I noted a little column just visible above the scrub: it was surmounted by a sphere and a cylinder. I immediately said to the Syracusans, some of whose leading citizens were with me at the time, that I believed this was the very object I had been looking for.
Cicero (106—43 BC), Tusculan Disputations, Book V, Sections 64—66.
Translation by Michael Grant in Cicero — On the Good Life, Penguin Books, New York, 1971, Pages 86—87.