Rainbow⁠

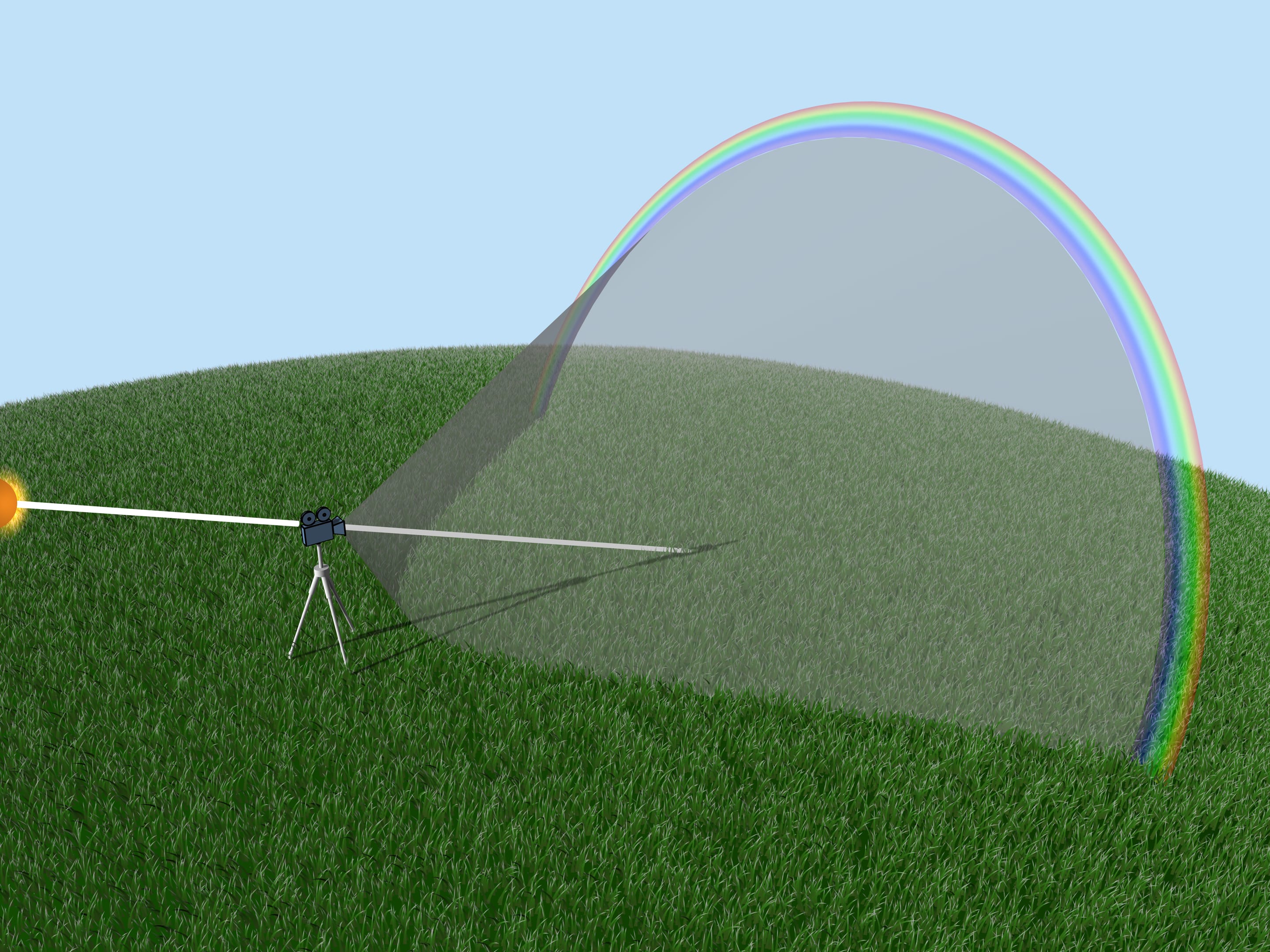
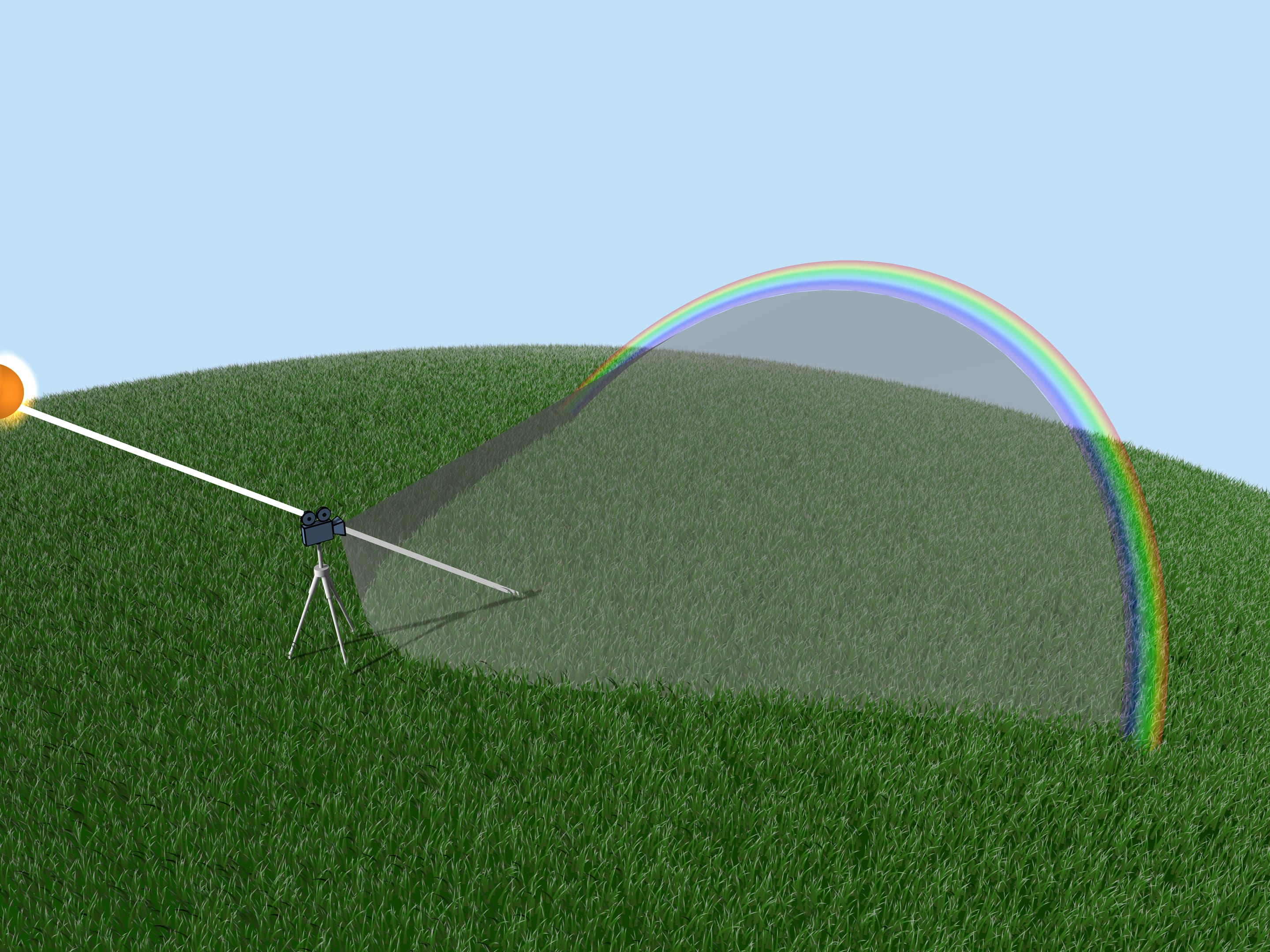
Sun. Rain. Rainbow.

Scien­tists of different times have tried to explain this natural phenom­enon. A complete theory of the rainbow goes beyond geometric and even wave optics and requires a powerful math­e­mat­ical appa­ratus. The film gives the first insight into the rainbow, which is never­the­less remark­able and by no means simple. This insight is based on the work of René Descartes and Isaac Newton.

René Descartes had explained the geom­etry of the rainbow: its shape and loca­tion in the sky. Isaac Newton “col­ored” the rainbow by explaining its colors.

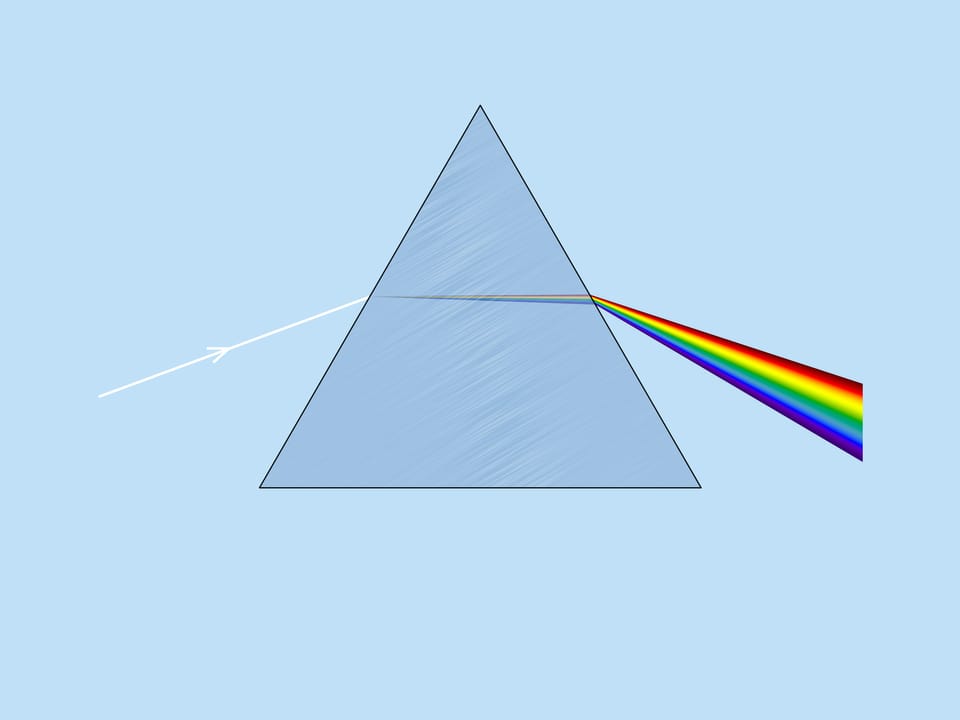
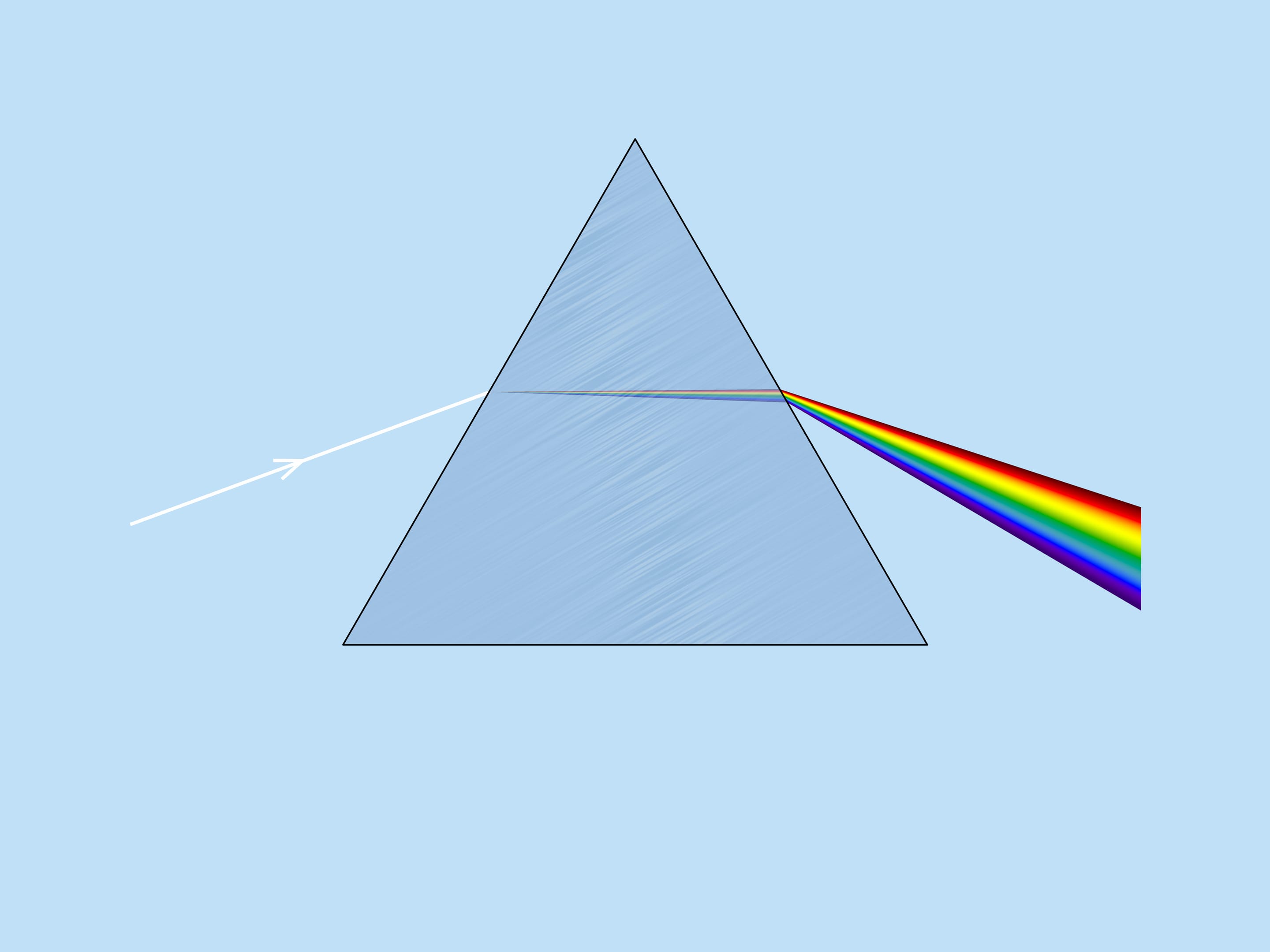
The great Isaac Newton, in his famous exper­i­ment with the glass prism, which is now essen­tial to physics lessons, broke down the white sunlight into its color compo­nents and showed that the different colors corre­spond to different refrac­tive indices. This effect is called light disper­sion. And it is because of the disper­sion the rainbow is multi­col­ored.

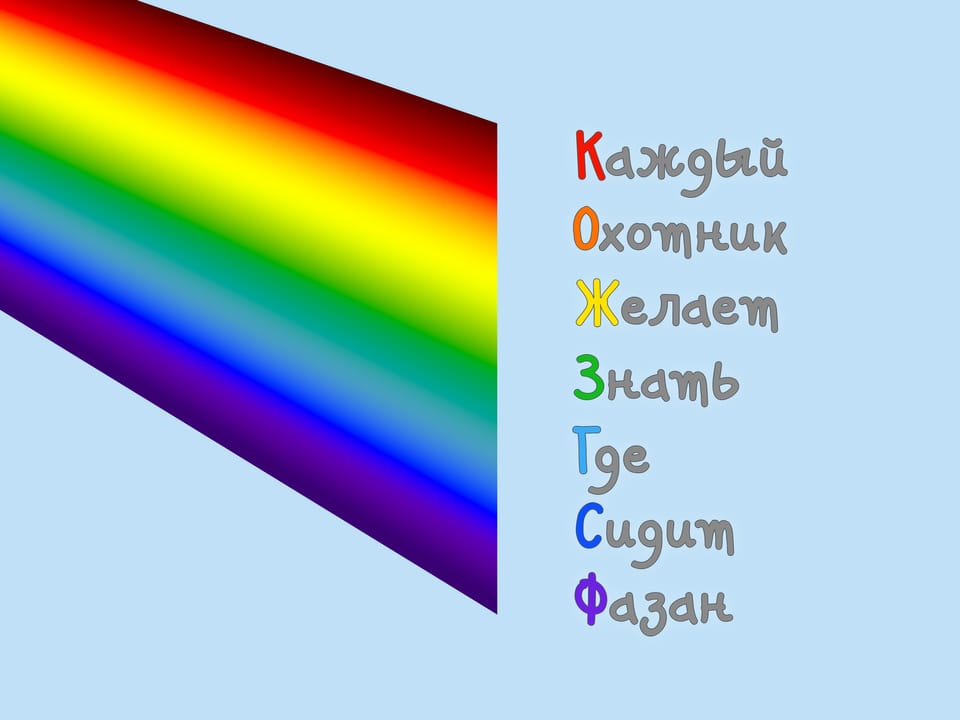
He distin­guished seven compo­nents in the resulting spec­trum: red, orange, yellow, green, blue, indigo, and violet. These colors are easy to remember with the well-known mnemonic rule, “Richard Of York Gave Battle In Vain (ROYGBIV)”. Inter­est­ingly, not all coun­tries have seven colors of the rainbow. In Japan, for example, there are six.

Rain­bows are formed by rain­drops in the air. To under­stand exactly how it happens, consider the path of the solar rays in a single droplet of water. Let’s assume that the droplet is shaped like a ball. (René Descartes conducted thou­sands of exper­i­ments with a glass spher­ical flask filled with water.) Because of symmetry, the path of a ray in a droplet depends only on its distance from the center of the drop. Consider the rays that lie in the plane “the Sun — observer’s eye — center of the drop”.

Since the Sun is located very far from the Earth, we can assume that its rays reach the Earth’s atmos­phere parallel to each other, and the angle of their inci­dence depends only on the height of the Sun above the horizon.

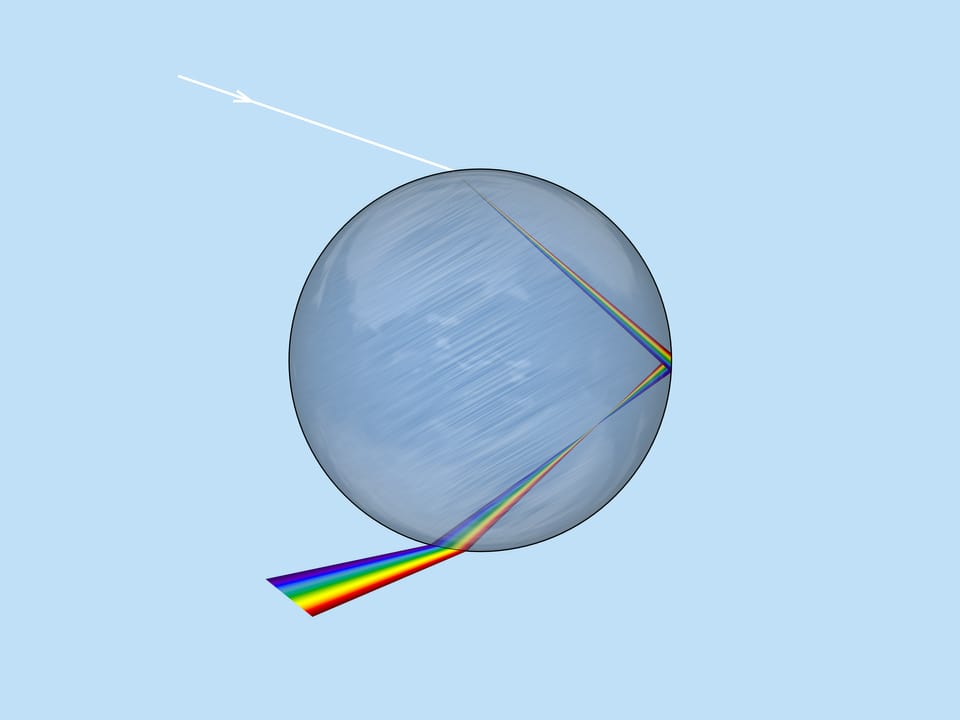
The solar rays forming the rainbow enter the droplet from the air and then leave outside, expe­ri­encing a series of refrac­tions and reflec­tions. At the border of two media, in this case water and air, both refrac­tion and reflec­tion always occur. We will only consider such a path of the rays which is rele­vant to the rainbow forma­tion.

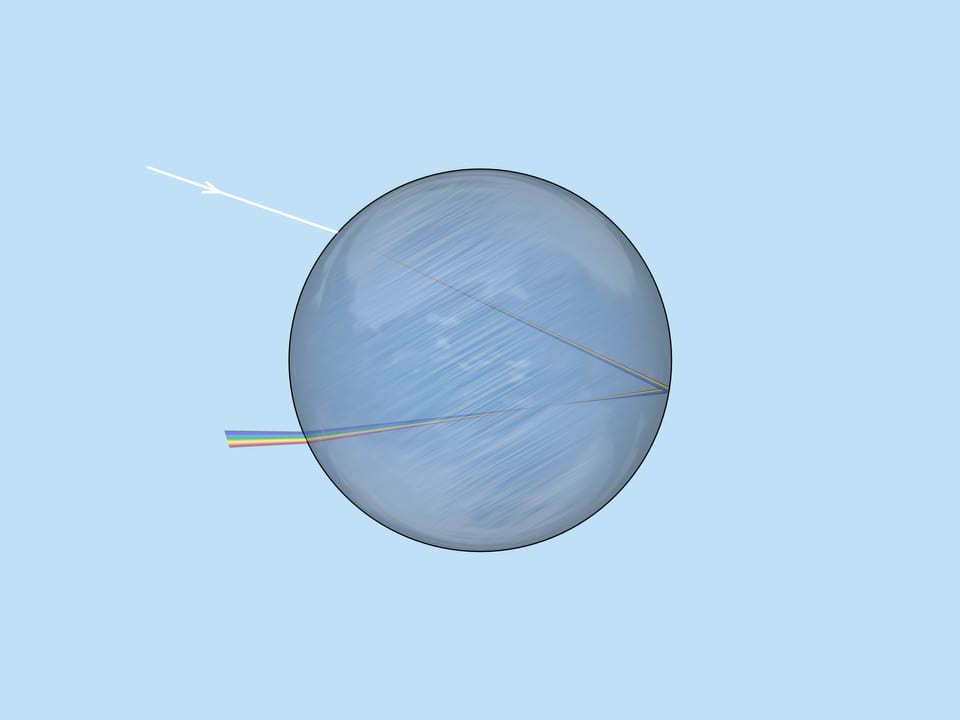
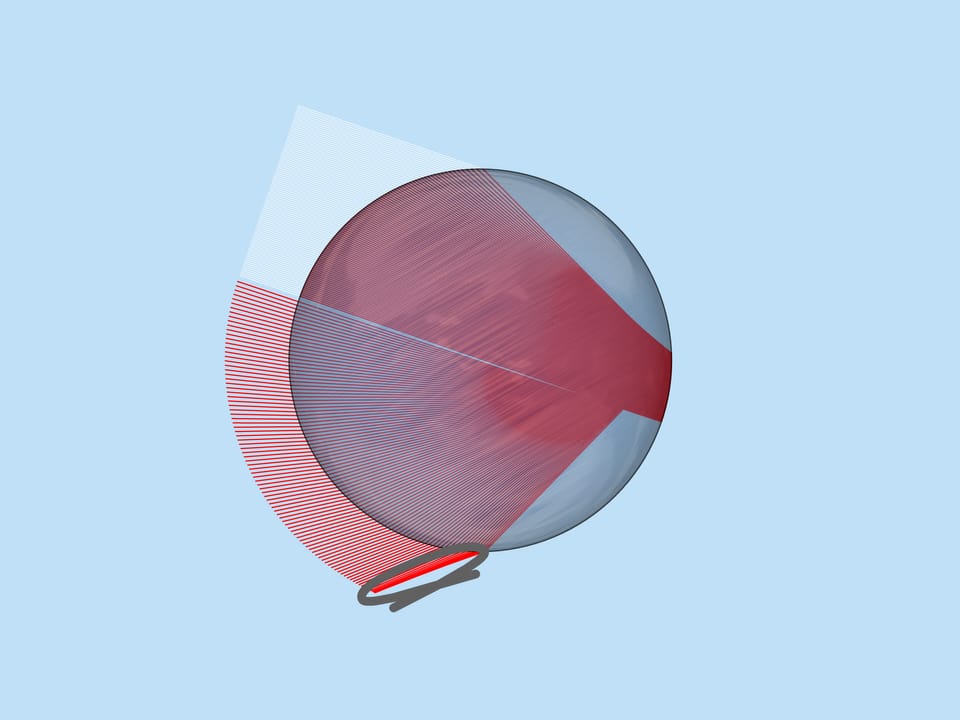
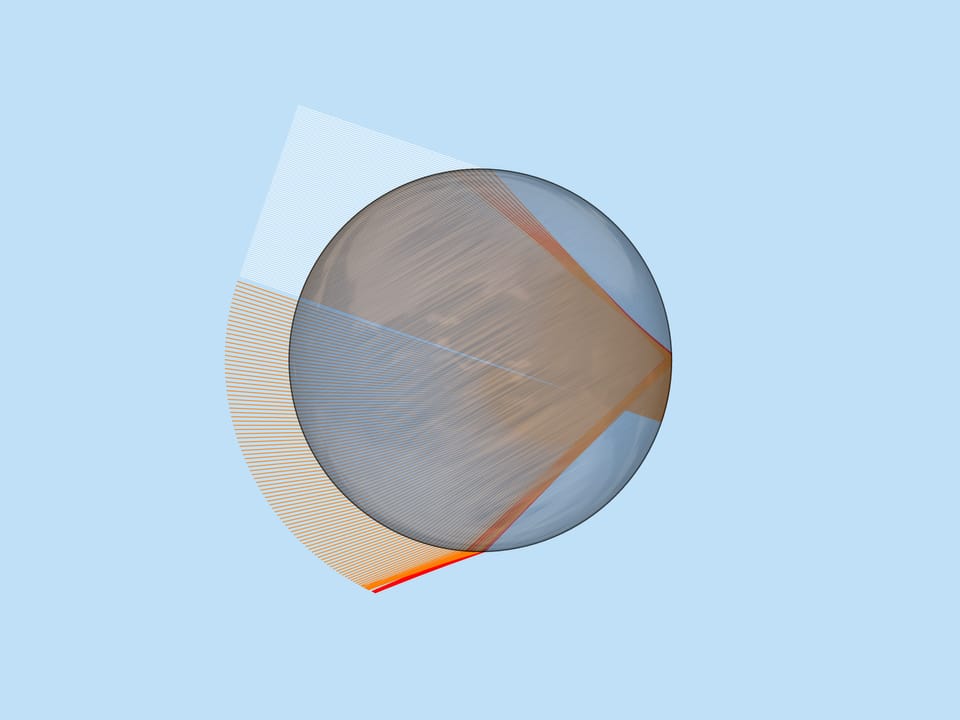
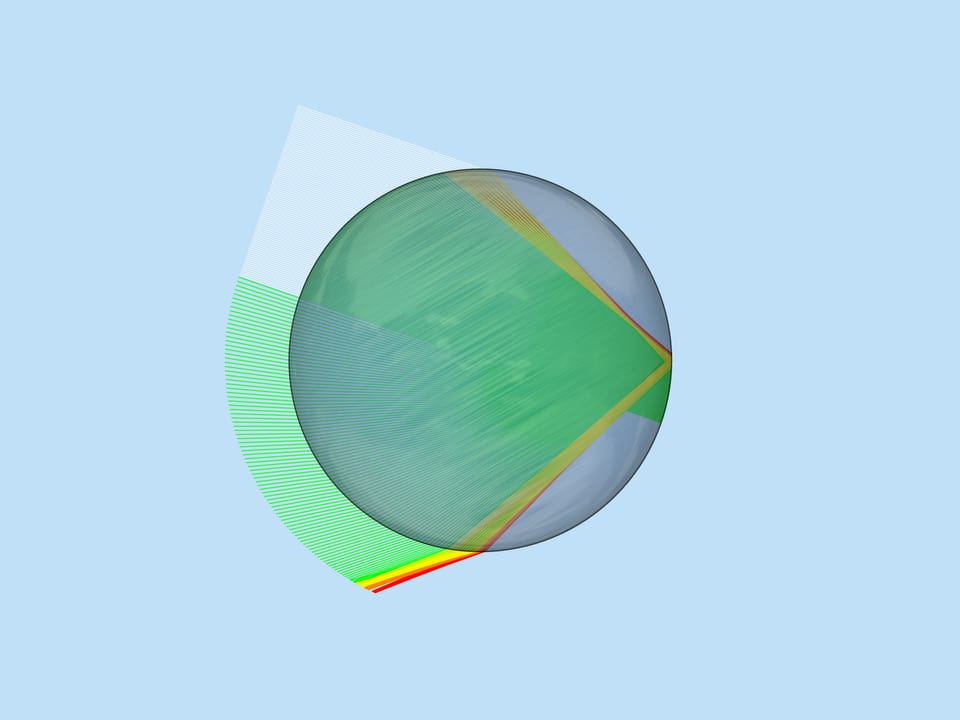
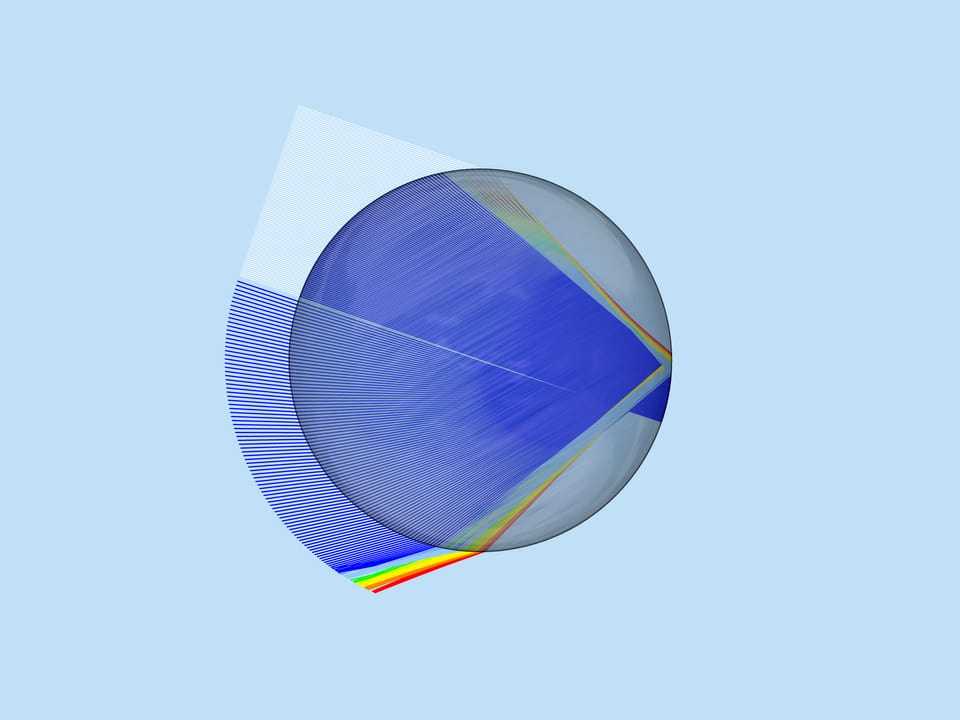
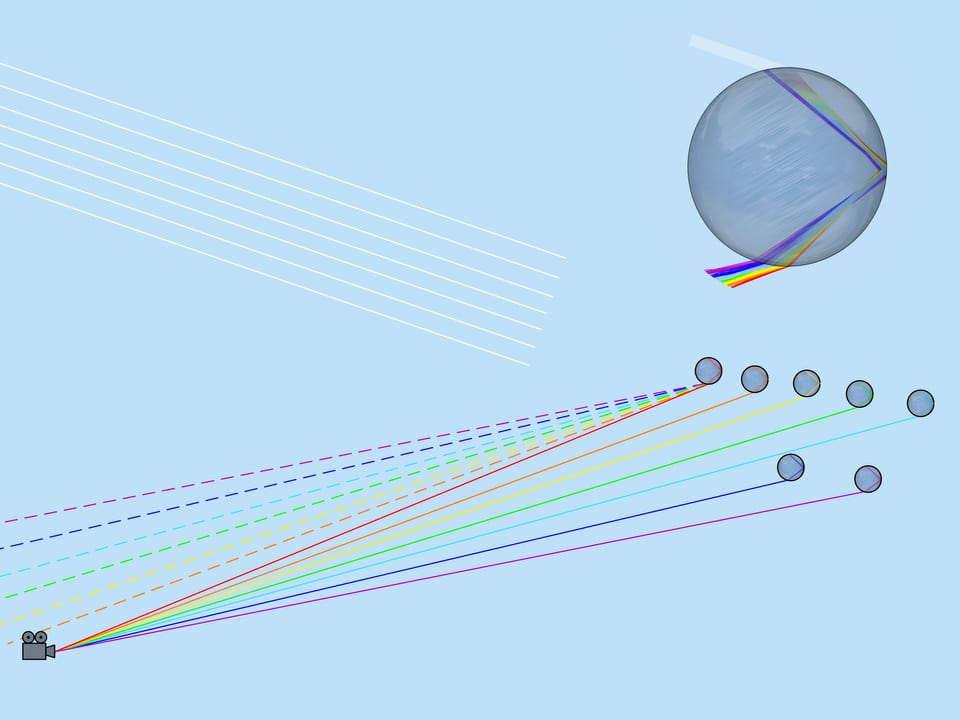
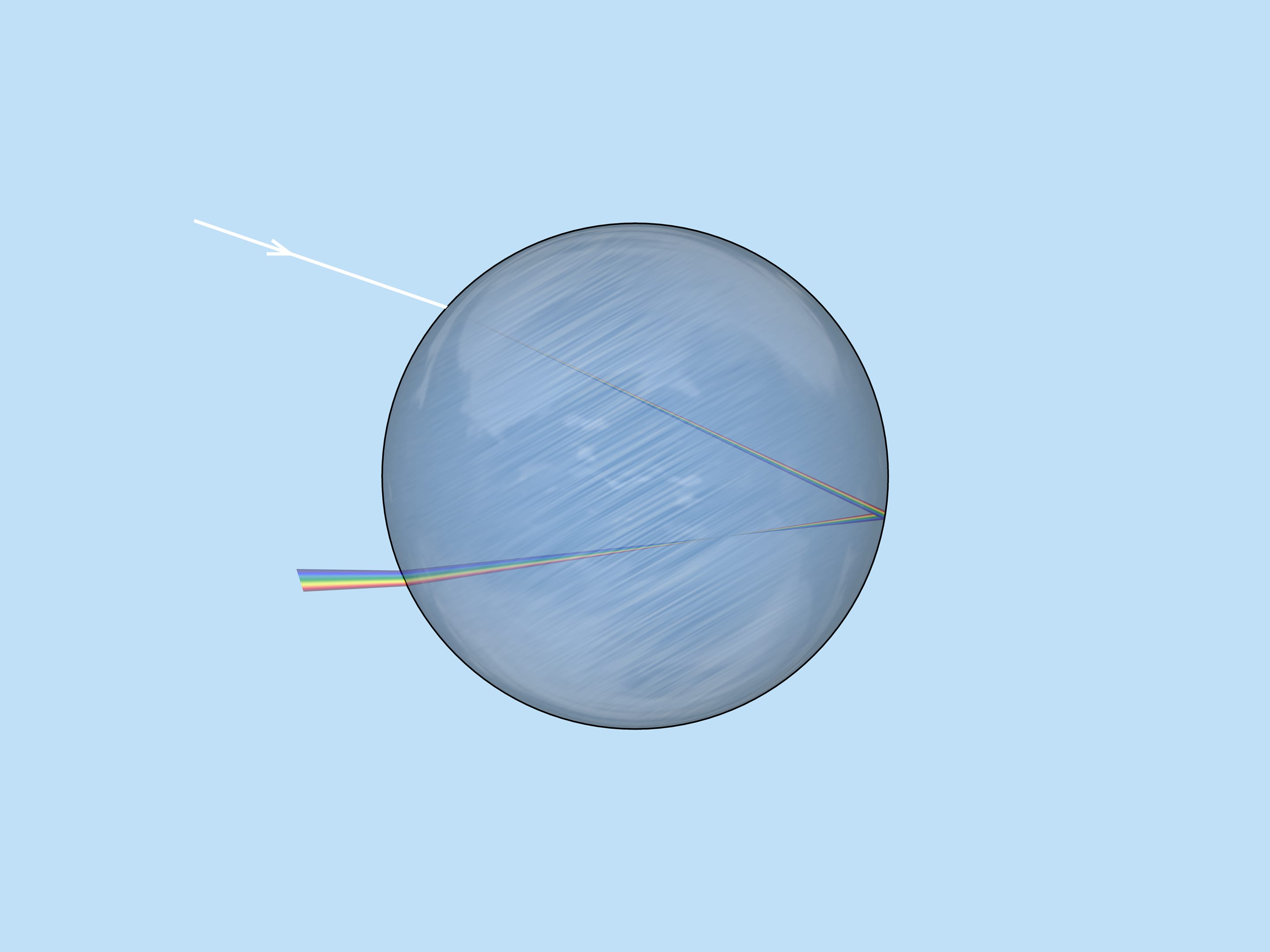
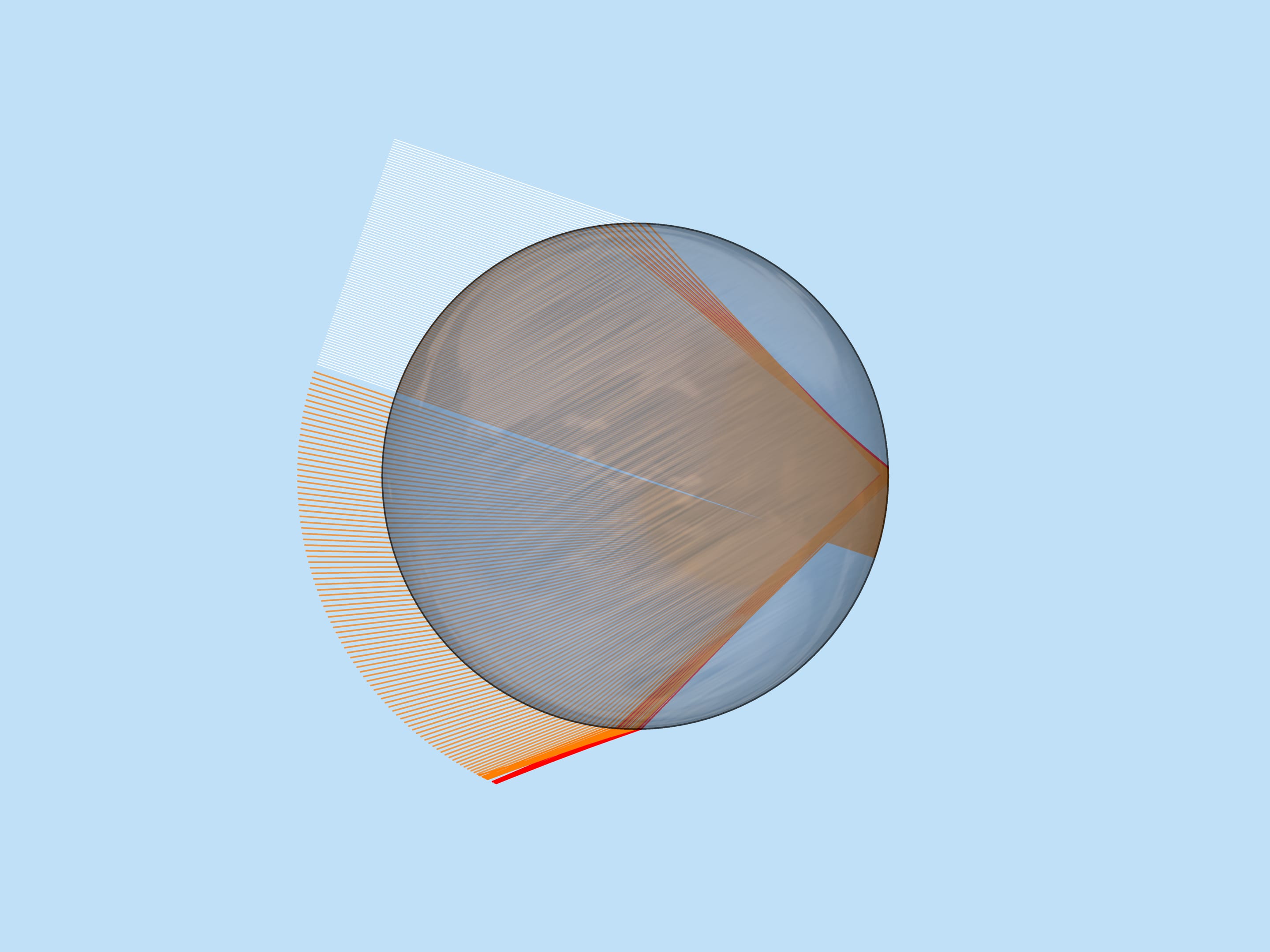
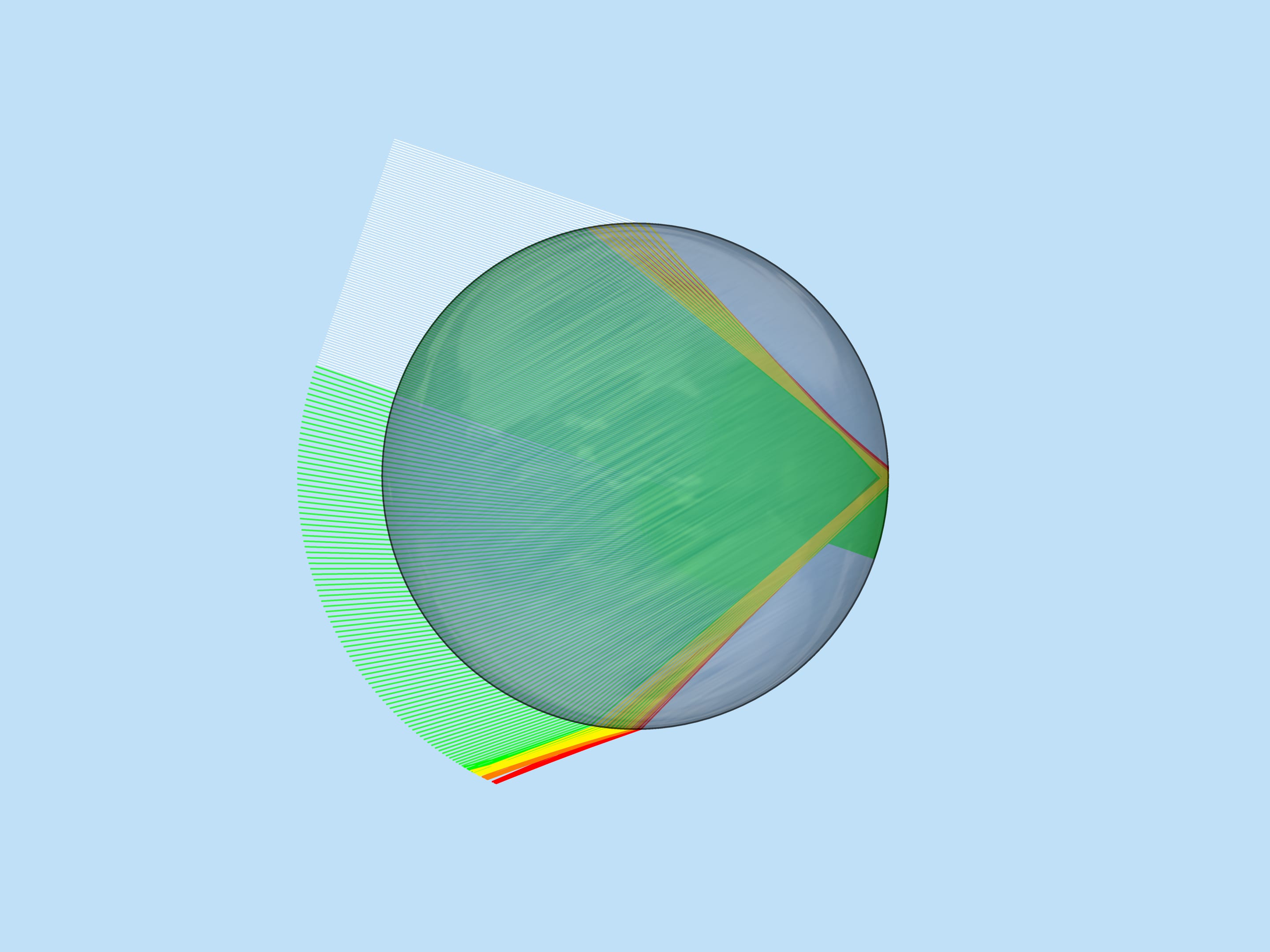
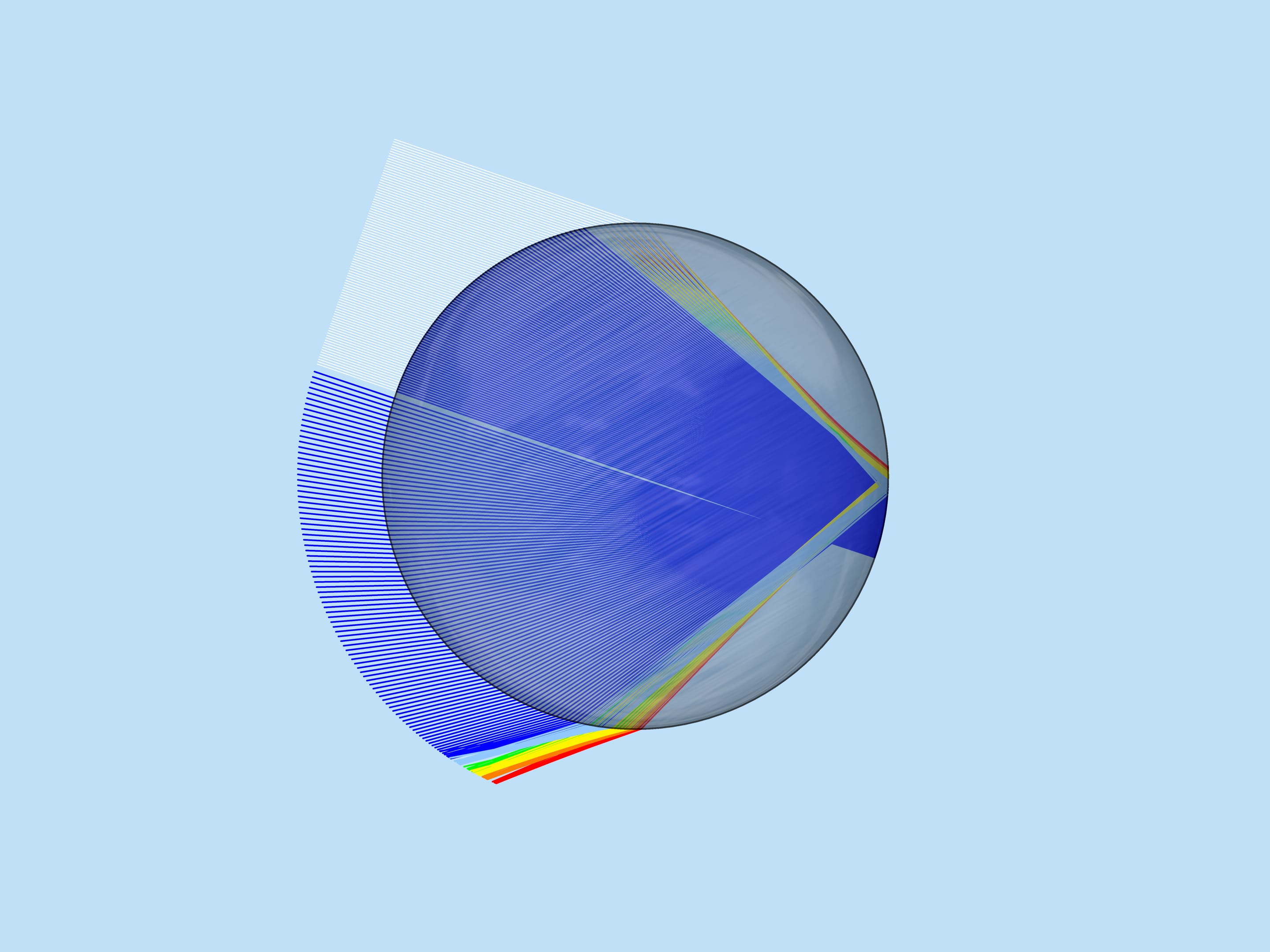
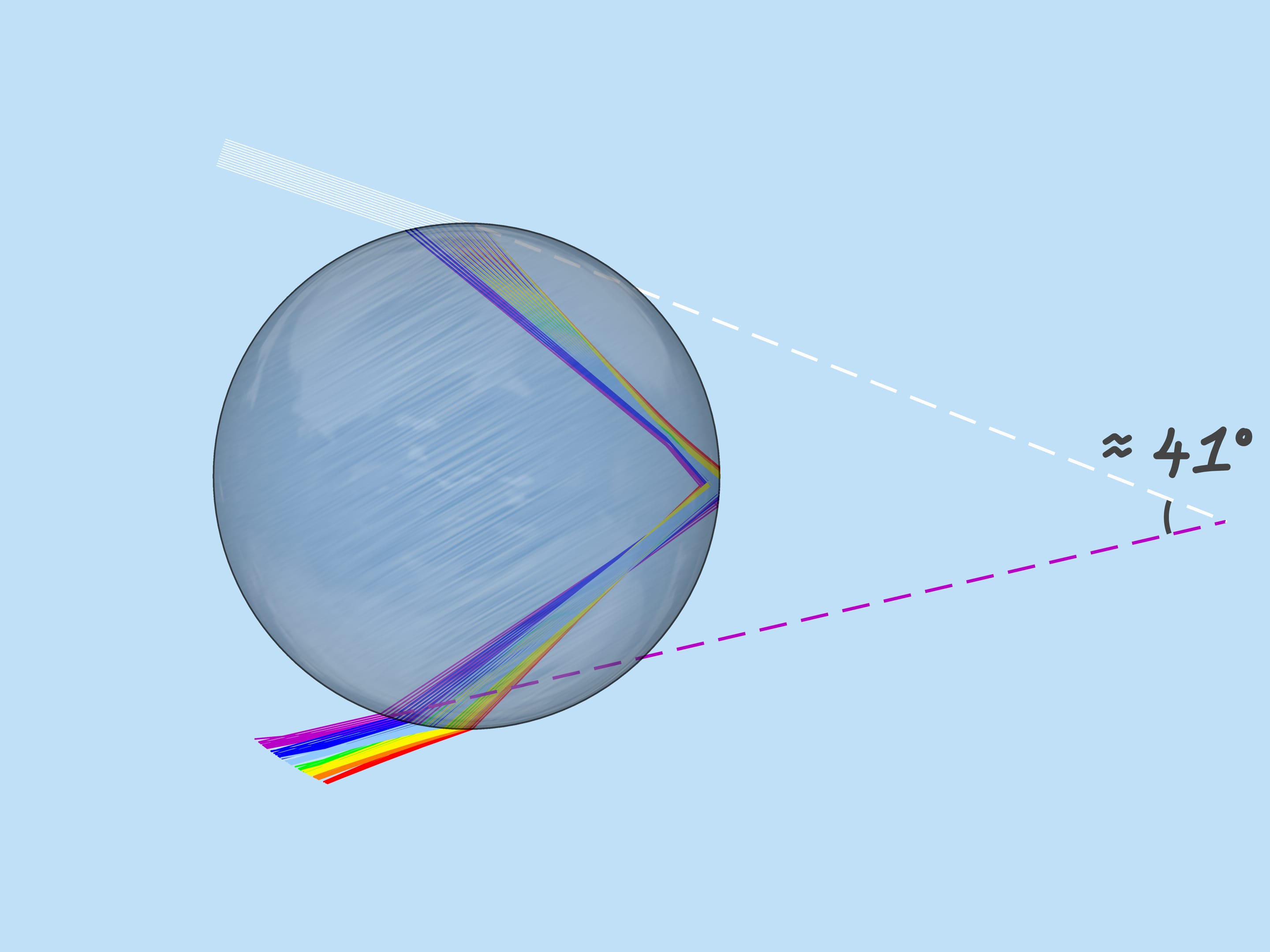
First, consider those rays that hit the upper half of the droplet. These rays are refracted on entering the droplet, then reflected from the back side of the droplet and, refracted again, go outside the droplet. As they refract, disper­sion occurs and colors appear.

The rays fall uniformly on the surface of the droplet facing the Sun. On leaving the droplet, the rays are distrib­uted nonuni­formly. Let’s see which rays form a rainbow.

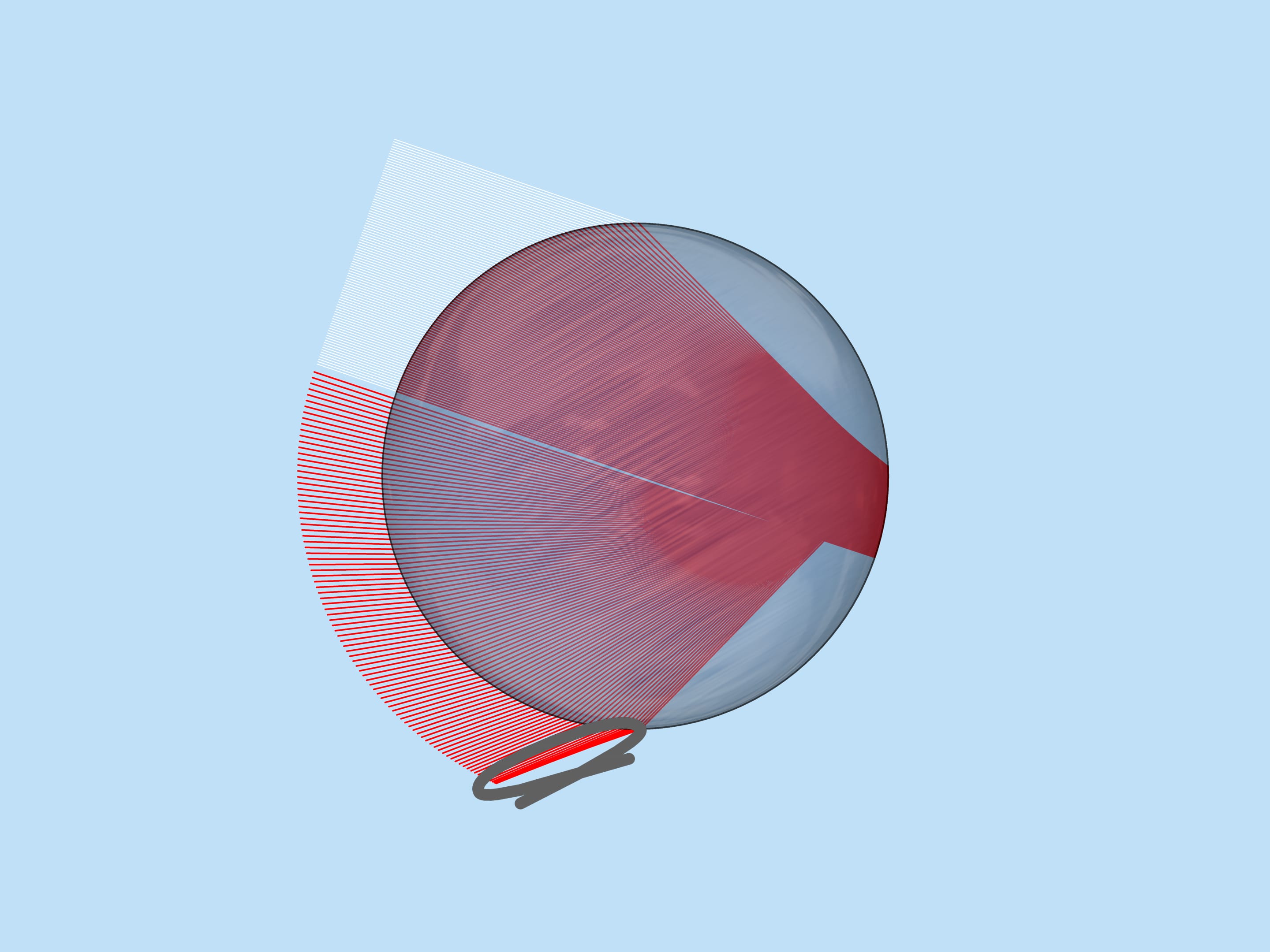
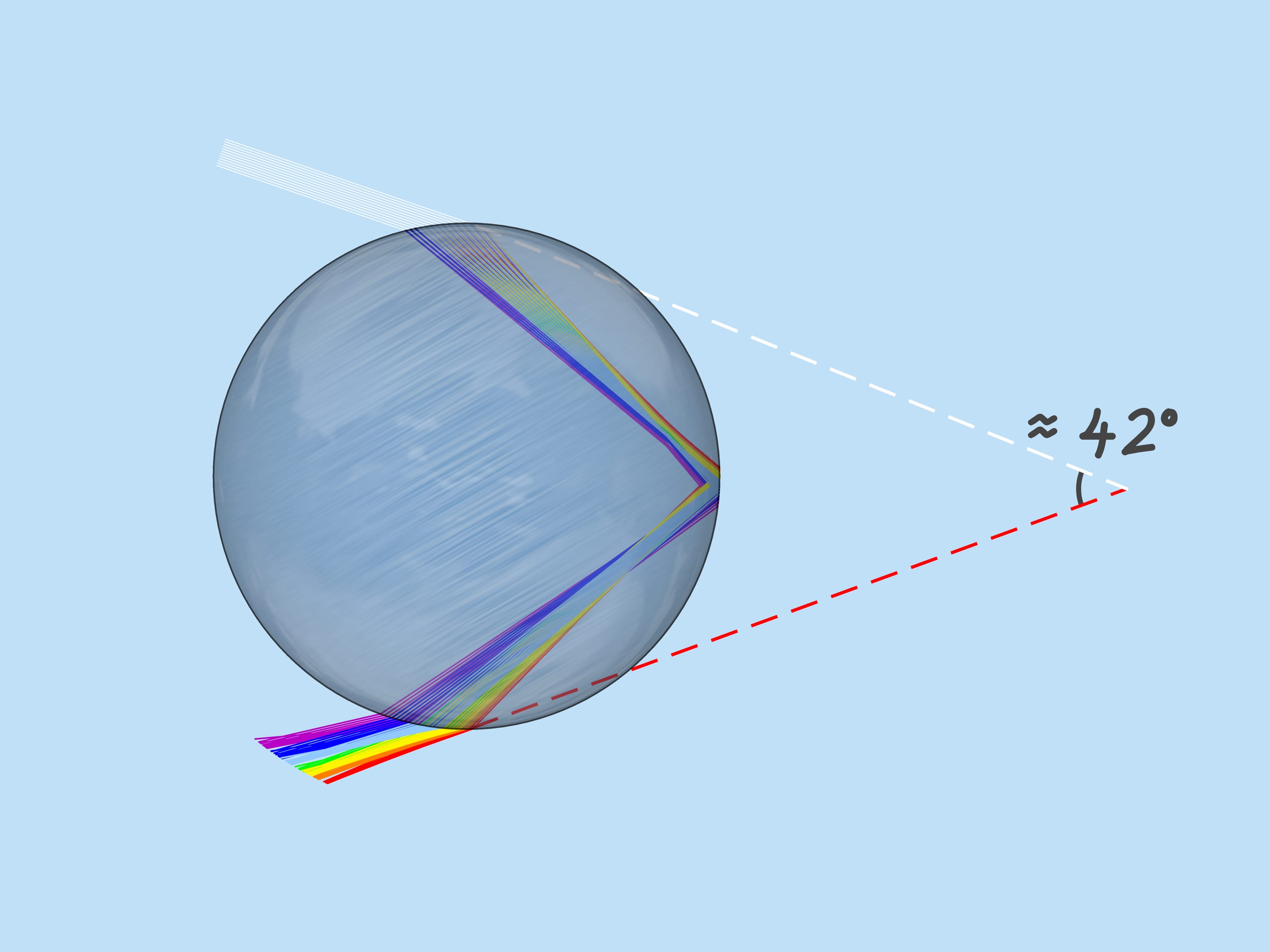
The ray that hits the droplet exactly in the center is not refracted (because the angle of inci­dence is $0^{\circ}$). Reflecting off the back side of the droplet, it will leave toward the inci­dent ray. Rays that hit the droplet close to its center are not refracted much (because their angle of inci­dence is close to $0^{\circ}$). After reflec­tion, these rays emerge almost towards the inci­dent rays, deflected from the return in the oppo­site direc­tion by a small angle. As the incoming rays move away from the center of the droplet, this angle increases, but at some point a maximum is reached: the rays distant from the center by about $0{,}86$ of the droplet radius are deflected the most. Further away from the center of the droplet, as far as those rays just touching the droplet, the angle decreases.

Near the maximum (or, as math­e­mati­cians say, extreme) value, the deflec­tion angle changes slowly, so there is an “accu­mu­la­tion” of outgoing rays. These rays are what are perceived as rain­bows.

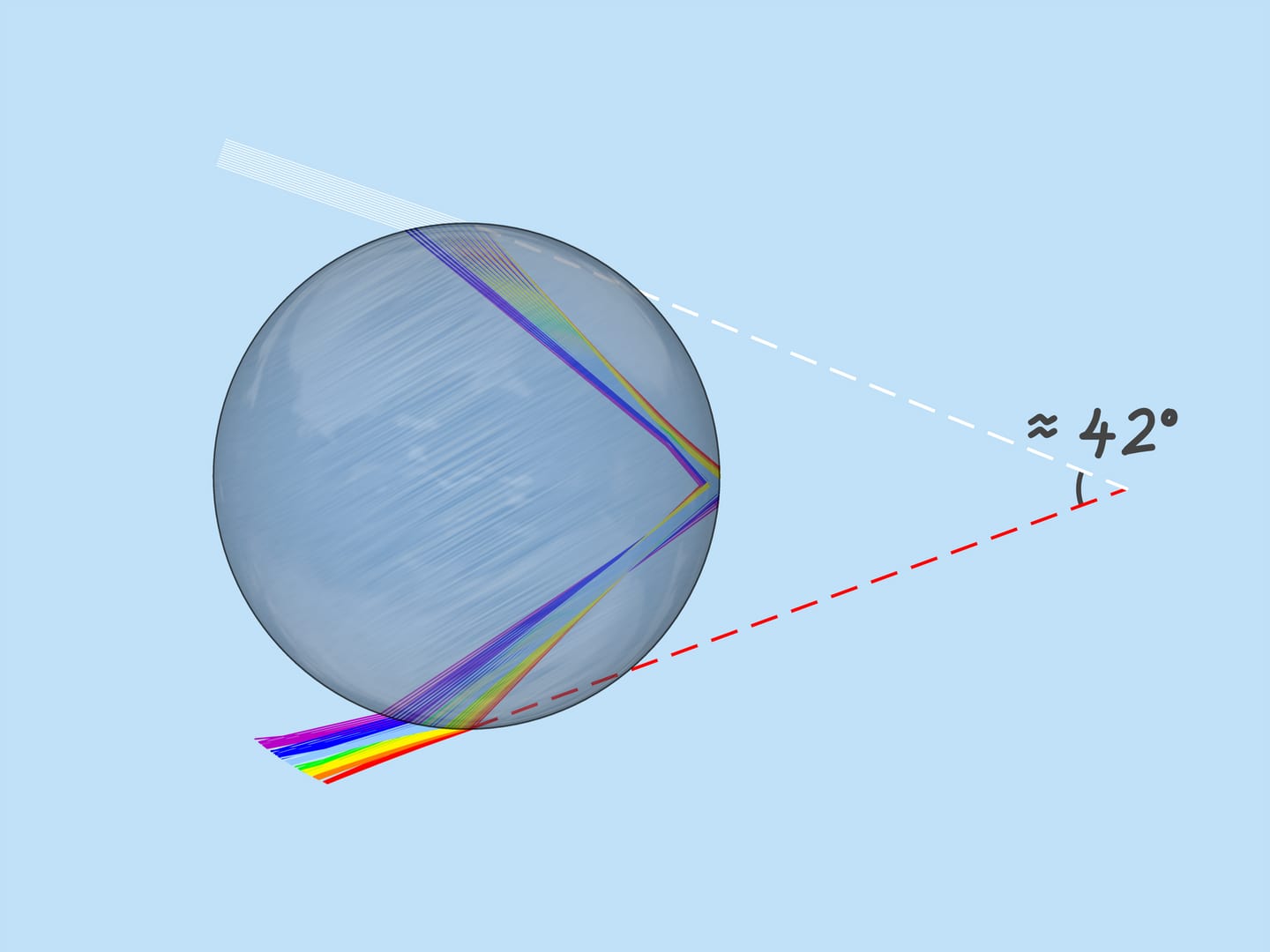
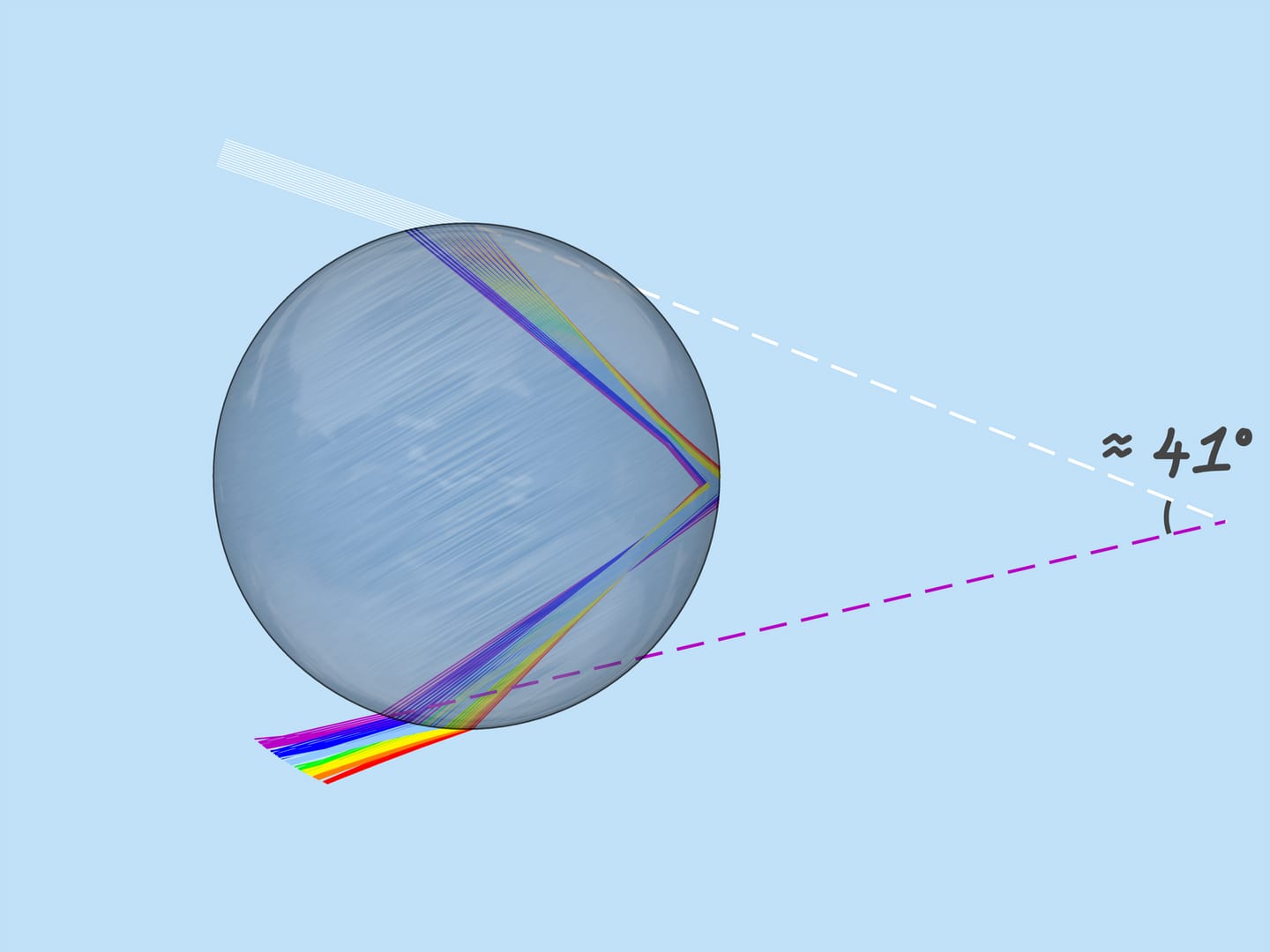
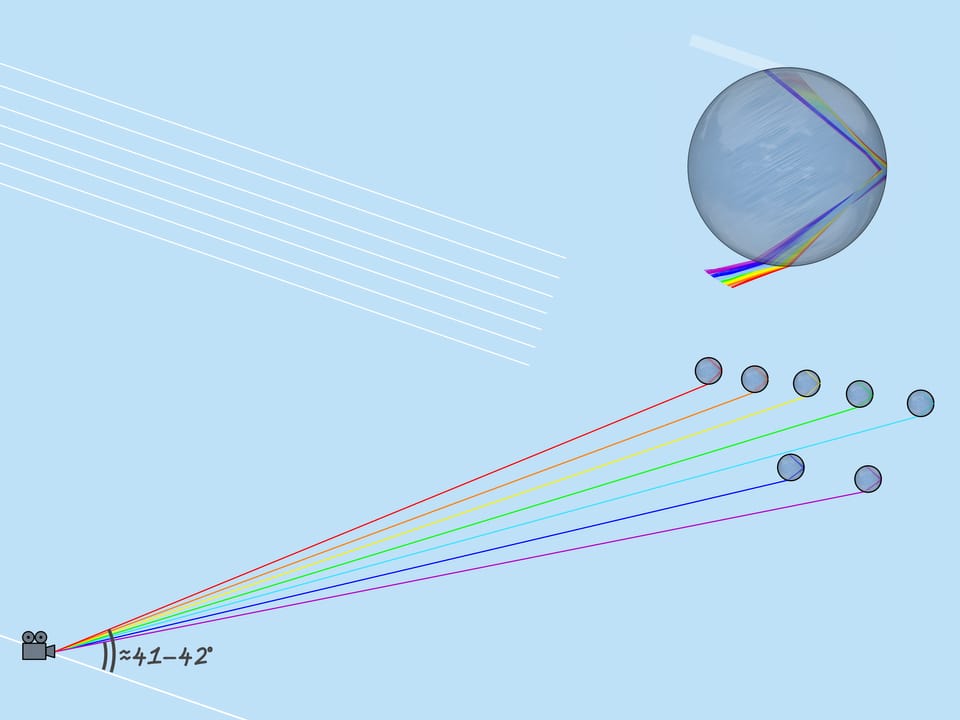
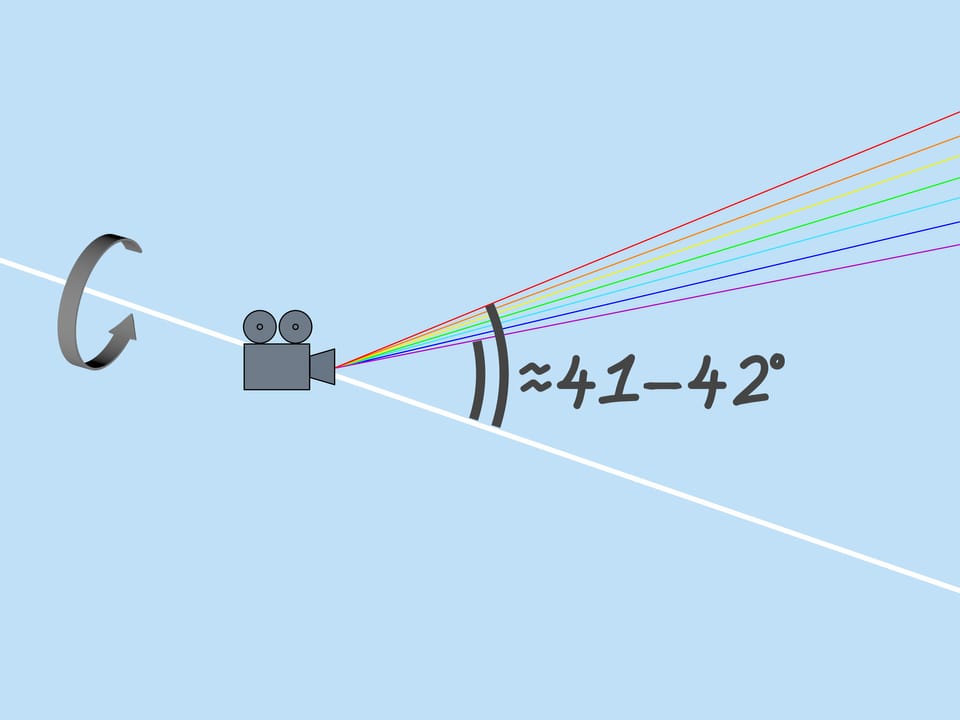
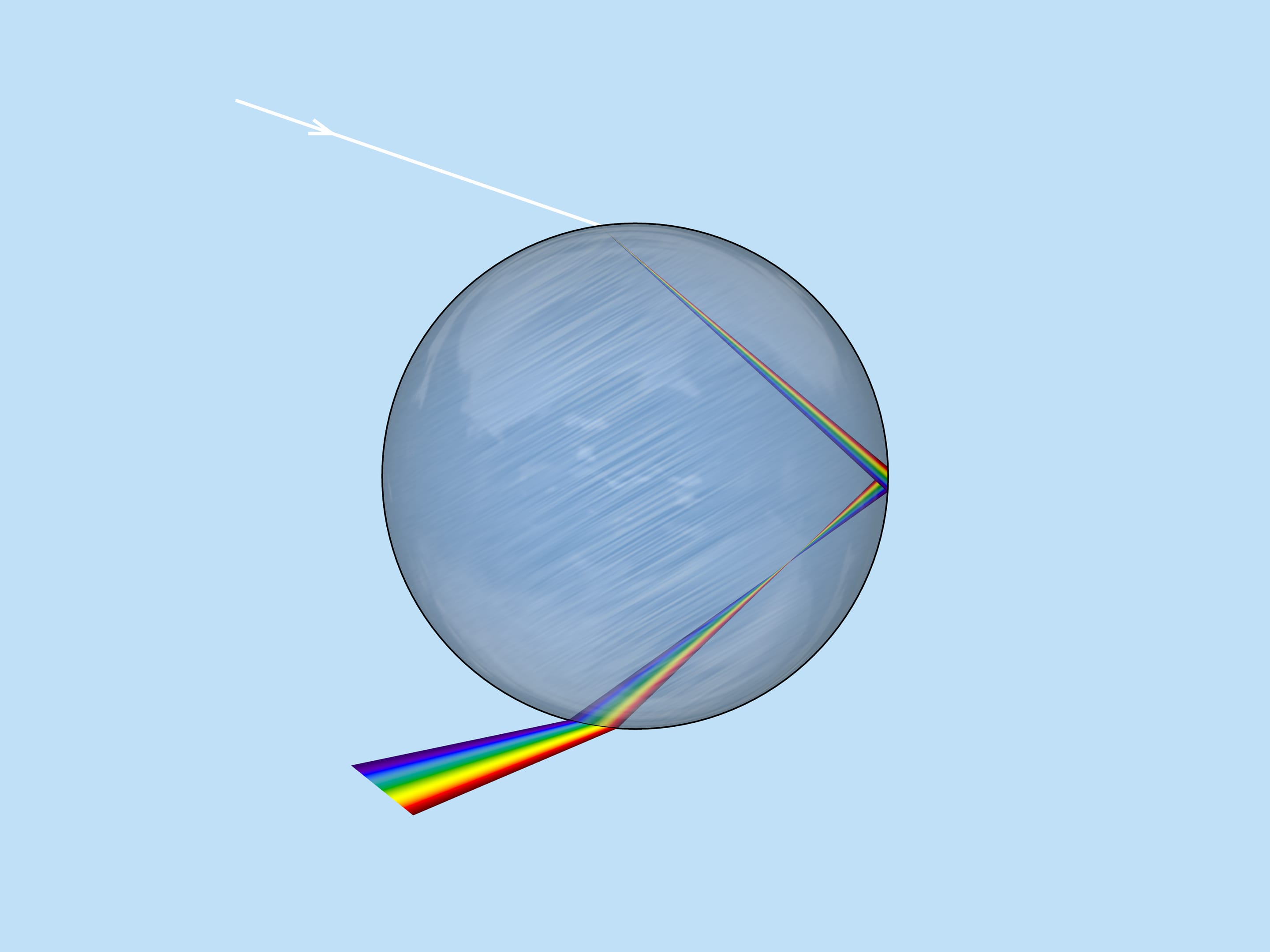
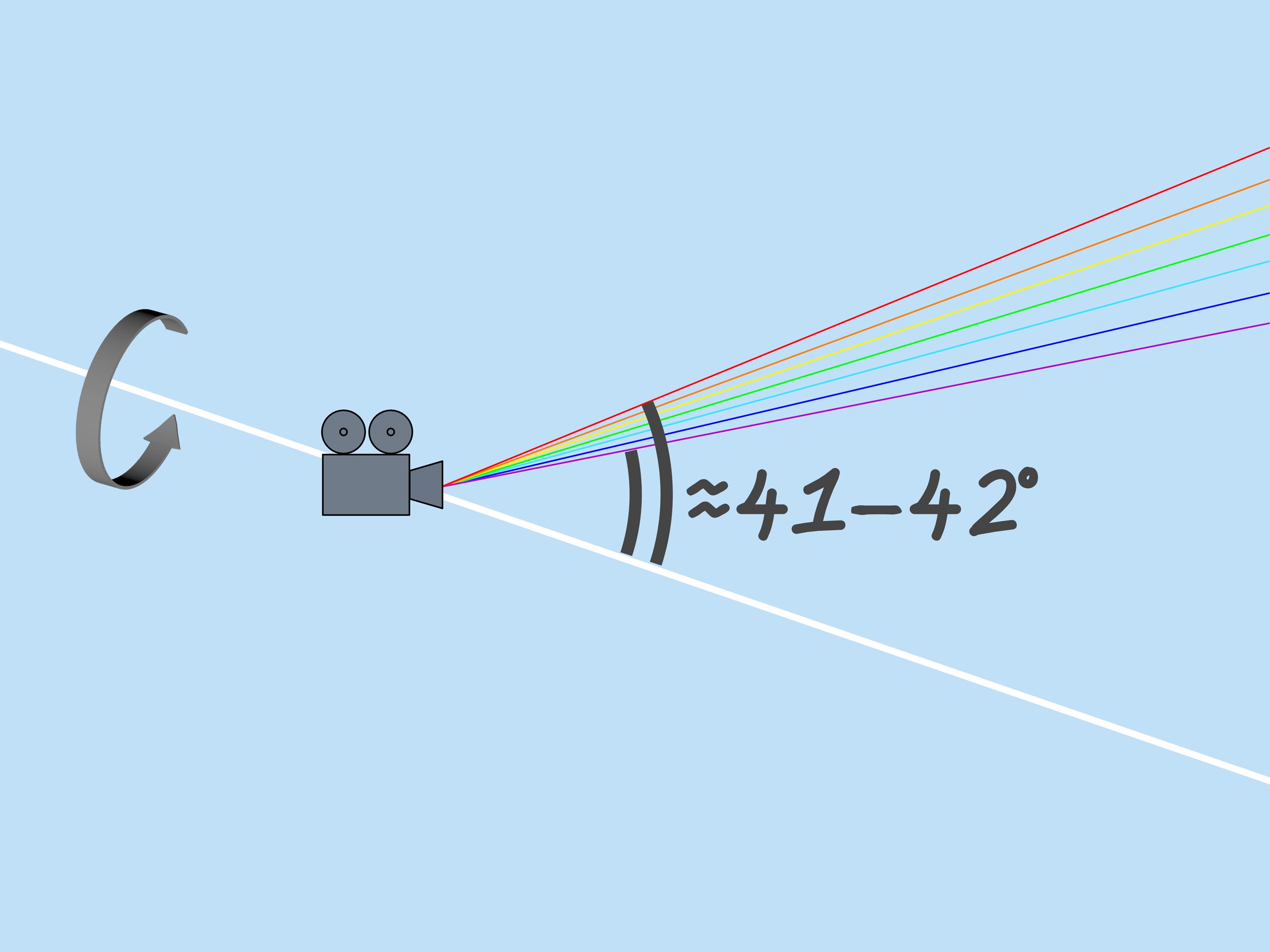
As mentioned above, the refrac­tive index for the rays of different colors is different, so the maximum deflec­tion angle is also different. For red, it is about $42^{\circ}$. And for violet, it’s $41^{\circ}$. The rays of the other colors lie between $41^{\circ}$ and $42^{\circ}$.

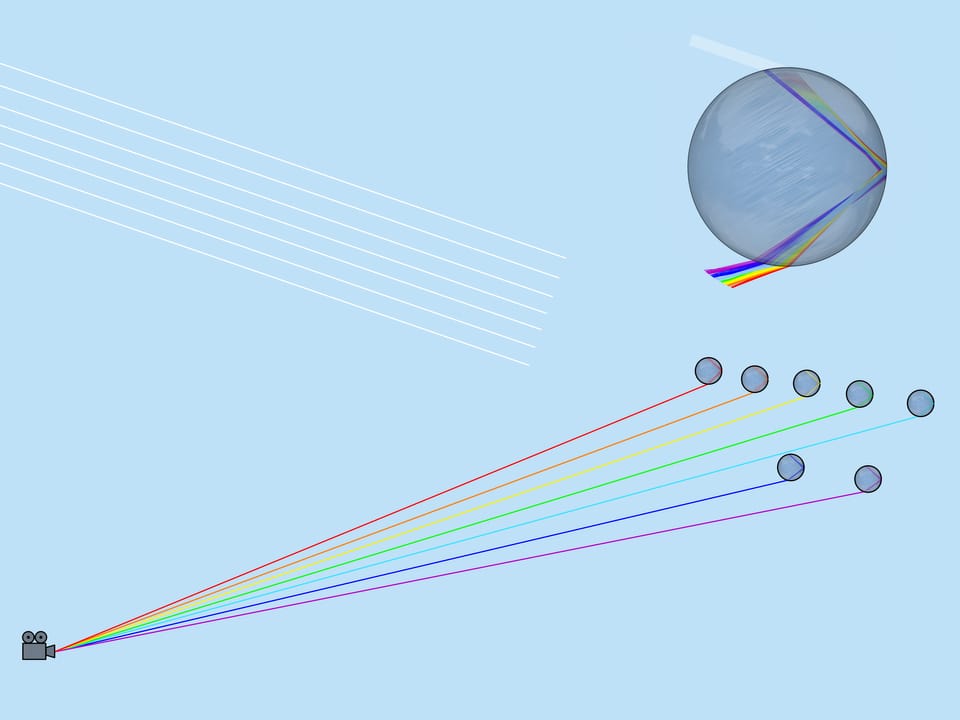
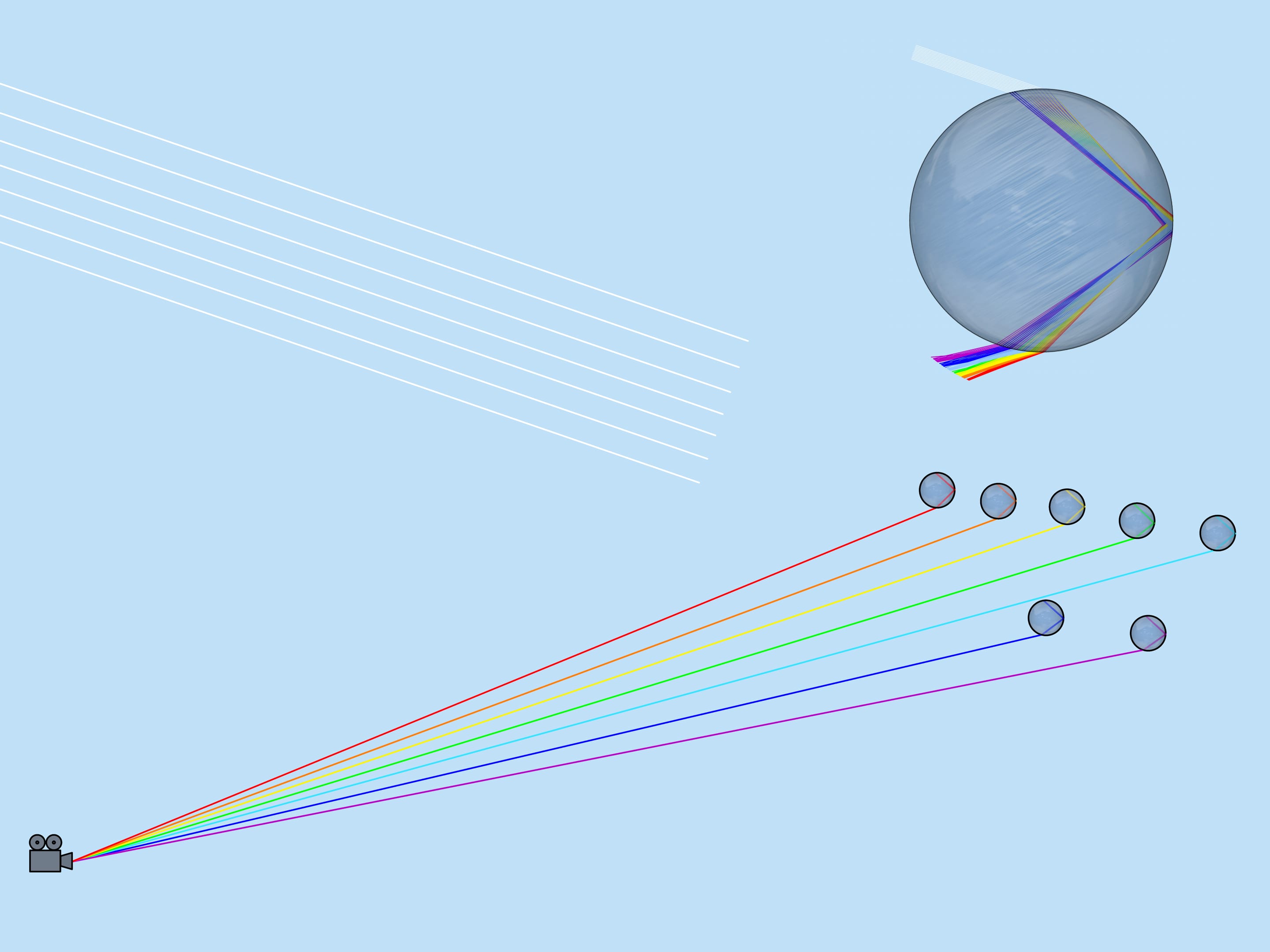
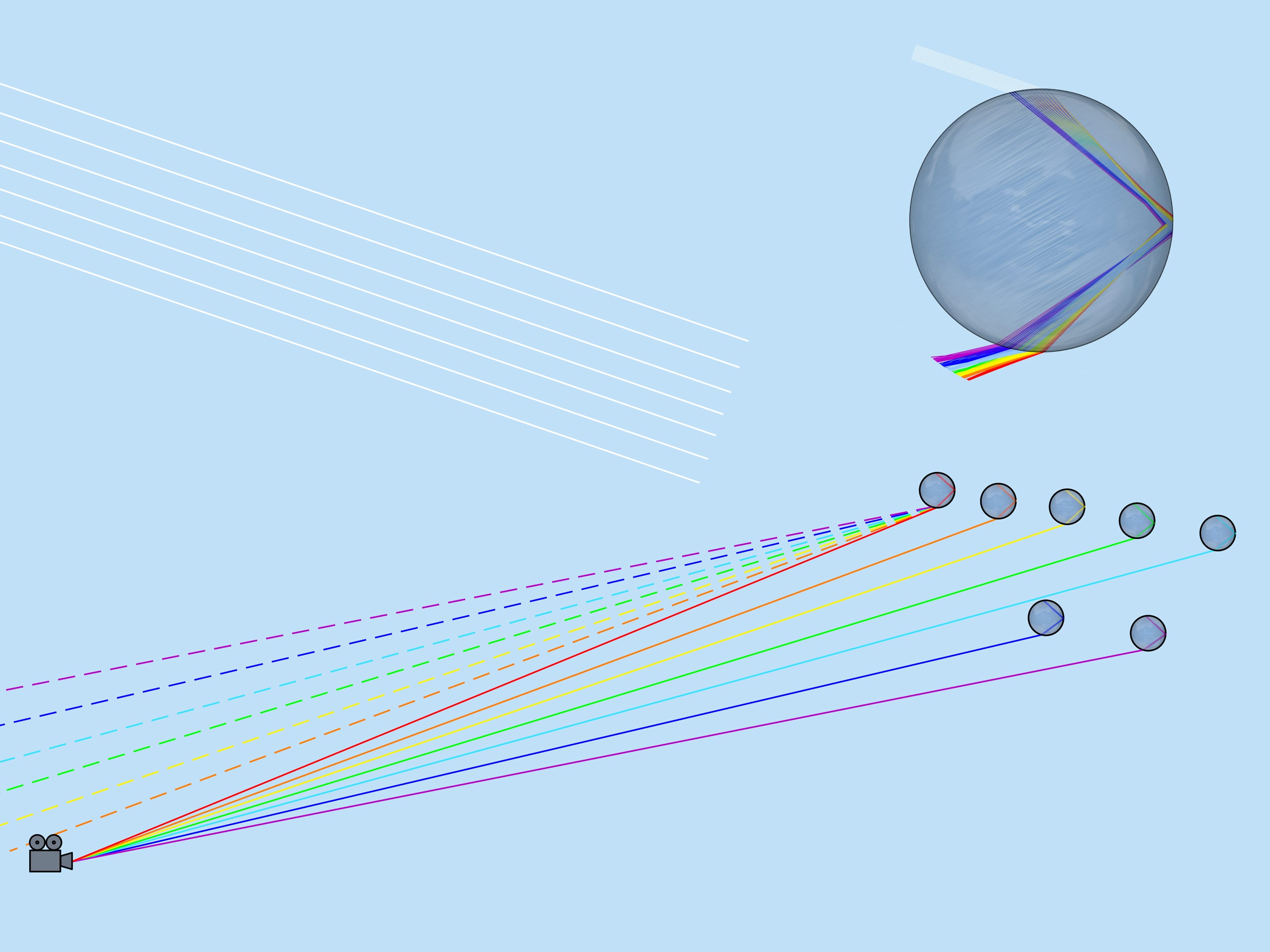
Looking at the picture of light rays passing through a droplet, we will notice that if for example yellow color came to the observer from the given droplet, no other color can come from this droplet (red will go lower, and blue will go higher than the observer). Thus only one color is visible from each droplet.

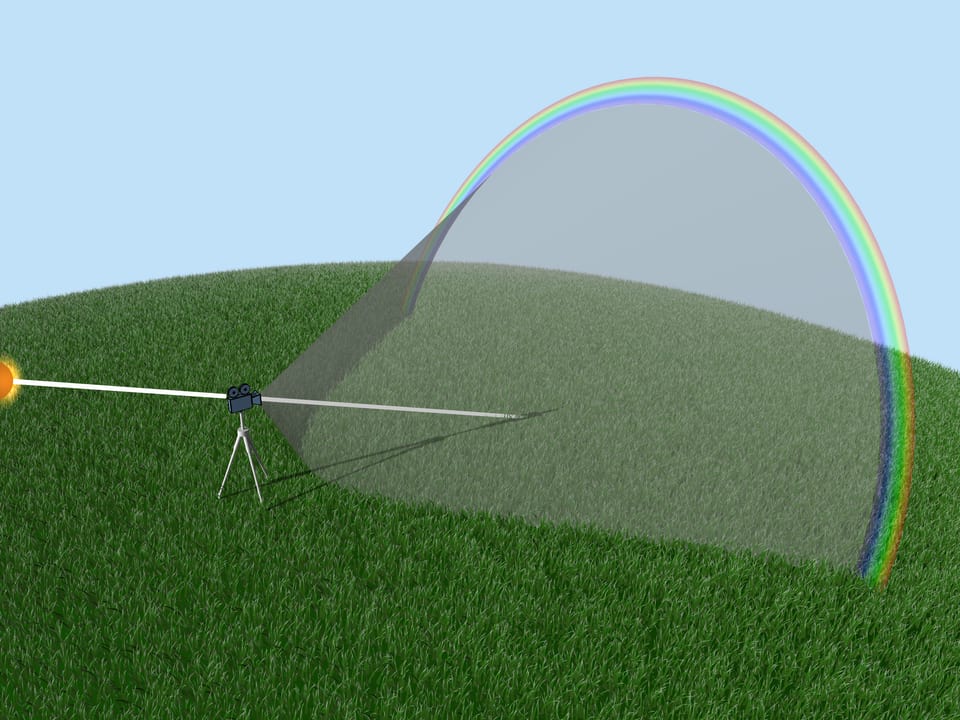
Now consider the whole multi­tude of rain­drops. Which rain­drops are respon­sible for the forma­tion of the rainbow color? From the above it is clear that, for example, the violet color is formed by those and only those droplets that lie on the straight line forming an angle $42^{\circ}$ with the coming to the Earth solar rays. So, the violet color of the rainbow lies on the surface of the cone with the vertex in the observer, the axis being a contin­u­a­tion of the segment “the Sun — observer’s eye”, and the aper­ture $42^{\circ}$. Other colors also lay on surfaces of cones with the same axis and corre­sponding to these colors aper­ture.

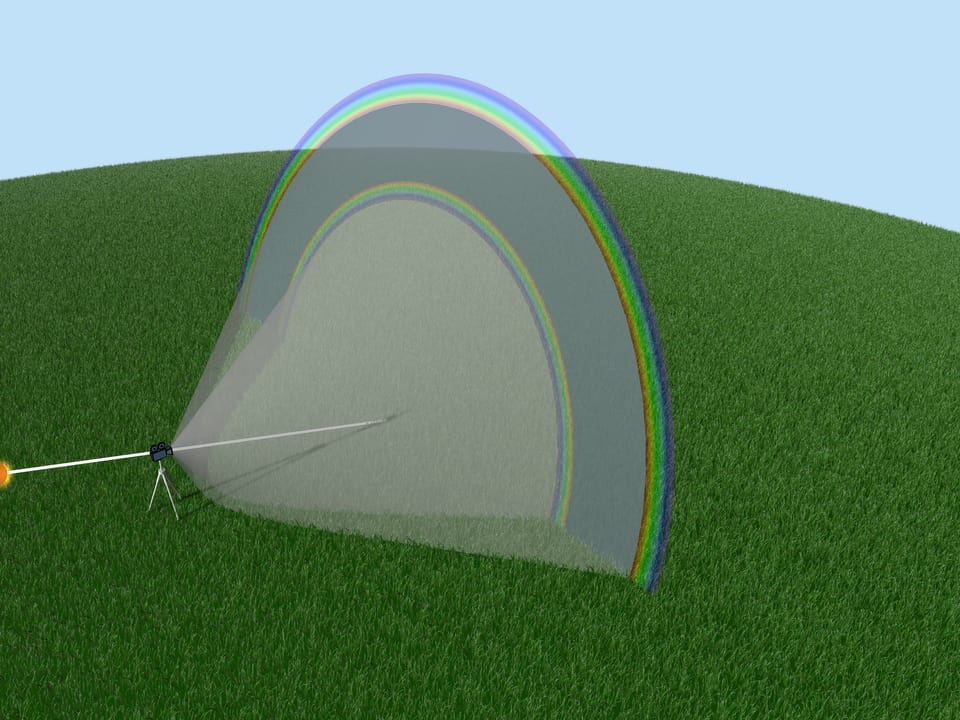
If an observer looks at the rainbow, then the Sun is behind him. The rainbow is said to be at the “anti-solar point.” The height of the rainbow depends on the posi­tion of the Sun. The largest rainbow is seen when the Sun is close to the horizon.

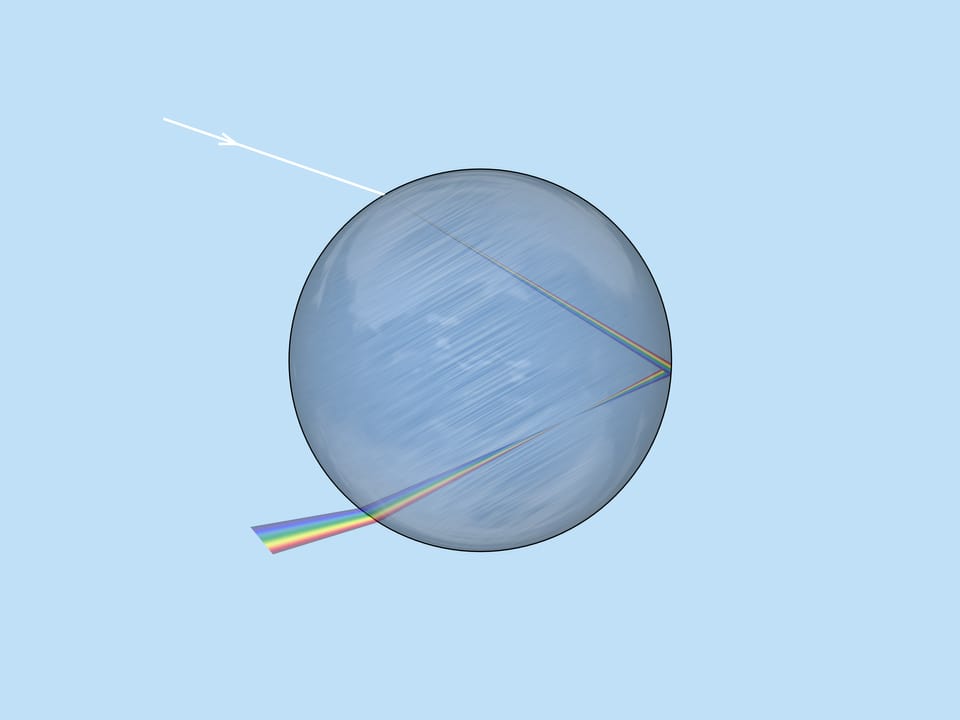
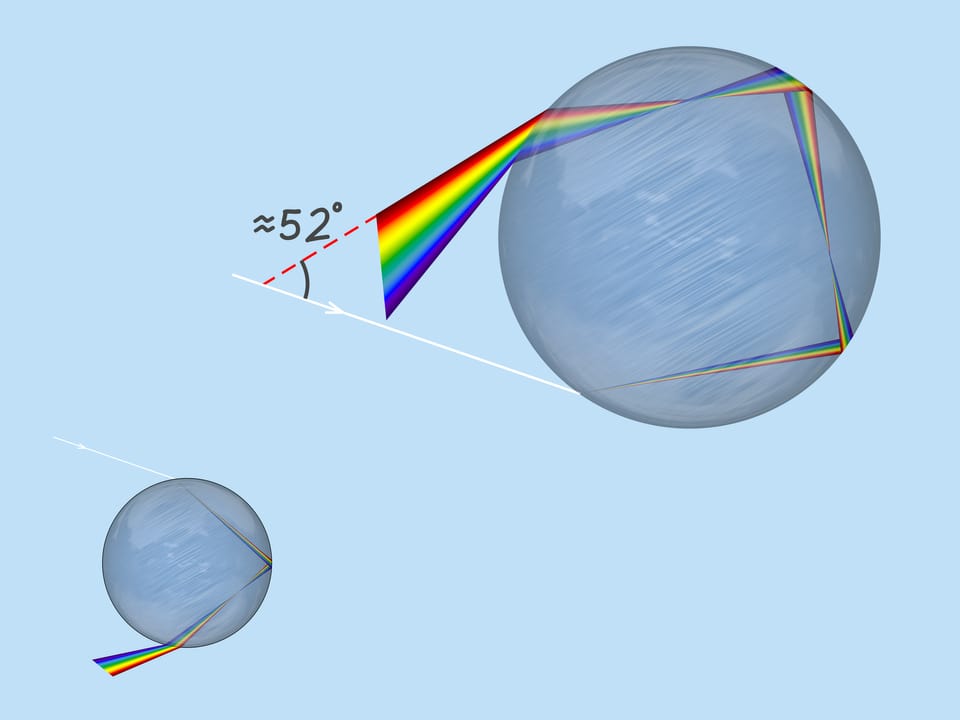
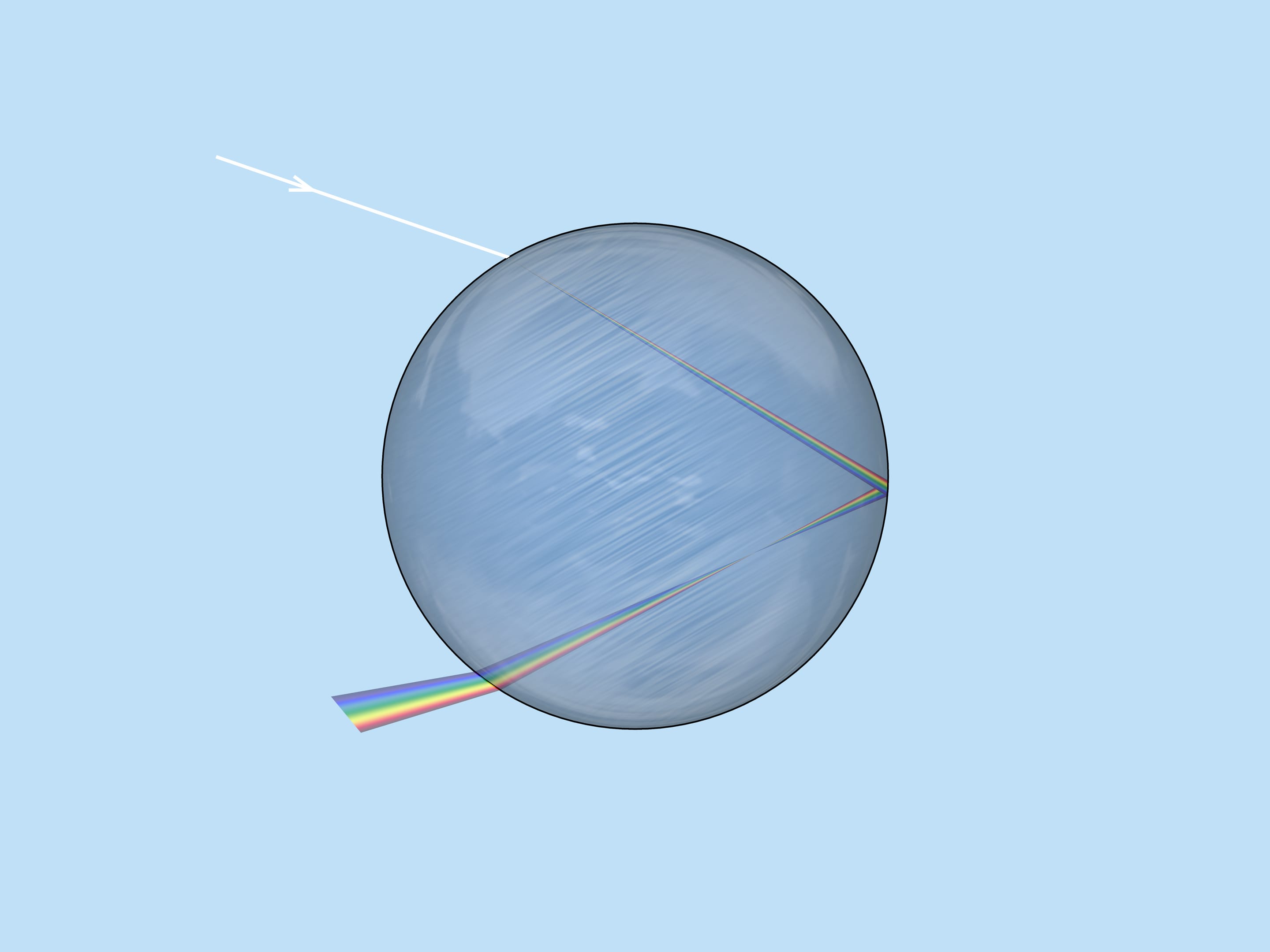
Now consider the solar rays hitting the lower part of the droplet. Due to symmetry, the above reasoning can be almost completely repli­cated. But then the solar rays go upwards on leaving the droplet, and the observer on the Earth cannot see them. However, another path of a solar rays in the droplet is also possible! The rays may reflect twice from the back side of the droplet and then leave it.

This path of the solar rays produces a second rainbow. The second rainbow can be seen at an angle of about $52^{\circ}$ to the “the Sun — observer’s eye” direc­tion. Thus, it is higher than the first rainbow. Since the rays were reflected from the sides of the droplet twice, the order of colors is reversed: red at the bottom and violet at the top.

With each reflec­tion, the inten­sity of light decreases, so the second rainbow is less vivid than the first. Theo­ret­i­cally, the third rainbow and higher order rain­bows also exist, but they are not visible under normal condi­tions because they are produced by many reflec­tions in the droplet.

An atten­tive person will notice the dark area of the sky located between the first and second rain­bows. The fact is that after inter­acting with the droplets, only small numbers of rays come to the observer at angles from $41^{\circ}$ to $52^{\circ}$. Another sign of the rainbow that is not always noticed is the light and dark stripes just below the violet arc of the first rainbow. However, their expla­na­tion goes beyond geometric optics.

It is impos­sible for an observer standing on the Earth to see a full circle of the rainbow in the sky. The full rainbow, the whole circle, can be seen in a foun­tain splashes not too high above the ground. In the sky, a full rainbow can be seen from an airplane.