Even a pile of identical bases can brighten up learning mathematics and physics.
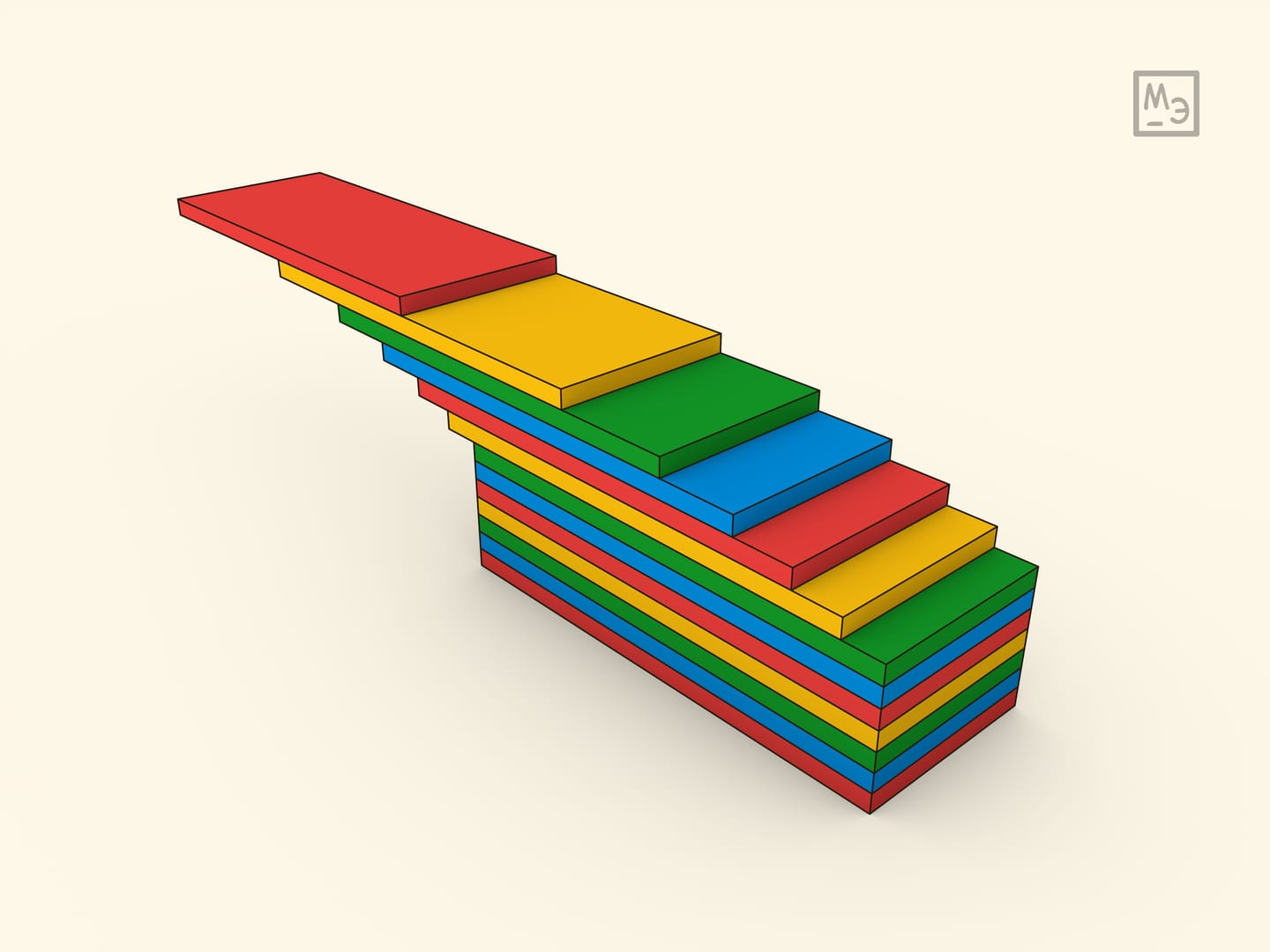
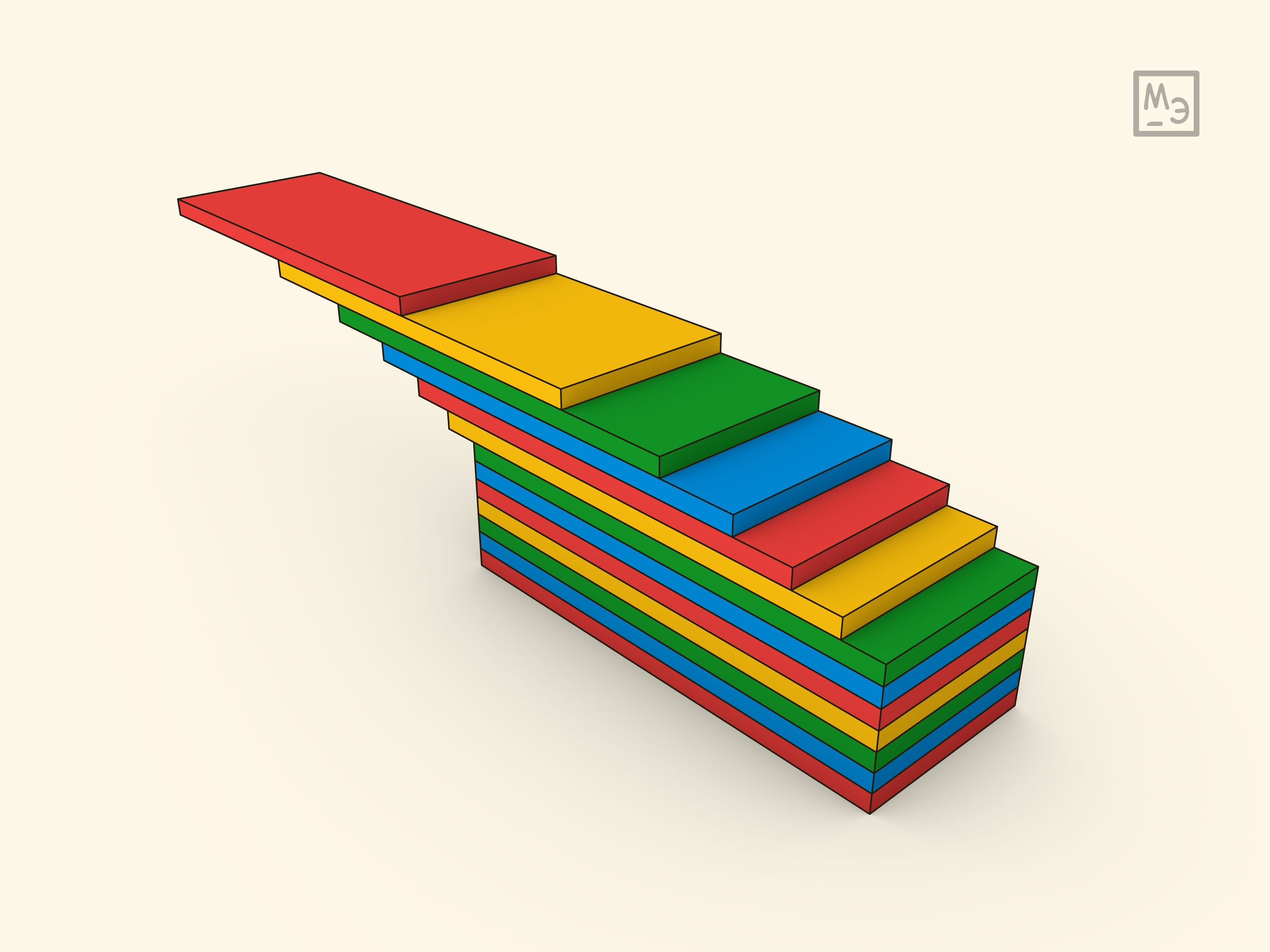
To what extent can one move an upper base, so that it won’t fall? We know the answer from our childhood: to the half of length — so that gravity centre doesn’t go out of the other layers’ basement.
And now — to what extent can one move two upper layers? In this case an experiment should be proved by calculation. It’s interesting to count, where a gravity centre of maximally moved several bases is located.
And how far can such “ladder” go horizontally? Mathematically, it can go infinitely, as maximally possible moves of the bases correspond to a divergent harmonic row. In the movie Stairway to infinity a mechanical loader and a tractor move bricks in an optimal way and explain the proof of a harmonic row’s divergence. And one can make an experiment with one’s friends, how far can one build such a ladder.
Museums
Other models in “Number theory”
