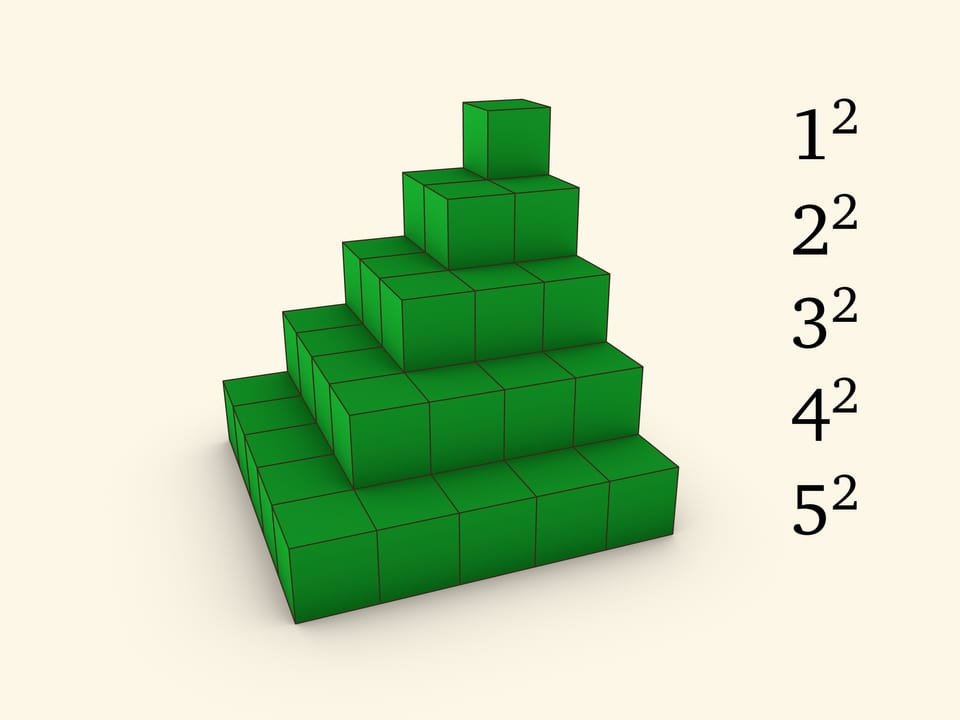
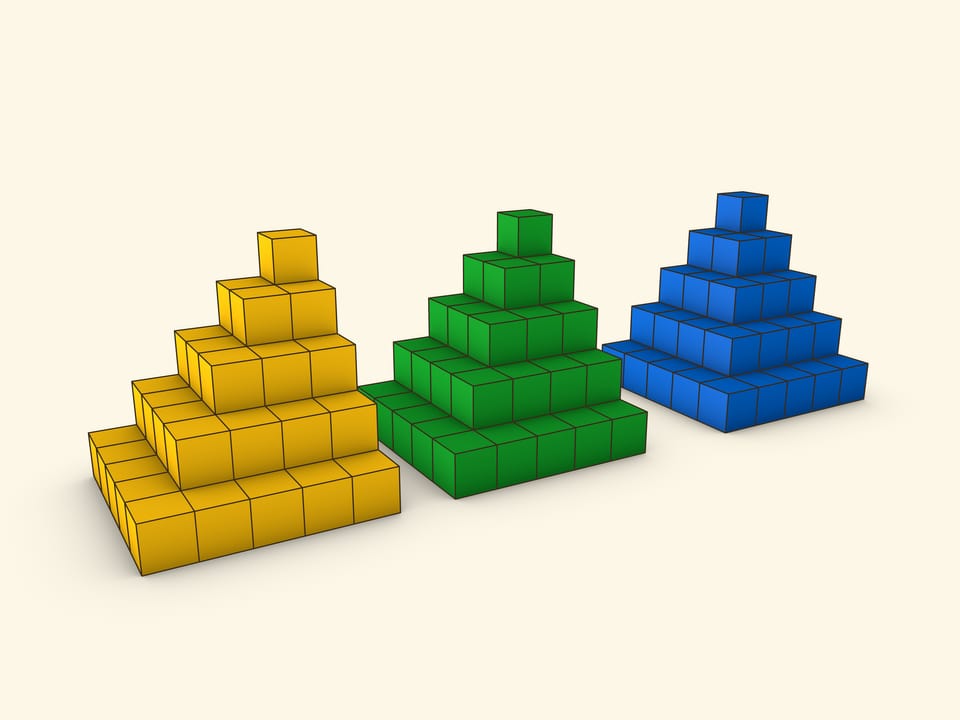
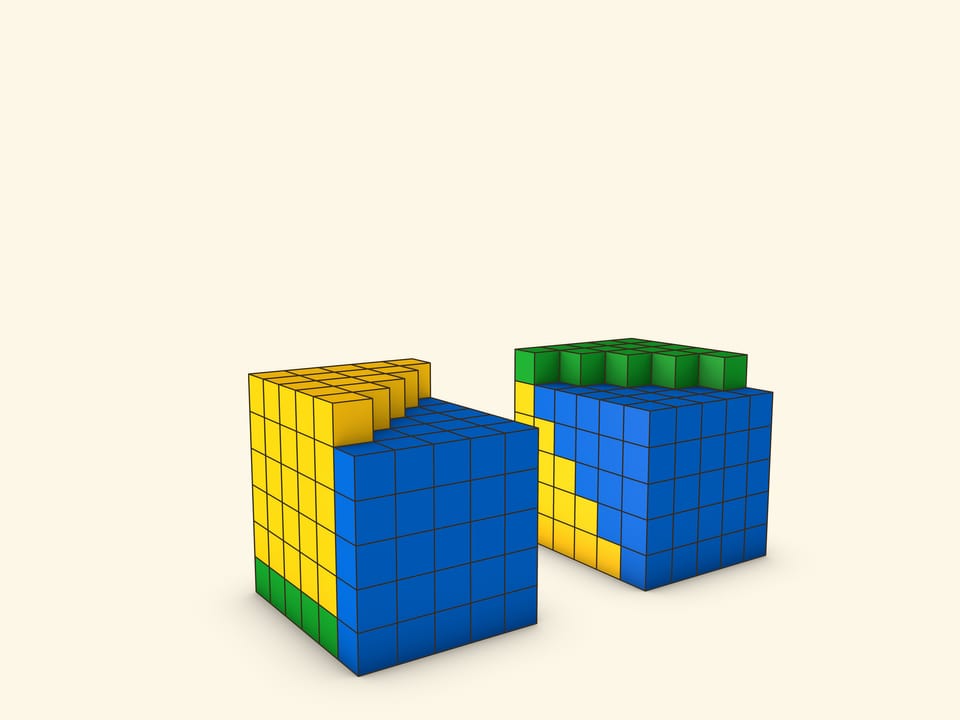
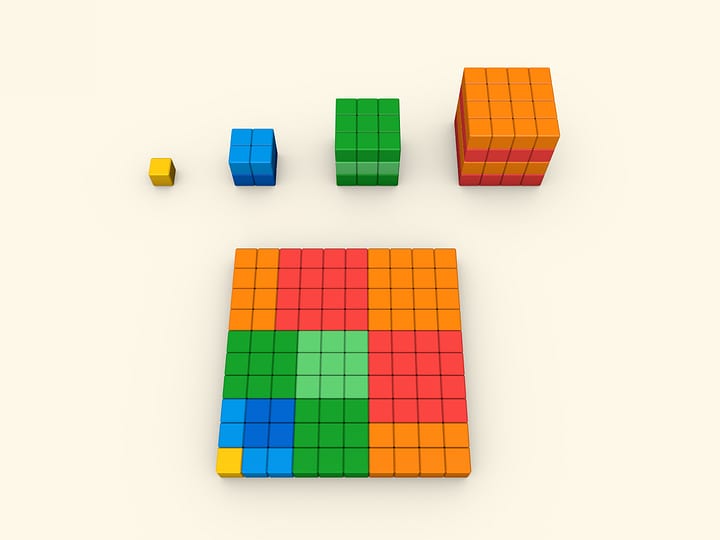
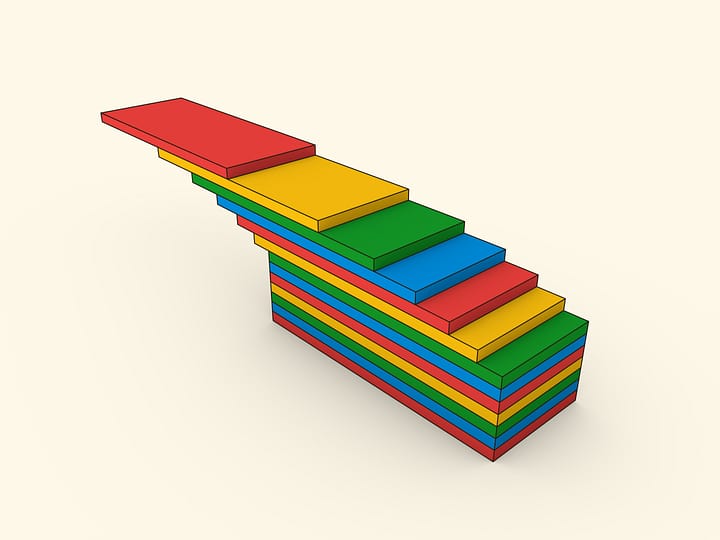
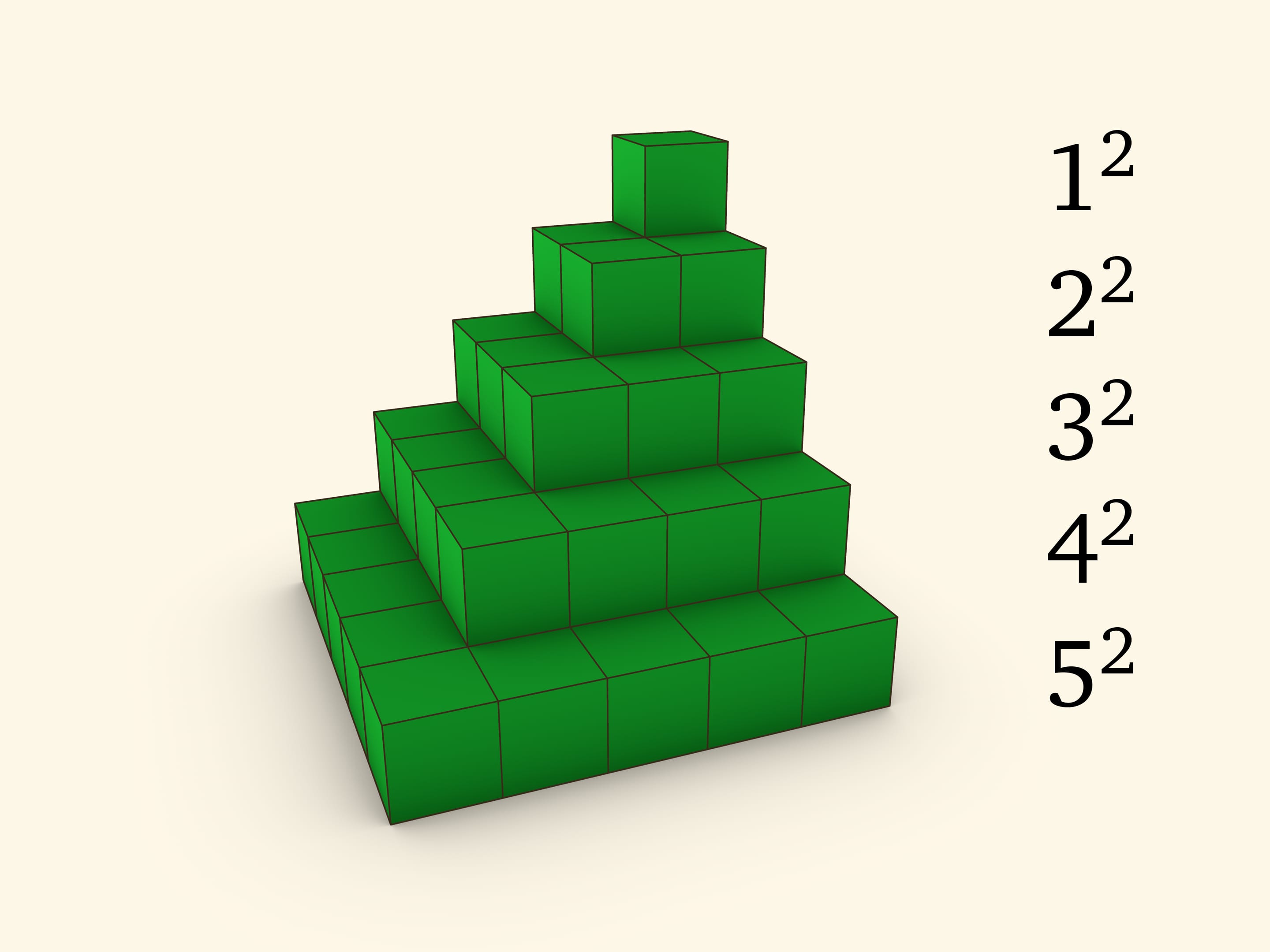
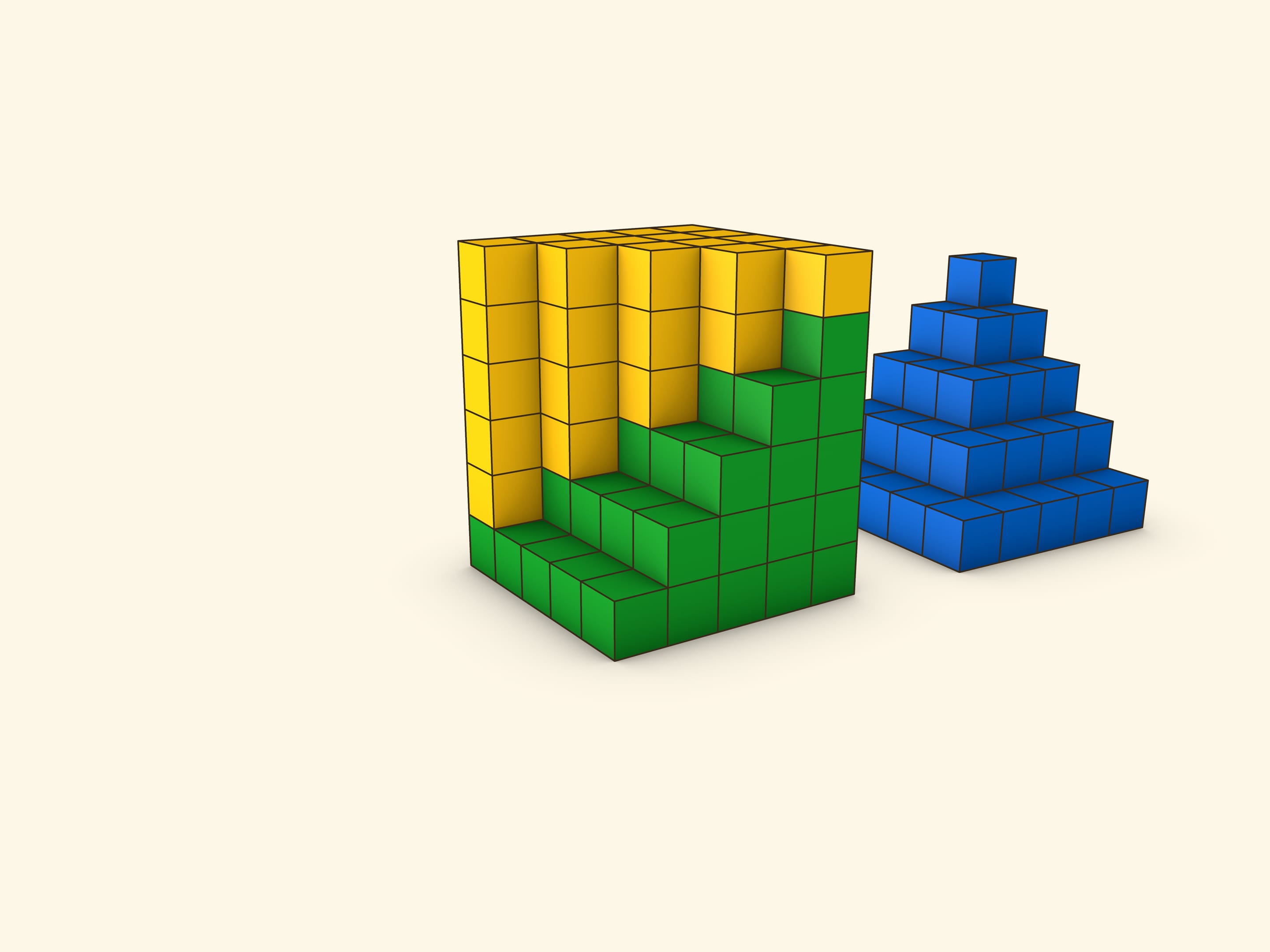
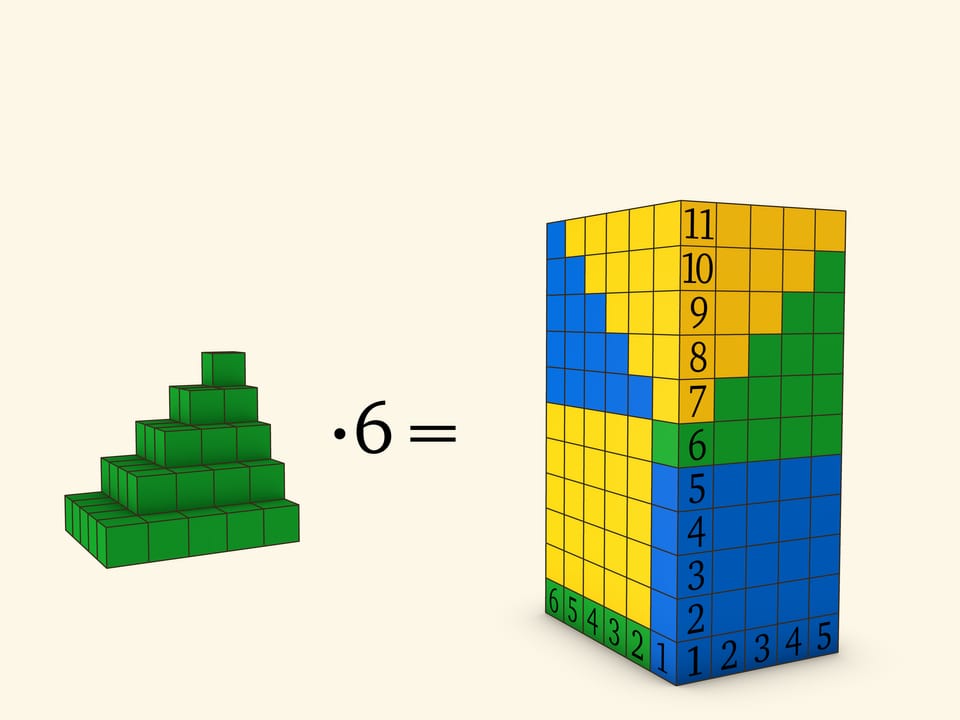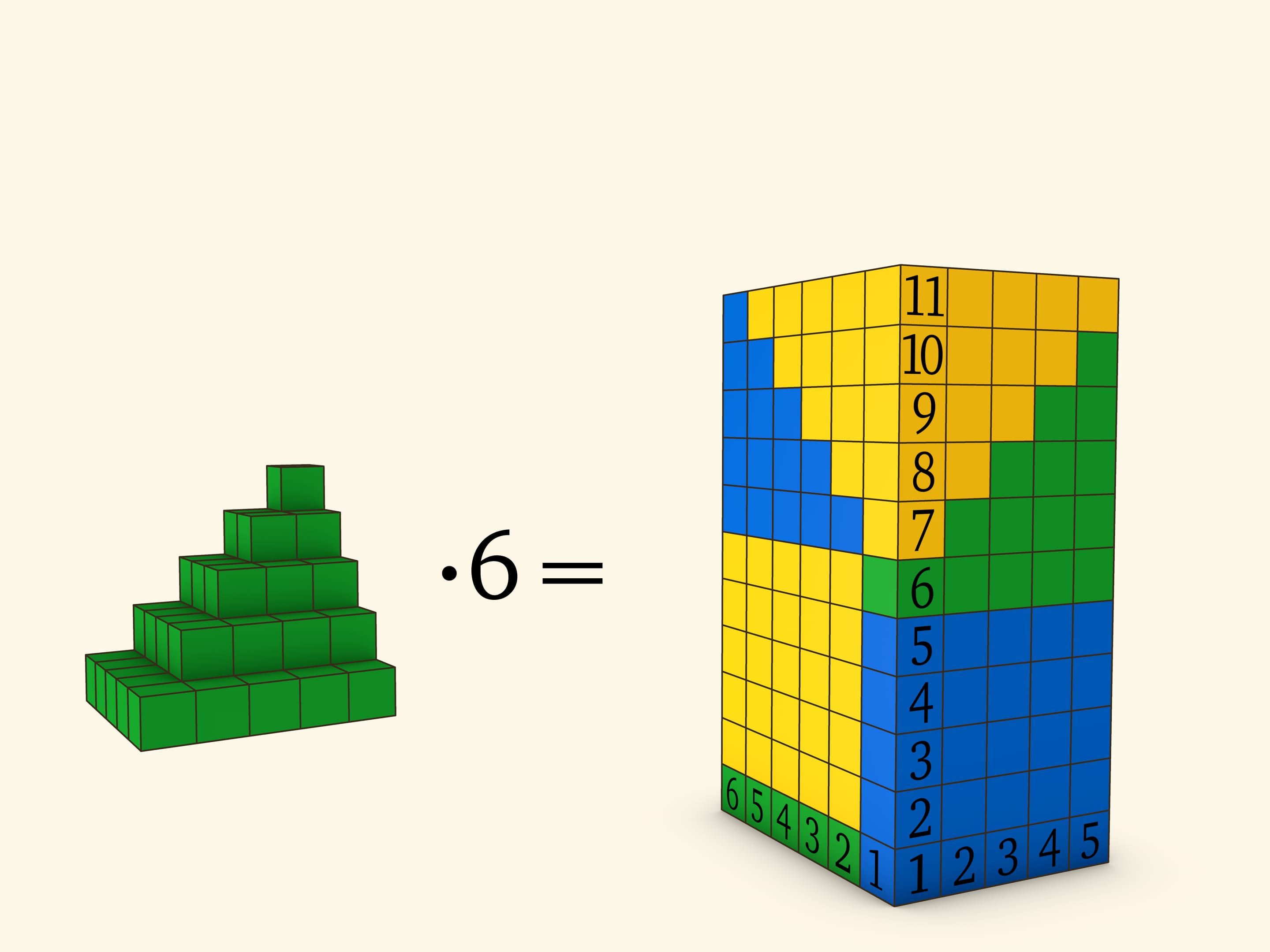Let’s take a square number (a square of some integer) of cubes. They can be arranged in a square. Let’s make five such squares for first five natural numbers. Put them one upon the other and glue them together. We get a stairs-like piece.
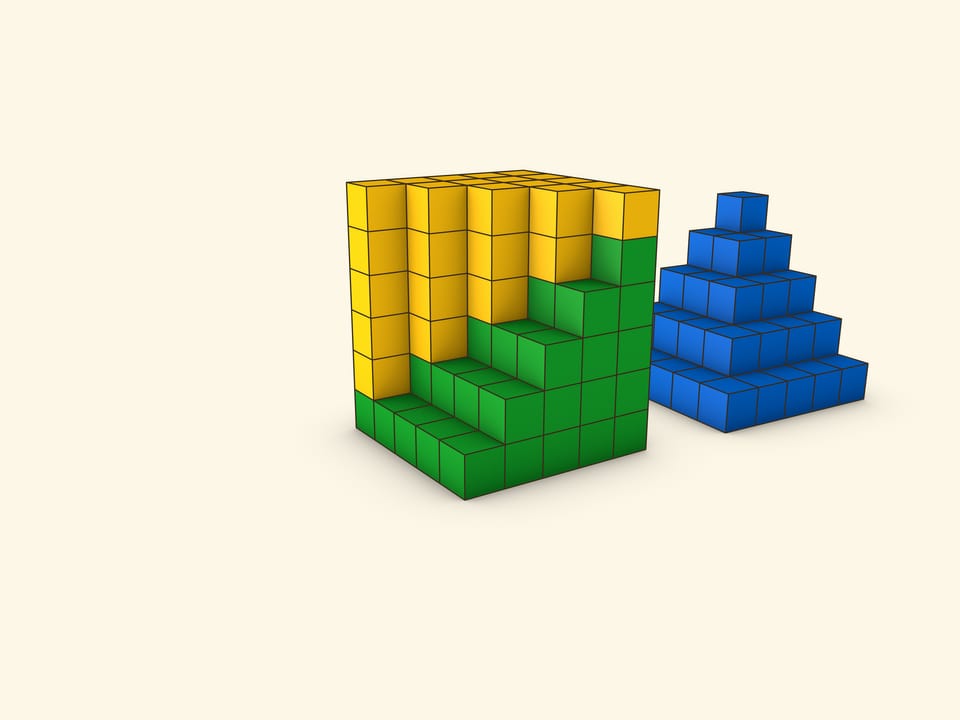
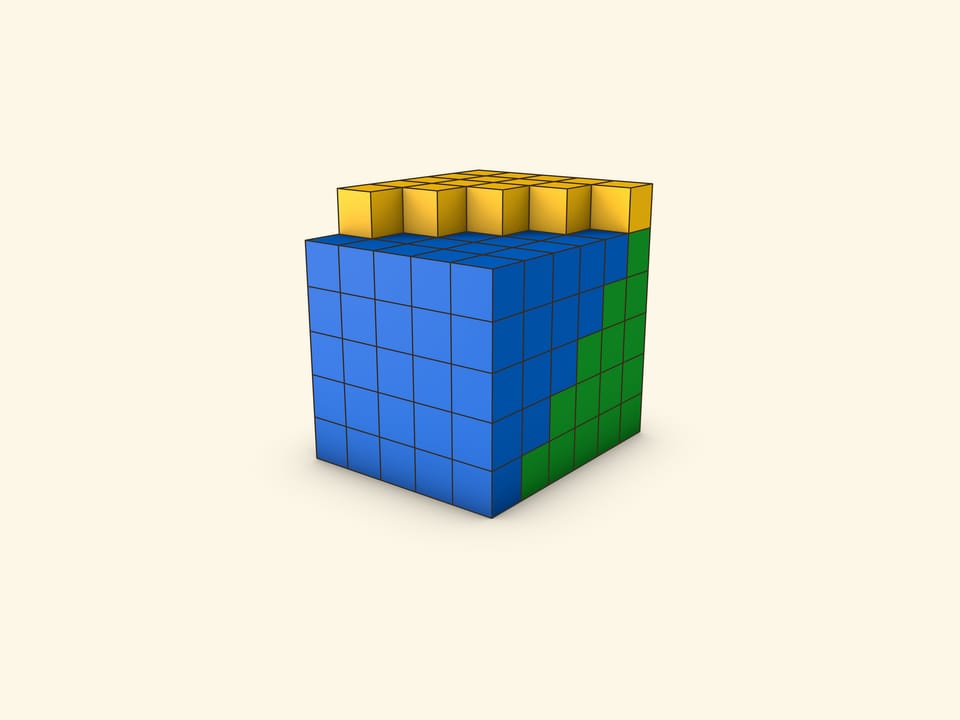
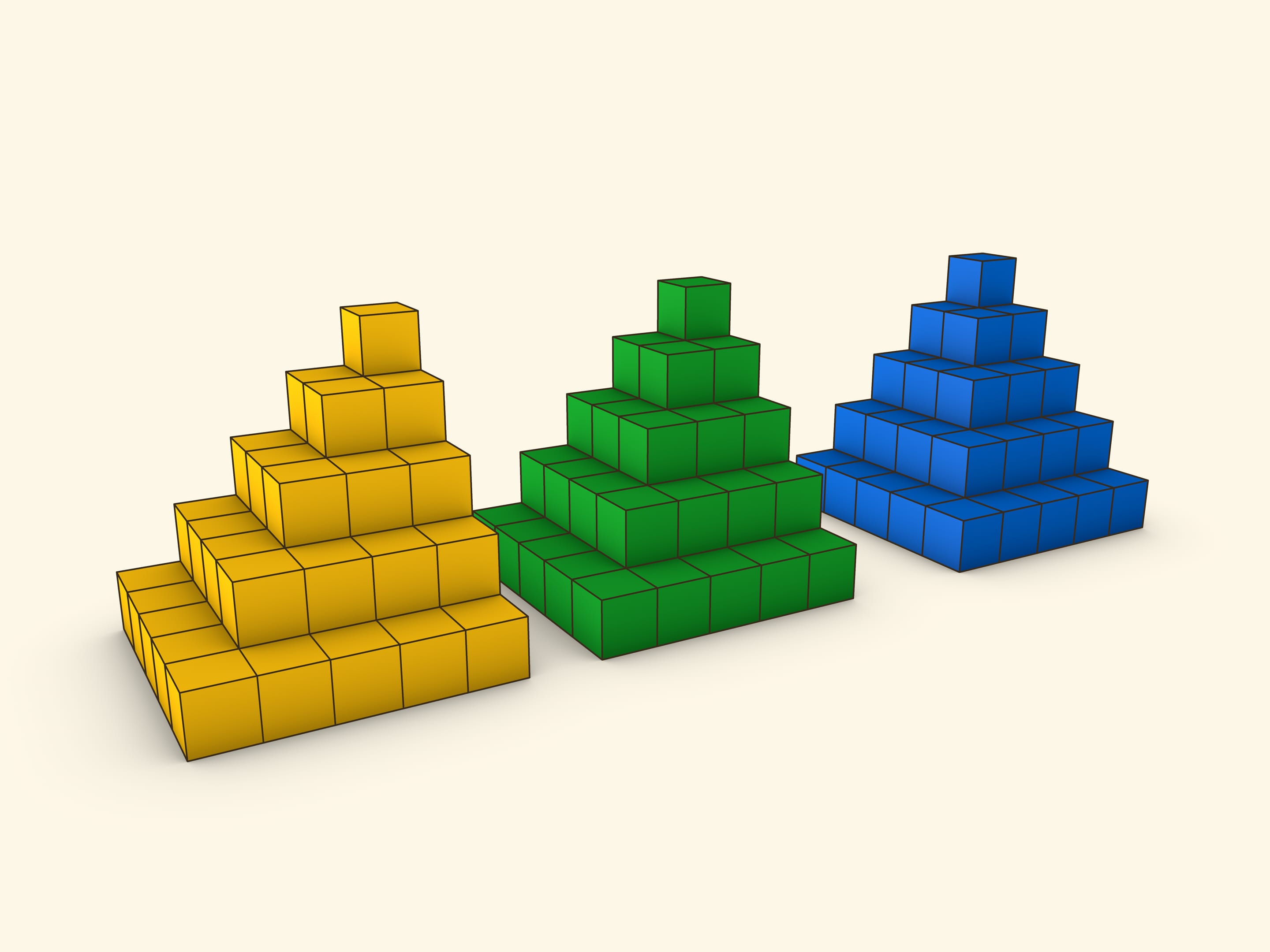
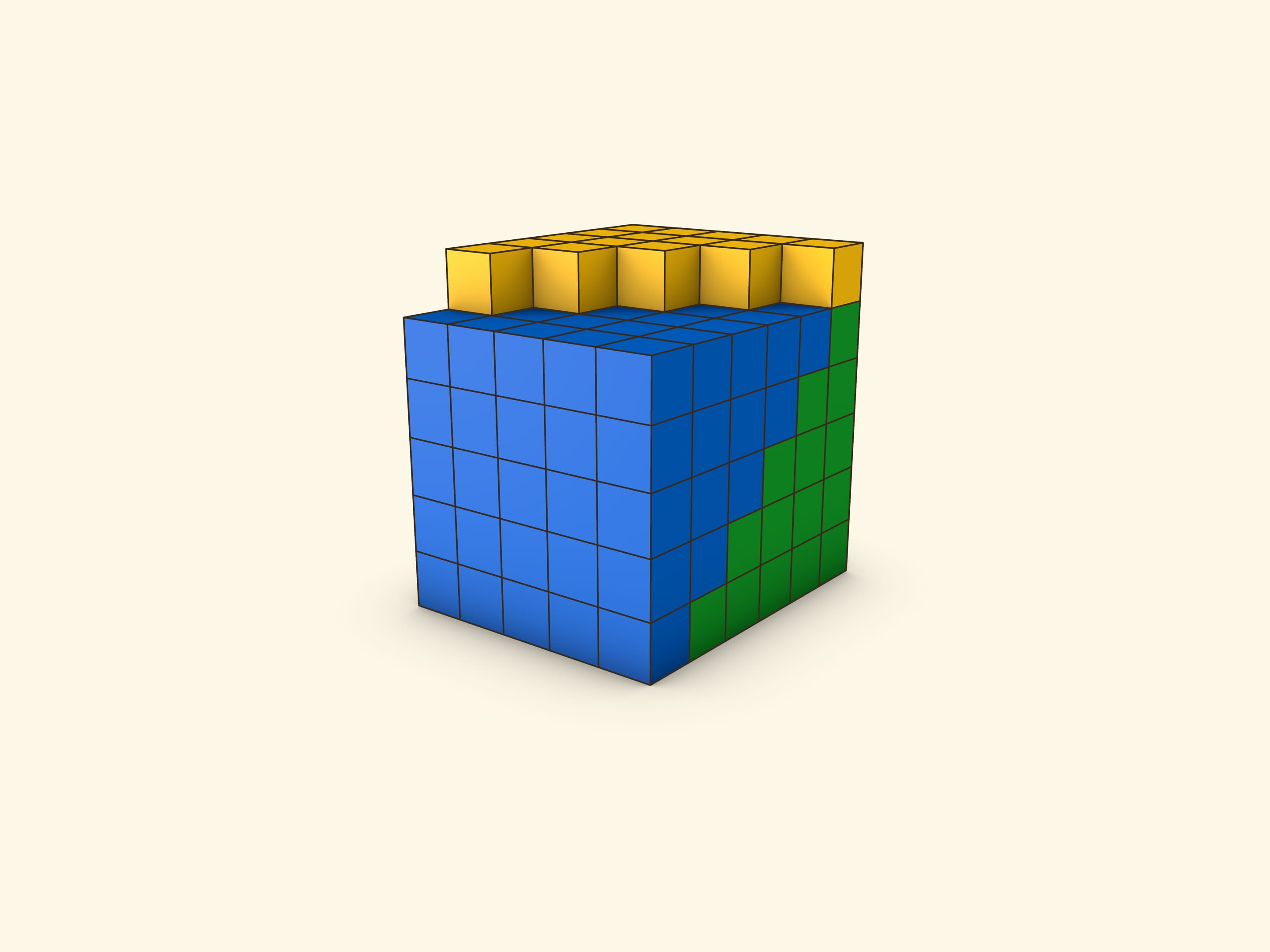
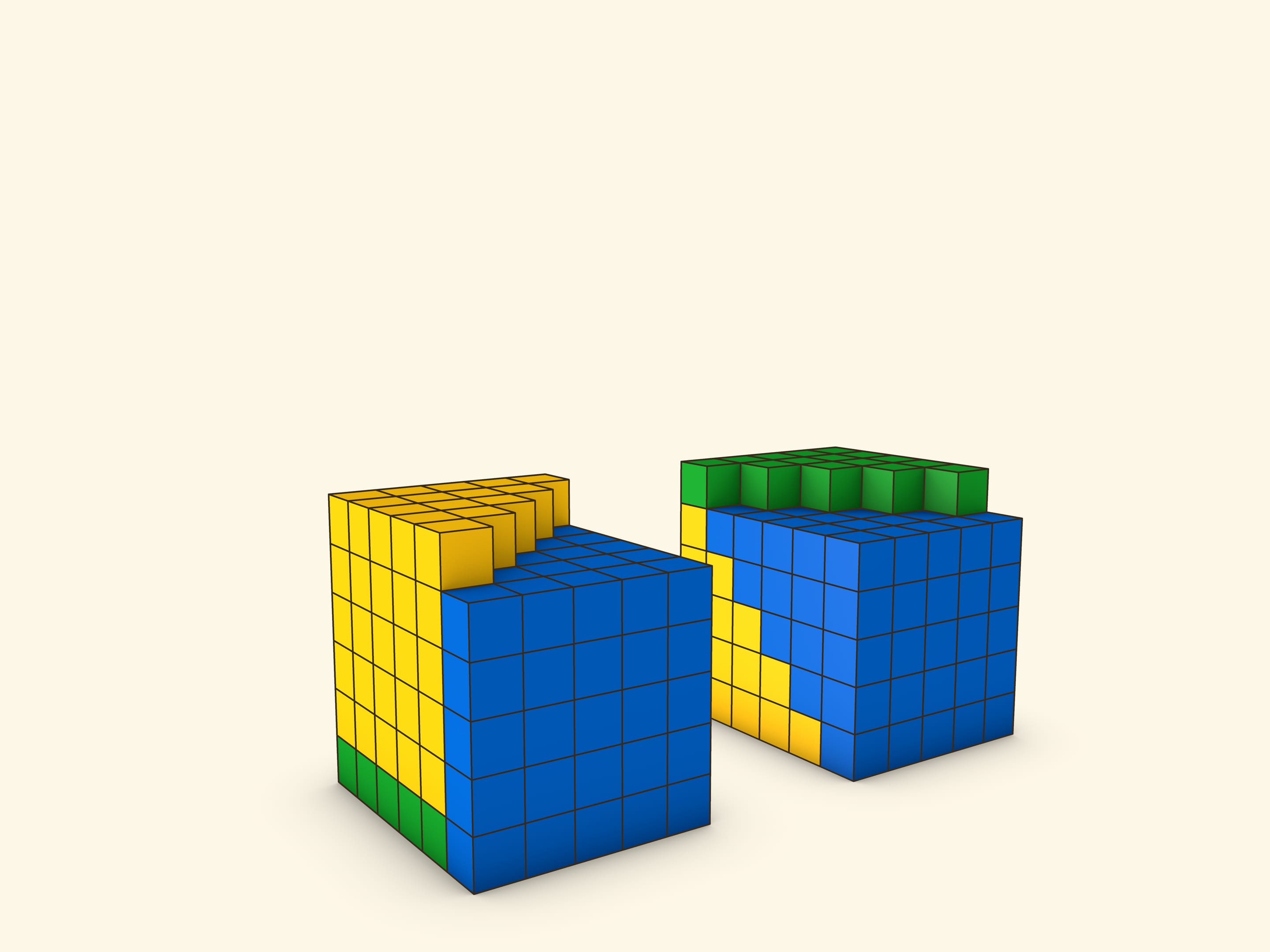
Three such pieces can be put together to form a figure looking like a parallelepiped with a ledge. Other three pieces form an alike shape. Putting the two together, we get a solid parallelepiped.
The volume of this parallelepiped, measured in number of cubes, equals, on the one hand, the product of each side’s lengths measured in cubes, and on the other hand, six times the sum of first five squares. From here one can guess the general formula for the sum of first n squares.
For those who liked the “wooden” model, we also keep the first version of the video.